Choose the correct option
Two seconds after the projection, a projectile is moving at 30∘ above the horizontal, after one more second it moves horizontally (g=10m/s2).
the magnitude of the initial velocity is 20√3m/s
the angle of projection is 60∘
the maximum height attained by the projectile is 45m
the horizontal range is 60m
The correct Answer is:D
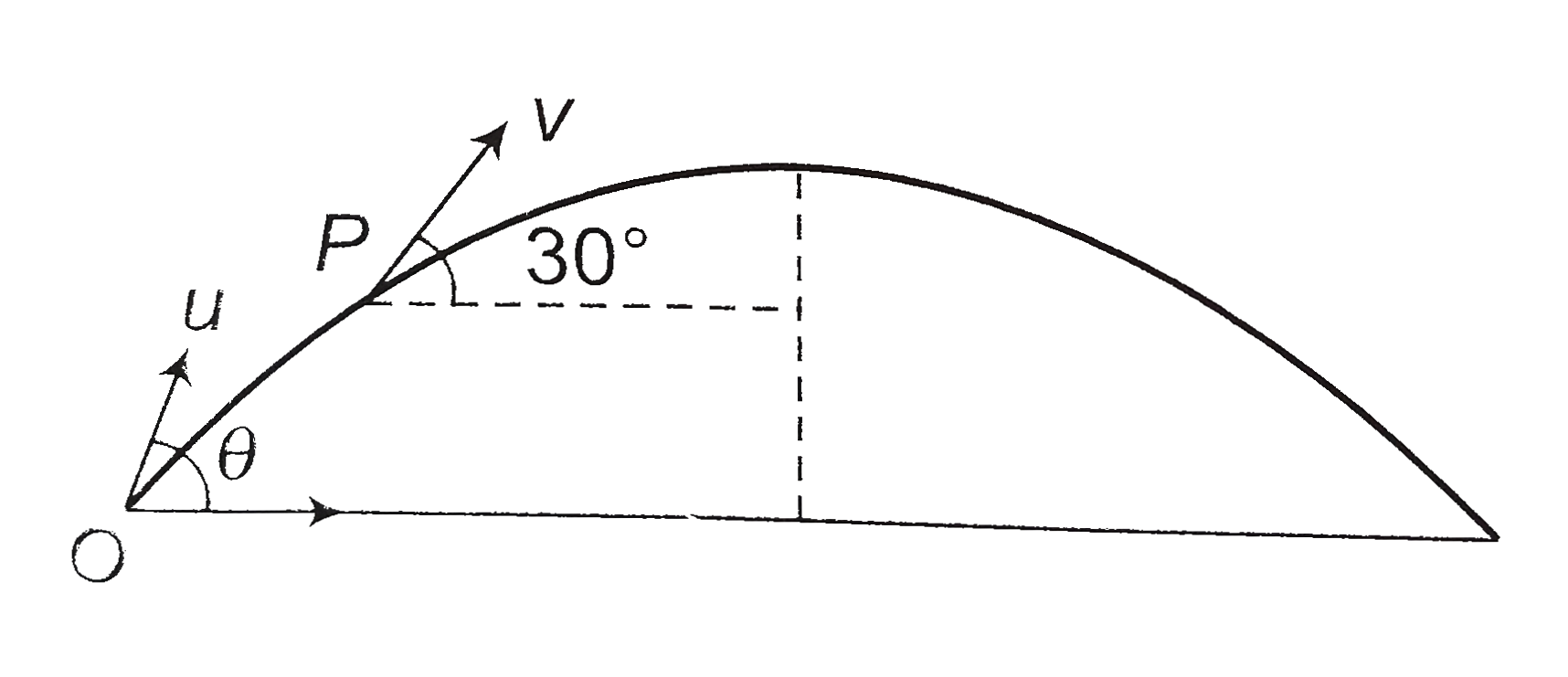
After 3s, the velocity becomes horizontal, i.e., particle reaches at the highest point
usinθg=3⇒usinθ=30 (i)
After 2s, the velocity makes an angle 30∘ with horizontal
tanα=vyvx=usinθ−gtucosθ
tan30∘=30−10×2ucosθ⇒1√3=10ucosθ
ucosθ=10√3 (ii)
u=√(usinθ)2+(ucosθ)2=20√3m/s
tanθ=usinθucosθ=√3⇒θ=60∘
Hmax=(usinθ)22g=45m
R=u2gsin2θ=120010sin120∘=60√3m
Topper's Solved these Questions
Similar Questions
Two seconds after projection, a projectile is travelling in a direction inclined at 30∘ to the horizontal. After one more second, it is travelling horizontally. Find the magnitude and direction of its velocity.
View SolutionTwo second after projection, a projectile is travelling in a direction inclined at 30∘ to the horizontal. After one more second, it is travelling horizontally. Find the magnitude and direction of the velocity of projection.
View Solution
Knowledge Check
- Question 1 - Select One
Two second after projection, a projectile is moving at 30∘ above the hoorizontal, after one more second it is moving horizontally. The intialy speed of the projectile is (g=10m/s2)
A10 m/sB34 m/sC30 m/sD40 m/s - Question 2 - Select One
Two second after projection, a projectile is travelling in a direction inclined at 30∘ to the horizontal. After one more second, it is travelling horizontally. Find the magnitude and direction of the velocity of projection.
Athe velocity of projection is 20 m/sBthe velocity of projection is 20√3m/sCthe angle of projection is 30∘ with vertical.Dthe angle of projection is 30∘ with horizontal - Question 3 - Select One
After one second the velocity of a projectile makes an angle of 45∘ with the horizontal. After another one more second its is travelling horizontally. The magnitude of its initial velocity and angle of projectile are
(g=10m/s2)A14.62m/s,60∘B14.62m/s,tan−1(2)C22.36m/s,tan−1(2)D22.36m/s,60∘
Two seconds after projection, a projectile is travelling in a direction inclined at 30∘ to the horizontal. After one more second, it is travelling horizontally. Find the magnitude and direction of its velocity.
View SolutionTwo seconds after projection a projectile is travelling in a direction inclined at 30∘ to the horizon, after one more second it is travelling horizontally. The magnitude and direction of its velocity are 64√3 and 60∘ respectively.
View SolutionWhat are the angles of projection of a projectile projected with velocity 30ms−1, so that the horizontal range is 45 m ? Take g=10ms−2.
View SolutionA particle is projected from the ground . One second after the projection, the particle is found to be moving at an angle 45∘ with the horizontal . Speed of the particle becomes minimum two seconds after the projection . If angle of projection is tan−1(n), then what is the value of n ?
View SolutionA projectile is thrown with velocity u making an angle θ with the horizontal. Its time of flight on the horizontal ground is 4 second. The projectile is moving at angle of 45∘ with the horizontal just one second after the projection. Hence the angle θ is (take g=10m/s2)
View Solution

