A ball is projected at an angle 45∘ with horizontal. It passes through a wall of height h at horizontal distance d1 from the point of projection and strikes the ground at a horizontal distance (d1+d2) from the point of projection, then
h=2dd_(2)d+d2
h=d1d2d1+d2
h=√2d1d2d1+d2
h=d1d22(d1+d2)
The correct Answer is:B
To solve the problem step by step, we need to analyze the projectile motion of the ball that is projected at an angle of 45∘ and passes through a wall of height h at a horizontal distance d1 before hitting the ground at a distance d1+d2.
Step 1: Understand the motion of the projectile
The ball is projected at an angle of 45∘ with the horizontal. The horizontal and vertical components of the initial velocity u can be expressed as:
- ux=ucos(45∘)=u√2
- uy=usin(45∘)=u√2
Step 2: Determine the time to reach the wall
The horizontal distance to the wall is d1. The time t1 taken to reach the wall can be calculated using the horizontal motion equation:
d1=ux⋅t1⟹t1=d1ux=d1√2u
Step 3: Calculate the height of the ball when it reaches the wall
The vertical position y of the ball when it reaches the wall can be calculated using the vertical motion equation:
y=uy⋅t1−12gt21
Substituting uy and t1:
h=u√2⋅d1√2u−12g(d1√2u)2
This simplifies to:
h=d1−gd212u2
Step 4: Determine the total range of the projectile
The total horizontal distance the ball travels before hitting the ground is d1+d2. The range R of a projectile launched at an angle θ is given by:
R=u2sin(2θ)g
For θ=45∘:
R=u2g
Thus, we can set:
d1+d2=u2g
Step 5: Relate u2 to d1 and d2
From the equation d1+d2=u2g, we can express u2:
u2=g(d1+d2)
Step 6: Substitute u2 back into the height equation
Substituting u2 into the height equation:
h=d1−gd212(g(d1+d2))
This simplifies to:
h=d1−d212(d1+d2)
Combining the terms gives:
h=2d1(d1+d2)−d212(d1+d2)=d1d2d1+d2
Final Result
Thus, the height h of the wall in terms of d1 and d2 is:
h=d1d2d1+d2
The ball is projected at an angle of 45∘ with the horizontal. The horizontal and vertical components of the initial velocity u can be expressed as:
- ux=ucos(45∘)=u√2
- uy=usin(45∘)=u√2
The horizontal distance to the wall is d1. The time t1 taken to reach the wall can be calculated using the horizontal motion equation:
d1=ux⋅t1⟹t1=d1ux=d1√2u
The vertical position y of the ball when it reaches the wall can be calculated using the vertical motion equation:
y=uy⋅t1−12gt21
Substituting uy and t1:
h=u√2⋅d1√2u−12g(d1√2u)2
This simplifies to:
h=d1−gd212u2
The total horizontal distance the ball travels before hitting the ground is d1+d2. The range R of a projectile launched at an angle θ is given by:
R=u2sin(2θ)g
For θ=45∘:
R=u2g
Thus, we can set:
d1+d2=u2g
From the equation d1+d2=u2g, we can express u2:
u2=g(d1+d2)
Substituting u2 into the height equation:
h=d1−gd212(g(d1+d2))
This simplifies to:
h=d1−d212(d1+d2)
Combining the terms gives:
h=2d1(d1+d2)−d212(d1+d2)=d1d2d1+d2
Thus, the height h of the wall in terms of d1 and d2 is:
h=d1d2d1+d2
Topper's Solved these Questions
Similar Questions
A body is projected at angle of 45∘ to the horizontal . What is its velocity at the highest point ?
View SolutionA body projected from a point O at an angle θ, just crosses a wall y m high at a distance. x m from the point of projection and strikes the ground at Q beyond the wall as shown, then find height of the wall
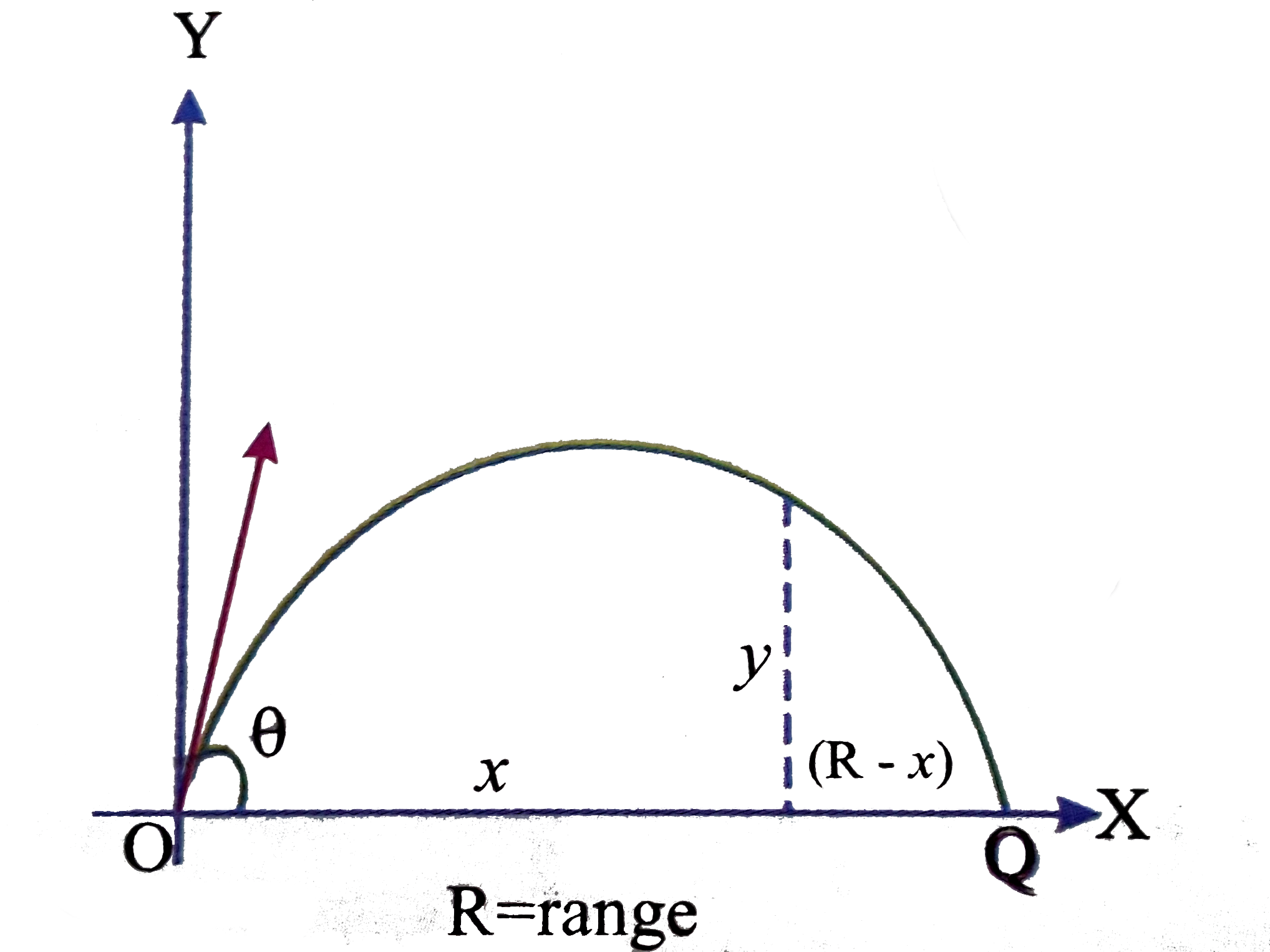 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A projectile is fired at an angle of 45∘ with the horizontal. Elevation angle of the projection at its highest point as seen from the point of projection is
A60∘Btan−1(12)Ctan−1(√32)D45∘ - Question 2 - Select One
A ball is projected with 20√2m/s at angle 45∘ with horizontal.The angular velocity of the particle at highest point of its journey about point of projection is
A0.1rad/sB1rad/sC0.3rad/sD0.4rad/s - Question 3 - Select One
A particle is projected at an angle θ with the horizontal . If angle of elevation of highest point of trajectory is ϕ when seen from point of projection, then
Atanϕ=2tanϕBtanθ=3tanϕCtanθ=2tanϕDtanθ=4tanϕ
A ball is projected from ground with a speed 70m/s at an angle 45∘ with the vertical so that it strikes a vertical wall at horizontal distance 4903m from the point of projection. If the ball returns back to the poin tof projection without any collision with ground then find the coefficient of restitution between the ball and wall.
View SolutionA ball projected from the ground with speed 10m/s at an angle of 45∘ with horizontal. It collides with a wall at a distance 2 m from the point of projection and returns to its original position. If the coefficient of restitution between the ball and wall is 1/x, find x.
 View Solution
View SolutionA particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA body projected just crosses a wall of 4m height,at 6m distance from the point of projection and it hits the ground at 12m away from the wall. The angle of projection is nearly
View Solution

