Three identical roads each of mass M and L are joined to form an equilateral triangle. Find the M.I. about an axis perpendicular to the plane of triangle and passing through
(a) center of triangle
(b) mid-point of a rod and
(c ) one vertex of triangle.
(a) Axis is passing through O and ⊥ar to the plane. M.I. of rod (1), about and axis passing through D and ⊥ar to the length (⊥ar to the plane) =ML212
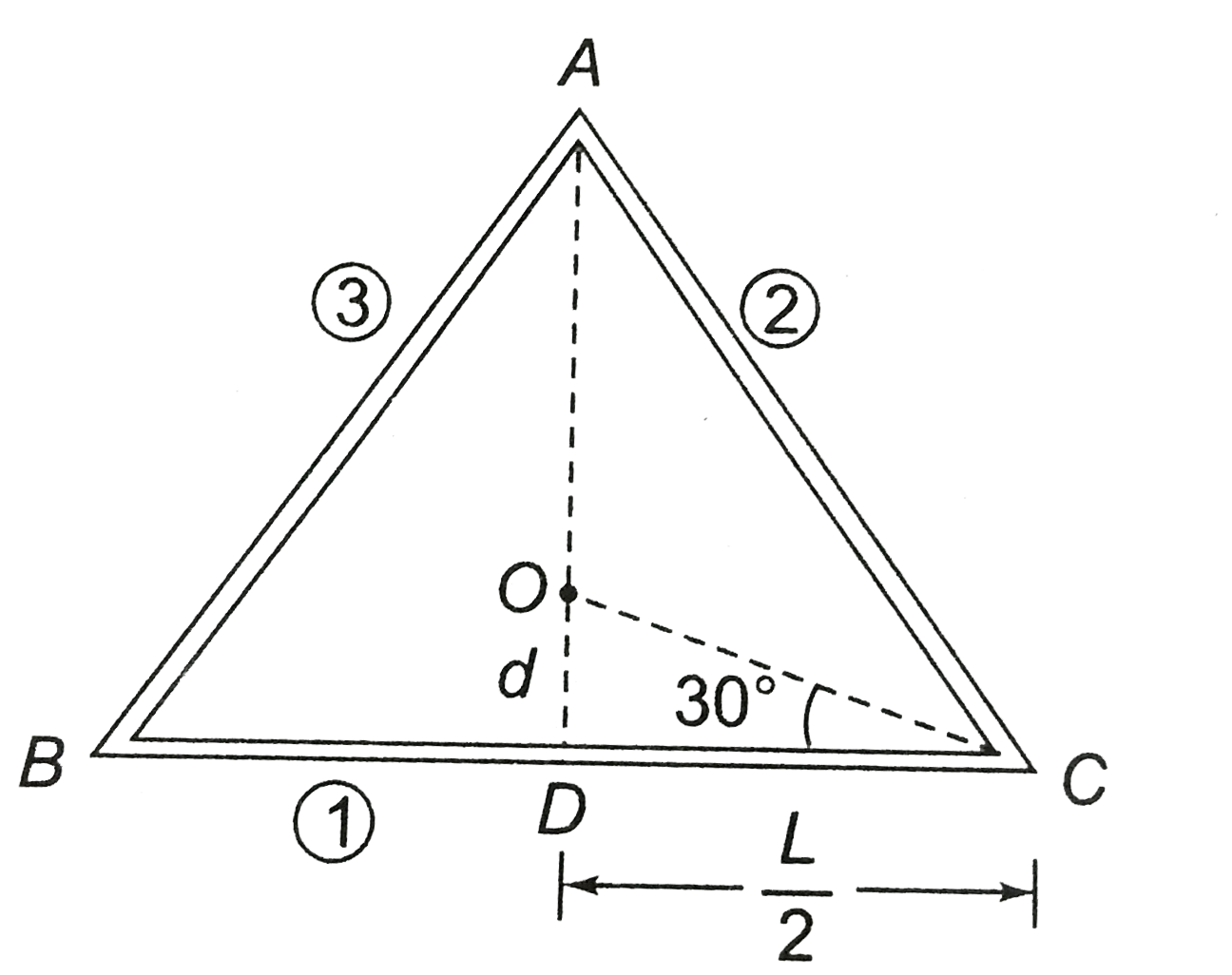
M.I. of rod (1), about axis through O
I1=ML212+Md2=ML212+M(12√3)2=ML26
{InΔODC,tan30∘=dL/2⇒d=L2√3}
Similar anyalysis for two other rods
I0=3I1=ML22
(b) 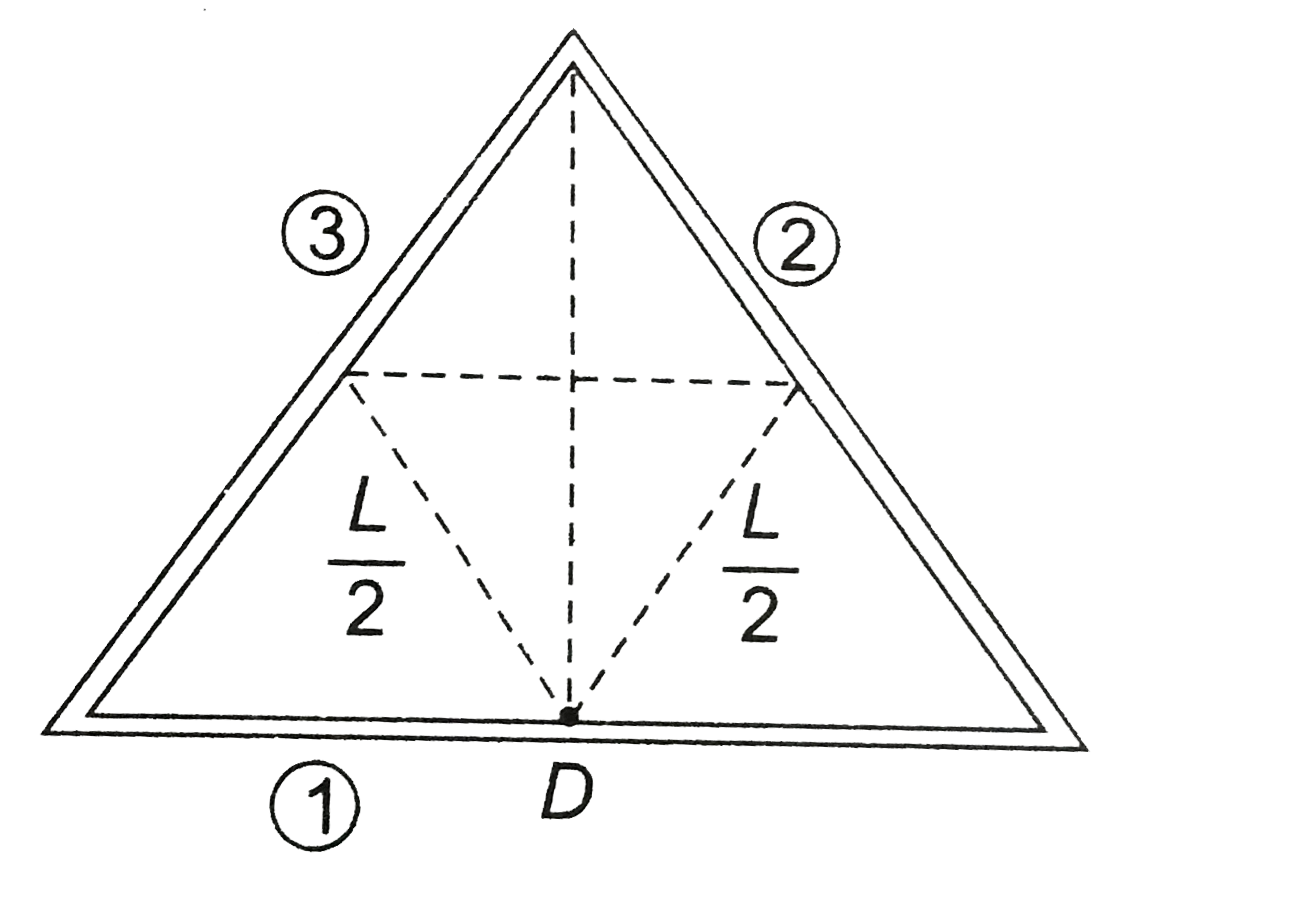
Axis is passing through D and ⊥ar to the plane
ID=I1+I2+I3
I1=ML212
I2=I3=ML212+M(L2)2=ML23
ID=ML212+ML23×2=34ML2
(If we join the mid-point of equilateral triangle of side L, we get another equilateral triangle of side L/2.)
(c) 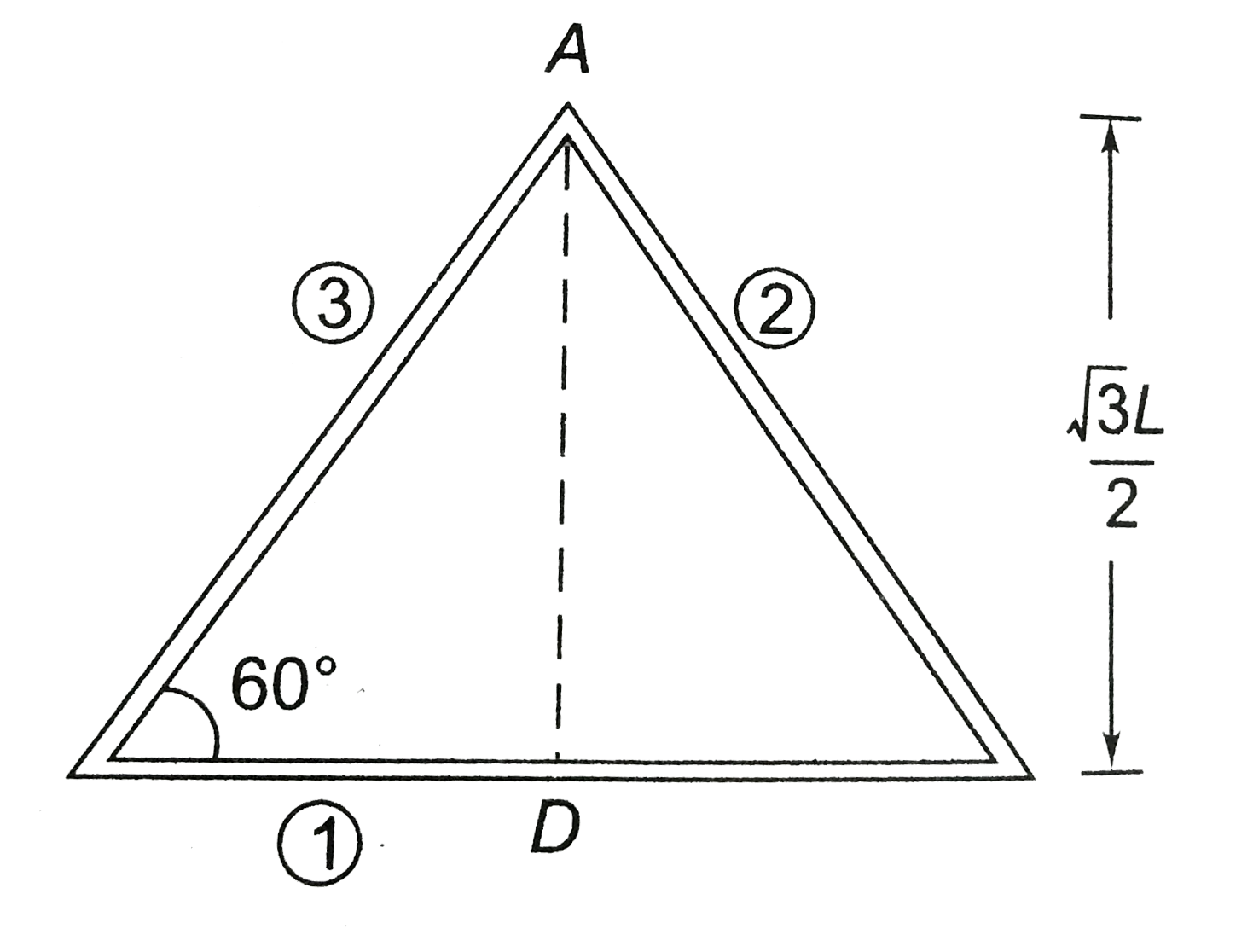
Axis is passing through A and ⊥ar to the plane. For rods (2) and (3), the axis through A is an axis passing through the end of rod and ⊥ar to the length
I2=I3=ML23
For rod (1)
I1=ML212+M(√3L2)2=5ML26
IA=I1+I2+I3=(56+13+13)=9ML26=3ML22
Alternatively for parts (b) and (c)
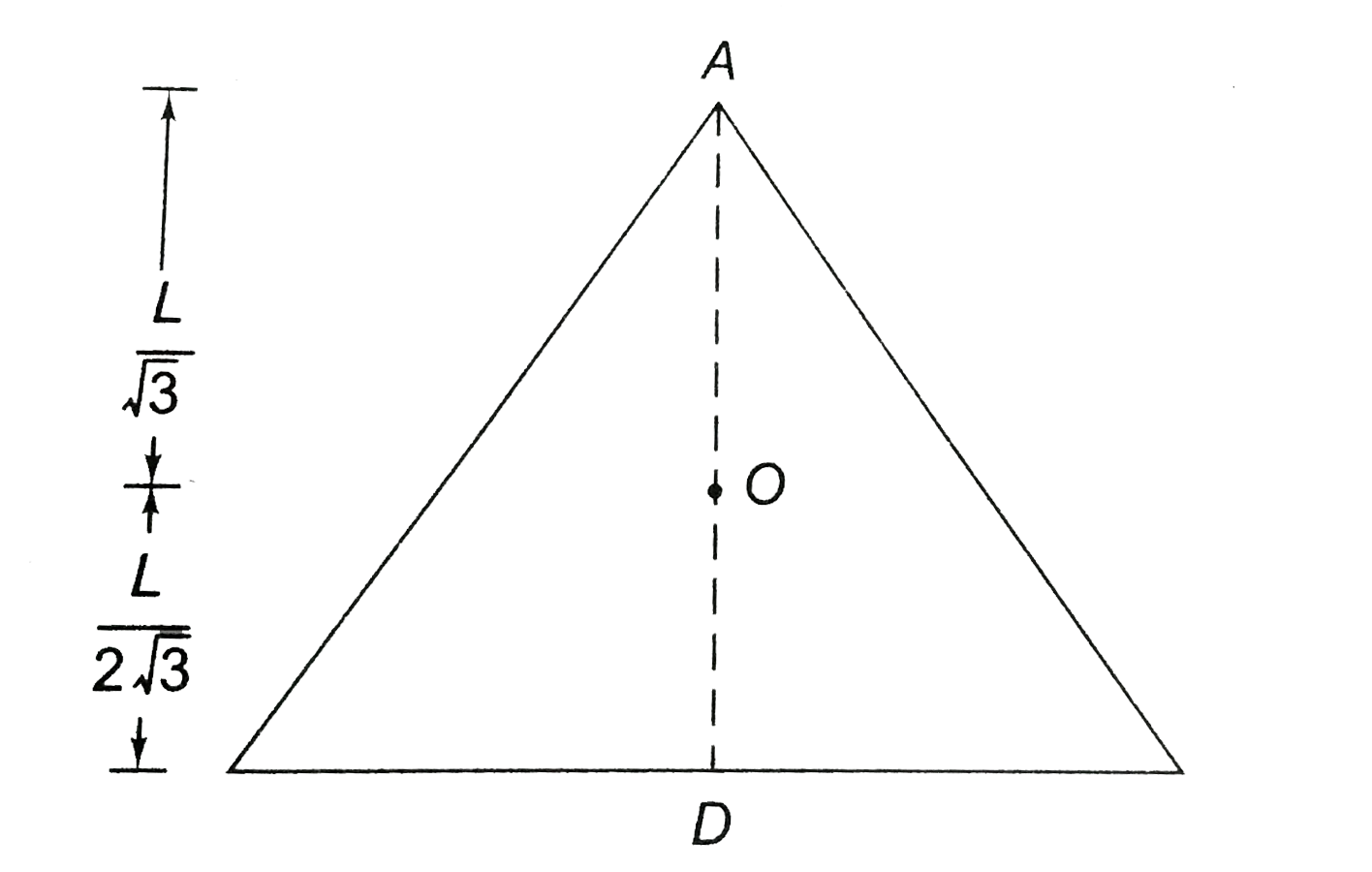
O is the center of mass of system of rods
ID=IO+3M(L2√3)2=ML22+3ML212=3ML24
IA=IO+3M(L√3)2=ML22+3ML23=3ML22
Topper's Solved these Questions
Similar Questions
Three identical rods each of mass M, length l are joined to form an equilateral ΔABC . Find the Moment of Inertia about BC as shown.
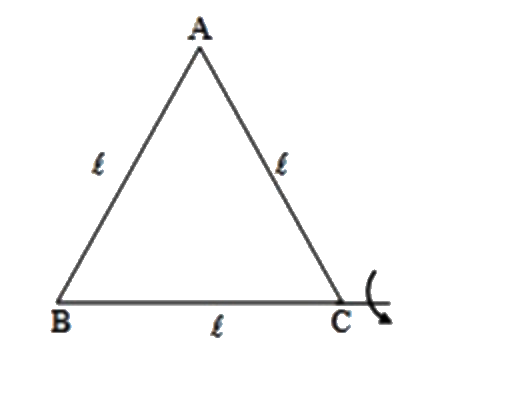 View Solution
View SolutionThree thin, uniform, indentical rods each or mass M and length L are joined as shown. Find the M.I. about an axis passing through O and perpendicular to the plane.
View Solution
Knowledge Check
- Question 1 - Select One
Three identical rods, each of mass m and length l, form an equaliteral triangle. Moment of inertia about one of the sides is
 Aml26Bml2C3ml24D2ml23
Aml26Bml2C3ml24D2ml23 - Question 2 - Select One
Three identical rods, each of length L, are joined to form a rigid equilateral triangle. Its radius of gyration about an axis passing thorugh a corner and perpendicular to plane of triangle is
AL√2BL22CL√2D2L - Question 3 - Select One
Three identical rods, each of length L, are joined to from a rigid equilateral triangle. Its radius of gyration about an axis passing through a corner and perpendicular to plane of triangle is
AL√2BL√3CL2DL3
A rod of mass 3 kg and of length 3m is bent in the form of an equilateral triangle. The moment of inertia of the triangle about a vertical axis perpendicular to plane and passing through centre of mass is
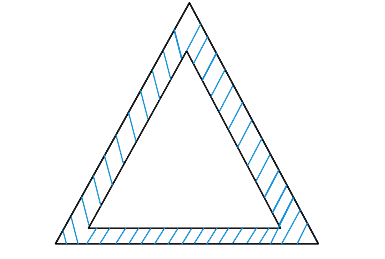 View Solution
View SolutionThree identical rods, each of length l, are joined to form a rigid equilateral triangle. Its radius of gyration about an axis pássing through a corner and perpendicular to the plane of the triangle is l√x. Find x.
View SolutionA rod of mass Mkg and length Lm is bent in the from of an equilateral tringle as shown in Gig. The moment of inertia of the triangle about a vertical axis perpendicular to the plane of the triangle and passing through the centre (in units of kgm2) is.
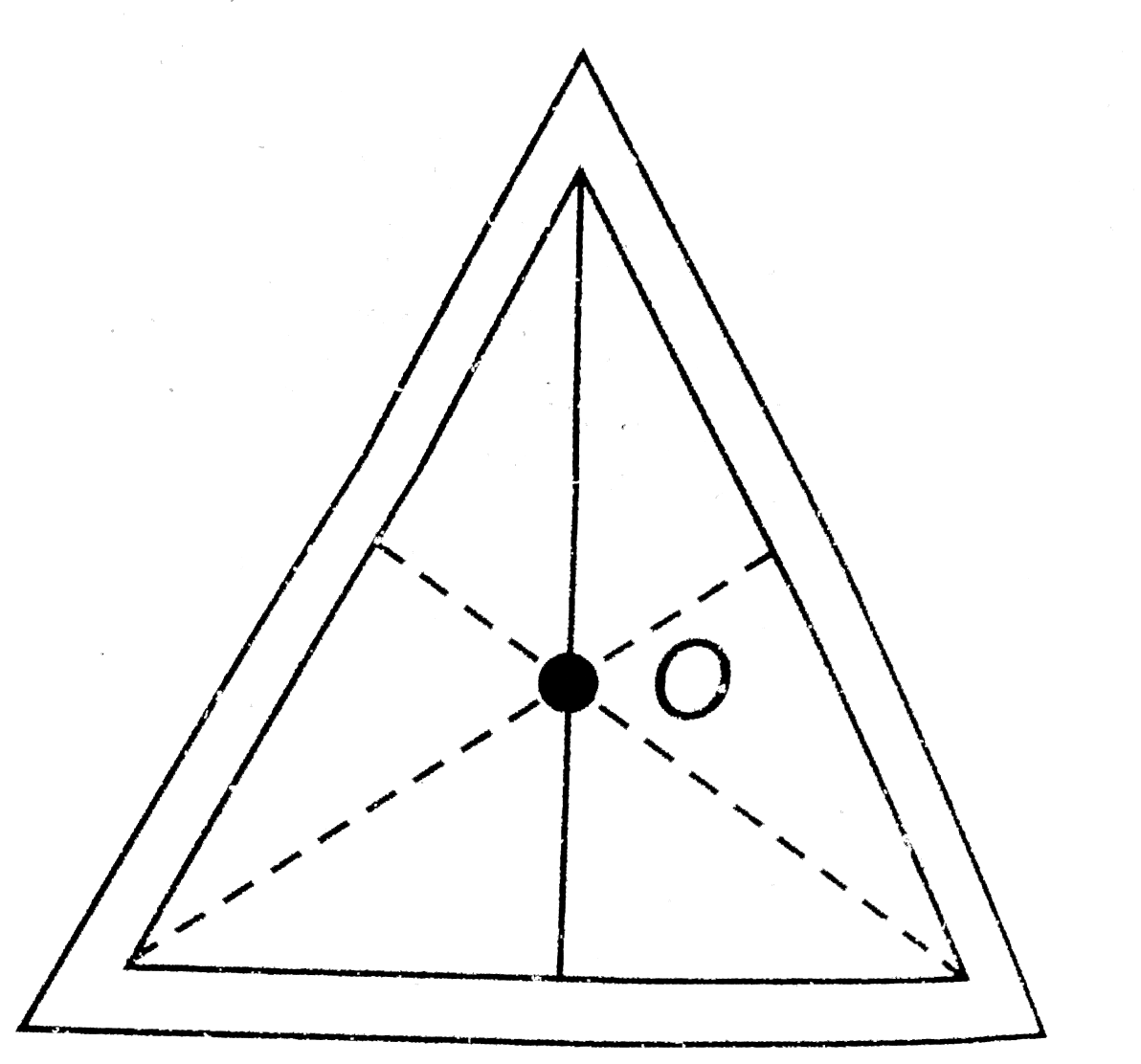 .View Solution
.View SolutionThree identical bar magnets each of magnetic moment M are placed in the form of an equilateral triangle as shown.
 View Solution
View Solution3 particles of mass 10 gm each are placed at the corners of an equilateral triangle of side 5 cm. The M.I. of the system about a perpendicular axis to the plane passing through a corner of the triangle will be : (in kg-m2)
View Solution

