From a disc of radius r1, a concentric disc of radius r2 is removed . The mass of the remaining portion is m. Find the M.I. of the remaining about an axis passing through the center of mass and perpendicular to the plane.
To find the moment of inertia (M.I.) of the remaining portion of the disc about an axis passing through the center of mass and perpendicular to the plane, we can follow these steps:
Step 1: Define the Masses
Let:
- mA = mass of the original disc of radius r1
- mB = mass of the removed concentric disc of radius r2
- m = mass of the remaining portion, which is given.
From the problem, we have:
m=mA−mB
Step 2: Calculate the Masses Using Density
Since both discs are made of the same material, their densities are equal. The density ρ can be expressed as:
ρ=mV
For a disc, the volume can be considered as the area times thickness (assuming uniform thickness). Thus, we can write:
mA=ρ⋅AreaA=ρ⋅πr21
mB=ρ⋅AreaB=ρ⋅πr22
Since the densities are equal, we can equate the ratios:
mAπr21=mBπr22
This simplifies to:
mA=mB⋅r21r22
Step 3: Substitute for Masses
From the equation m=mA−mB, we can substitute mA:
m=mB⋅r21r22−mB
m=mB(r21r22−1)
From this, we can solve for mB:
mB=mr21r22−1
Step 4: Find the Moment of Inertia
The moment of inertia of a disc about an axis through its center is given by:
IA=12mAr21
And for the removed disc:
IB=12mBr22
The moment of inertia of the remaining portion is:
I=IA−IB
Substituting the values:
I=12mAr21−12mBr22
Step 5: Substitute for mA and mB
Now we can substitute mA and mB into the equation:
I=12(m+mB)r21−12mBr22
Step 6: Simplify the Expression
Substituting the value of mB from Step 3 into the moment of inertia equation and simplifying will give us the final expression for the moment of inertia of the remaining portion.
Final Expression
After performing the algebraic simplifications, we arrive at:
I=12m(r21+r22)
Let:
- mA = mass of the original disc of radius r1
- mB = mass of the removed concentric disc of radius r2
- m = mass of the remaining portion, which is given.
m=mA−mB
Since both discs are made of the same material, their densities are equal. The density ρ can be expressed as:
ρ=mV
mA=ρ⋅AreaA=ρ⋅πr21
mB=ρ⋅AreaB=ρ⋅πr22
mAπr21=mBπr22
mA=mB⋅r21r22
From the equation m=mA−mB, we can substitute mA:
m=mB⋅r21r22−mB
m=mB(r21r22−1)
mB=mr21r22−1
The moment of inertia of a disc about an axis through its center is given by:
IA=12mAr21
And for the removed disc:
IB=12mBr22
I=IA−IB
Substituting the values:
I=12mAr21−12mBr22
Now we can substitute mA and mB into the equation:
I=12(m+mB)r21−12mBr22
Substituting the value of mB from Step 3 into the moment of inertia equation and simplifying will give us the final expression for the moment of inertia of the remaining portion.
After performing the algebraic simplifications, we arrive at:
I=12m(r21+r22)
Topper's Solved these Questions
Similar Questions
From a thin uniform disc of radius 2R. Another disc of diameter 2R is removed. The mass of the remaining portion is m. Find the M.I. of the shaded portion about an axis passing through O and pependicular to the plane.
View SolutionFrom a semicircular disc of radius r1, another semicircular disc of radius r2 is removed. Find the c.m. of the remaining position.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
From a circular disc of mass M and radius R, a part of 60∘ is removed. The M.I. of the remaining portion of disc about an axis passing through the center and perpendicular to plane of disc is
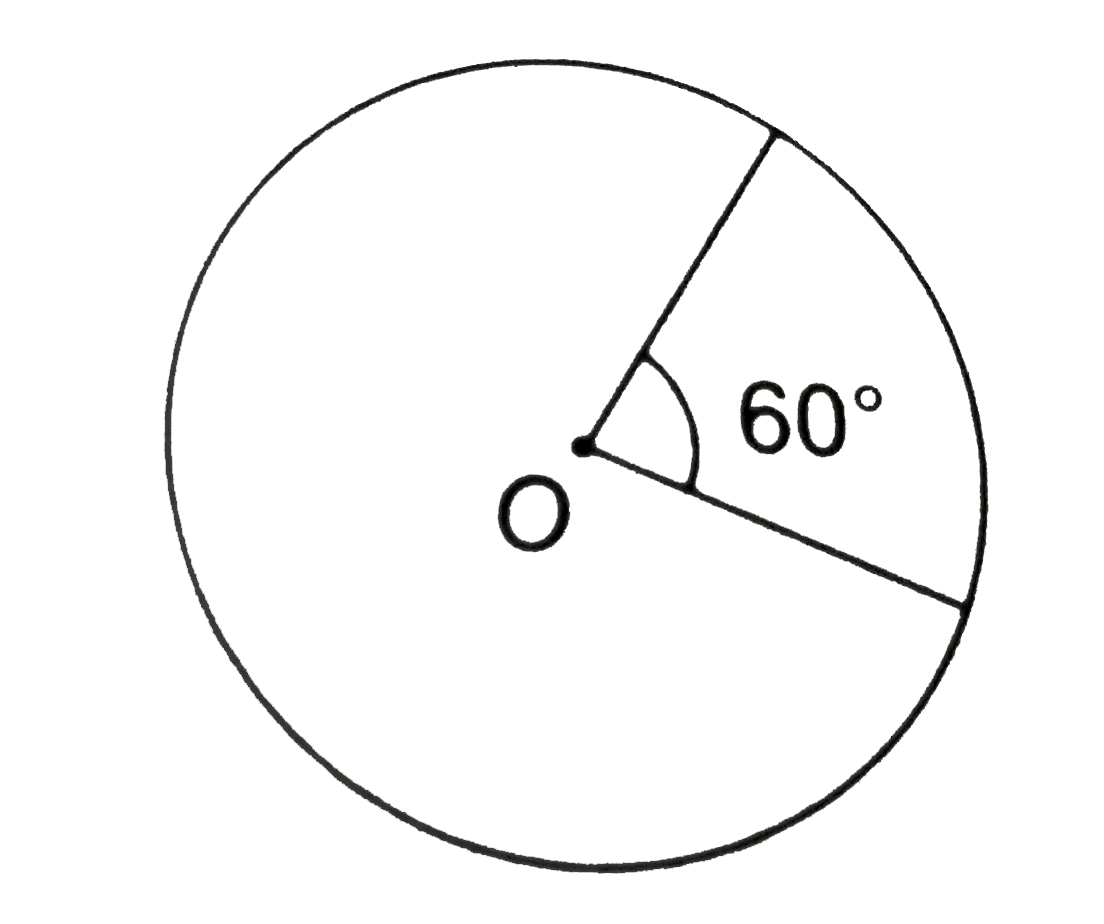 A56MR2B53MR2C512MR2D524MR2
A56MR2B53MR2C512MR2D524MR2 - Question 2 - Select One
Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
A1/2MR2BMR2C1/4MR2D3/4MR2 - Question 3 - Select One
The radius of gyration of a uniform disc of radius R, about an axis passing through a point R2 away from the centre of disc, and perpendicular to the plane of disc is:
A√12RB√2RC√32RD√34R
From a circular disc of radius R, another disc of diameter R is removed. Locate c.m. of the remaining portion.
 View Solution
View SolutionFrom a ring of mass M and radius R, a 30o sector is removed. The moment of inertia of the remaining portion of the ring, about an axis passing through the centre and perpendicular to the plane of the ring is
View SolutionCalculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionOut of a disc of mass M and radius R a concentric disc of mass m and radius r is removed. The M.I. of the remaining part about the symmetric axis will be :
View Solution

