A point mass m is a distance x from the centre of mass M and radius R on its axis. Find the gravitational force between the two. What will this force be if x>>R and x<<R? For what value of x is the force maximum?

Gravitational field due to ring at P
E=GMx(R2+x2)3/2
Force on m
F=mE=GMmx(R2+x2)3/2
If x<<R,F=GMmxR3
x>>R,F=GMmx(R2+x2)3/2=GMmxx3(1+R3x2)3/2
=GMmx2
F=GMmx(R2+x2)3/2
For F to be maximum,
dFdx=0⇒(R2+x2)3/2.1−x.32(R2+x2)1/2.2x=0
(R2+x2)−3x2=0
x±R√2
Fmax=GMm(R/√2)(R2+x2)3/2=GMm(R/√2)3R22.√3R√2=2FMm3√3R2
Topper's Solved these Questions
Similar Questions
A particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View SolutionFrom a solid sphere of mass M and radius R, a solid sphere of radius R/2 is removed as shown. Find gravitational force on mass m as shown
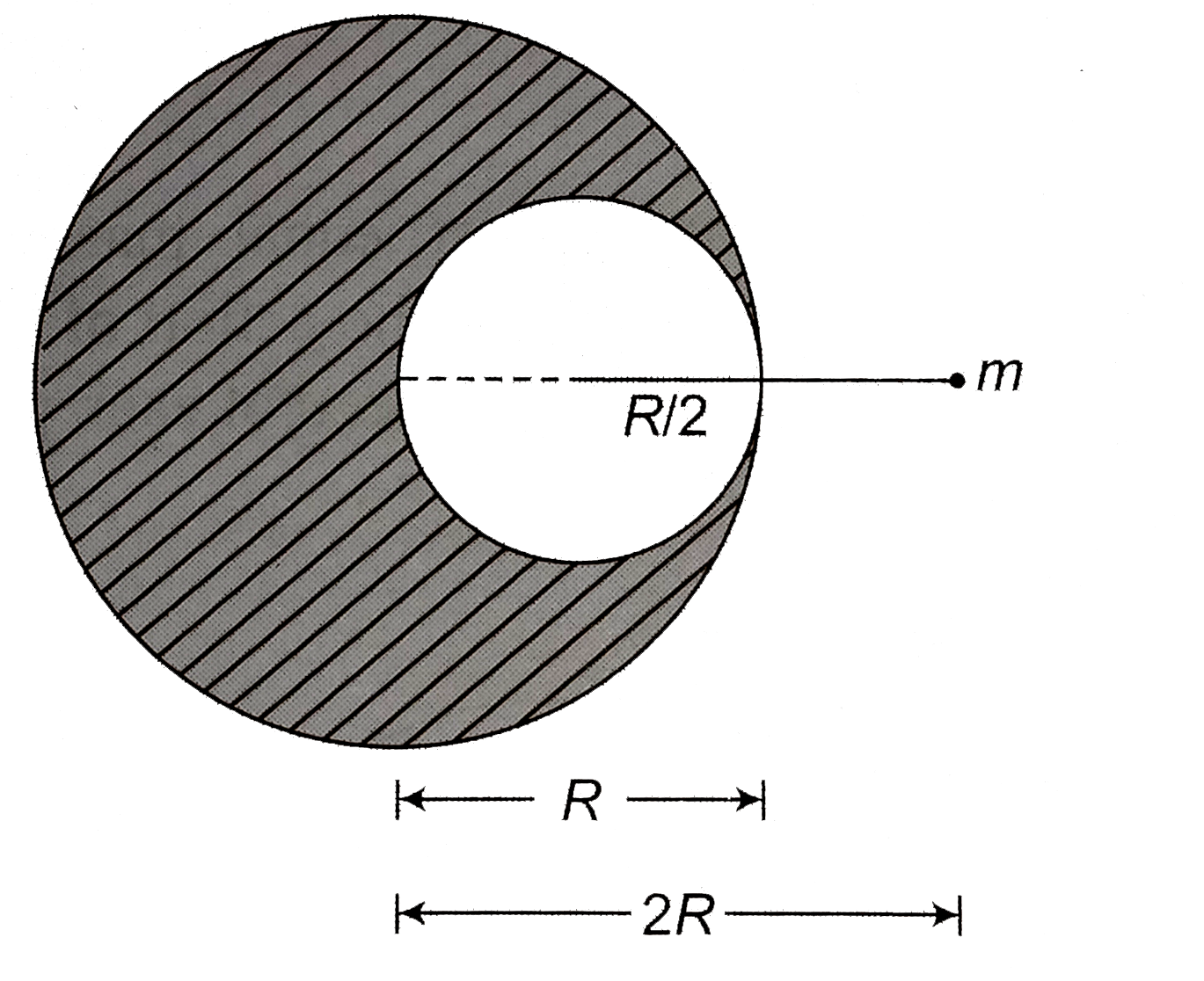
View Solution
Knowledge Check
A point mass m0 is placed at distance R/3 from the centre of spherical shell of mass m and radius R. the gravitational force on the point mass m0 is
A4Gmm0R2BzeroC9Gmm0R2DNone of theseP is a point at a distance r from the centre of a spherical shell of mass M and radius a, where r<a. The gravitational potential at P is
A−GMrB−GMaC−GMra2D−GM(a−ra2)A particle of mass m is located inside a spherical shell of mass M and radius R. The gravitational force of attraction between them is
AGMmRBGMmR2C−GMmR2D0
A particle of mass m is ocated at a distance r from the centre of shell of mass M and radius R. The force between the shell and mass F(r). The plot of F(r) ve r is :
View SolutionA mass m is placed at point P lies on the axis of a ring of mass M and radius R at a distance R from its centre. The gravitational force on mass M is
View SolutionA particle of mass m lies at a distance r from the centre of earth. The force of attraction between the particle and earth as a function of distance is F(r ),
View SolutionThe gravitational force between two particles of masses m1 and m2 separeted by the same distance, then the gravitational force between them will be
View SolutionA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
View Solution
