Two uniform circular discs, each of mass $1 k g$ and radius $20 c m$ , are kept in contant about the tangent passing through the point of contact. Find the moment of inertia of the system about the tangent passing through the point of contact.

Video Solution

Text Solution

Verified by Experts

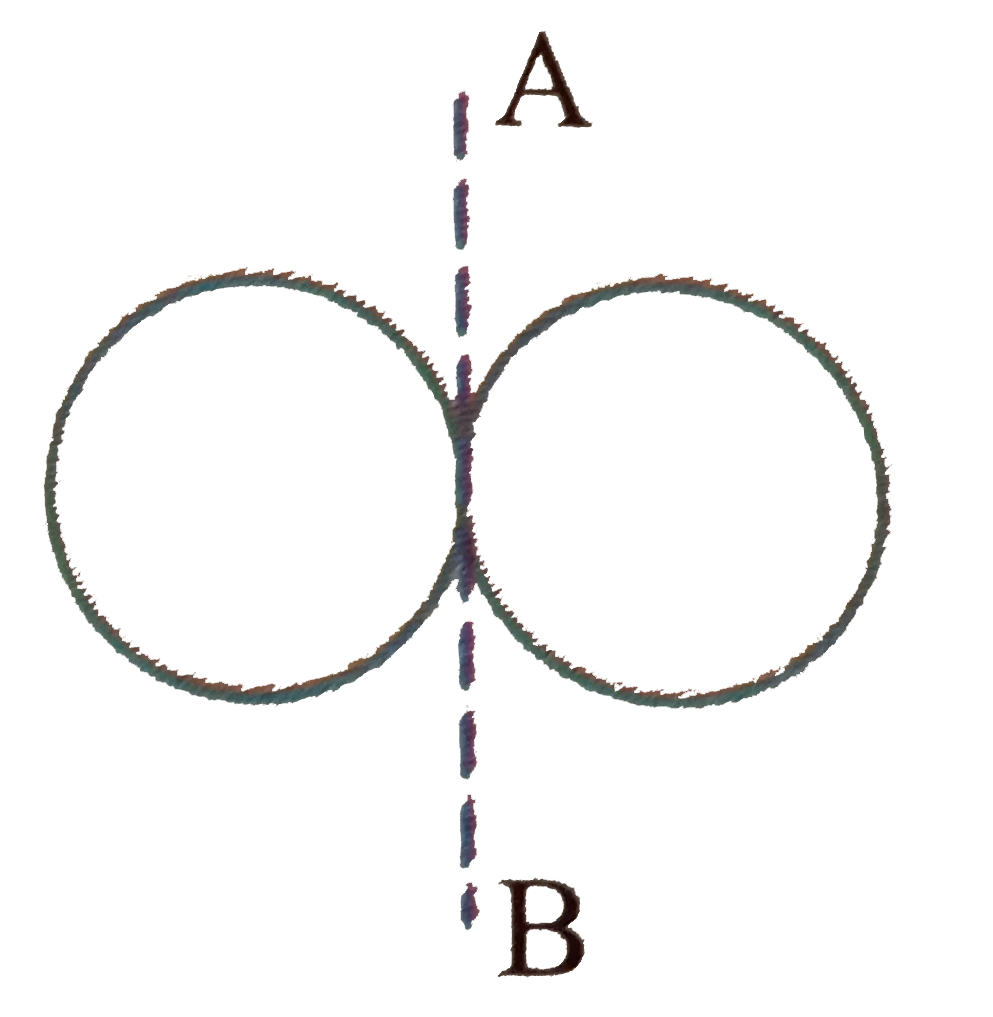
$M a s s M = 1 k g, r = 20 \times 10^{- 2} m$
$I_{1} = \frac{M R^{2}}{4} + M R^{2} = \frac{5 M R^{2}}{4}$
Similarly, $I_{2} = \frac{5 M R^{2}}{4}, I = I_{1} + I_{2}$
$∴ I = \frac{10 M R^{2}}{4} = \frac{10 \times 1 \times {(20 \times 10^{- 2})}^{2}}{4} = 0.1 k g m^{2}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Knowledge Check

Question 1 - Select One
Two identical circular plates each of mass $M$ and radius $R$ are attached to each other with their planes $⊥^{r}$ to each other. The moment of inertia of system about an axis passing through their centres and the point of contact is
A $\frac{M R^{2}}{4}$
B $\frac{5 M R^{2}}{4}$
C $\frac{3}{4} M R^{2}$
D $M R^{2}$
Question 2 - Select One
The tangent at any point of the circle is _______to the radius through the point of contact.
AA)parallel
BB)Intersecting
CC)perpendicular
DD)equal
Question 3 - Select One
Two thin uniform circular rings each of radius 10 cm and mass 0.1 kg are arranged such that they have a common centre and their planes are perpendicular to each other. The moment of inertia of this system about an axis passing through their common centre and perpendicular to the plane of one of the rings in $k g - m^{2}$ is
A $1.5 \times 10^{- 3}$
B $5 \times 10^{- 3}$
C $1.5 \times 10^{- 6}$
D $18 \times 10^{- 4}$

Recommended Questions

Home
Ask Doubt
Profile