Find the moment of inertia of a pair of spheres, each having a mass m and radius r kept in contact about the tangent passing through the point of contact.
The correct Answer is:A, B, D
The two bodies of mass m and radius are movgn along the common tangent. Therefore moment of inertial of th first body about XY tangent
mr2+25mr2
=momentof∈ertiaofthesenconbodyXYtan≥nt<br>=mr^2+2/5mr^2=7/5mr^2<br>Therefore,≠tmomentof∈ertia<br>=7/5mr^2+7/5mr^2
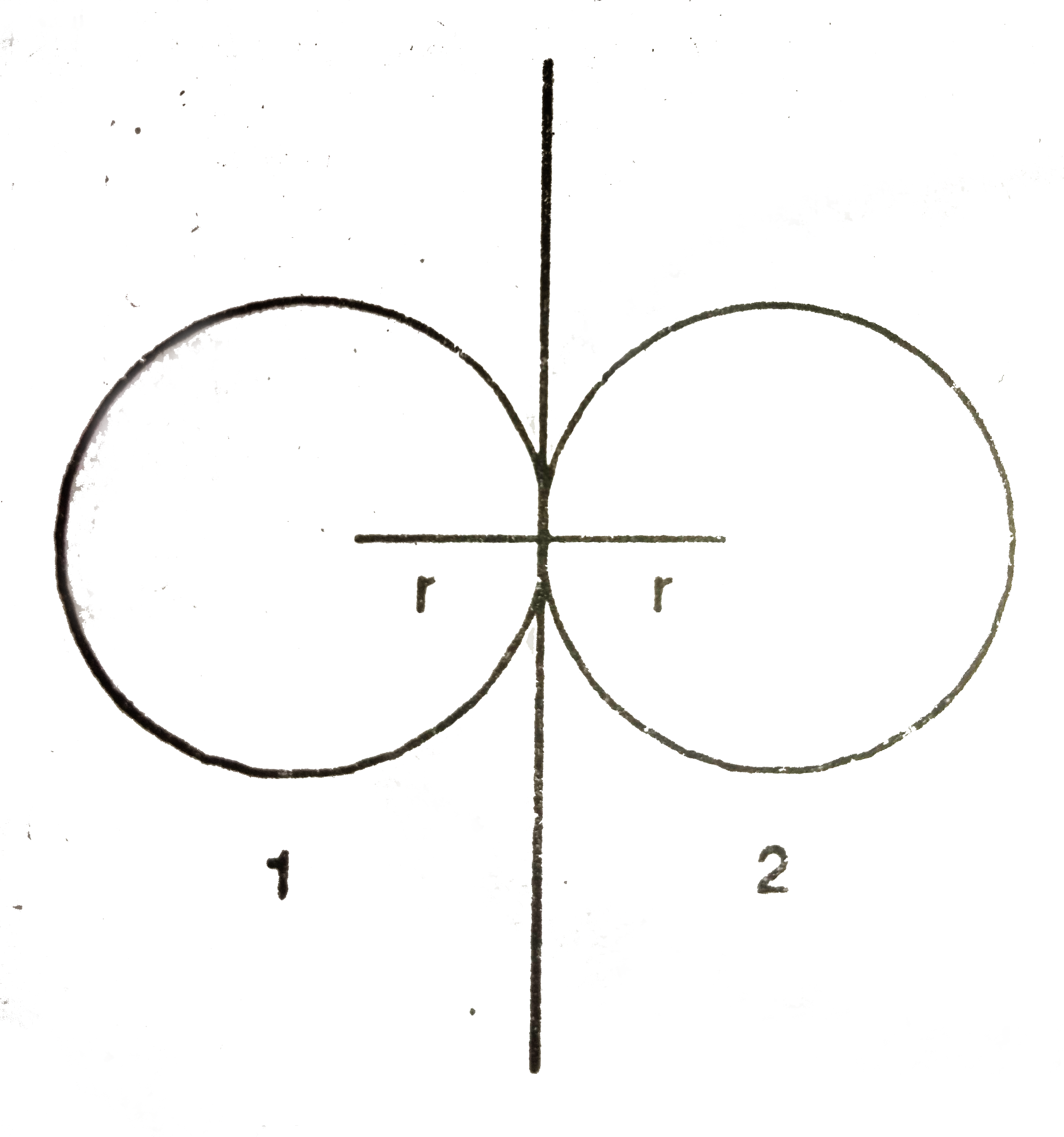
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
Two uniform circular discs, each of mass 1kg and radius 20cm, are kept in contant about the tangent passing through the point of contact. Find the moment of inertia of the system about the tangent passing through the point of contact.
View SolutionUsing the formula for the moment of inertia of a uniform sphere, find the moment of inertia of a thin spherical layer of mass m and radius R relative to the axis passing through its centre.
View Solution
Knowledge Check
The moment of inertia of a solid sphere of mass ‘M' and radius 'R' about a tangent to the sphere is
A25MR2B63MR2C45MR2D75MR2The moment of inertia of a uniform semicircularwire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is :
A12Mr2BMt2C25Mr2D14Mr2
Find moment of inertia of a uniform solid sphere (mass m, radius r) about a tangent.
View SolutionFind the moment of inertia of a uniform sphere of mass m and radius R about a tangent if the spheres (1) solid (ii) hollow?
View SolutionFind out the moment of inertia of the circular arcs shown, each having mass M, radius R and having uniform mass distribution about an axis passing through the centre and perpendicular to the plane ?

View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View SolutionCalculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
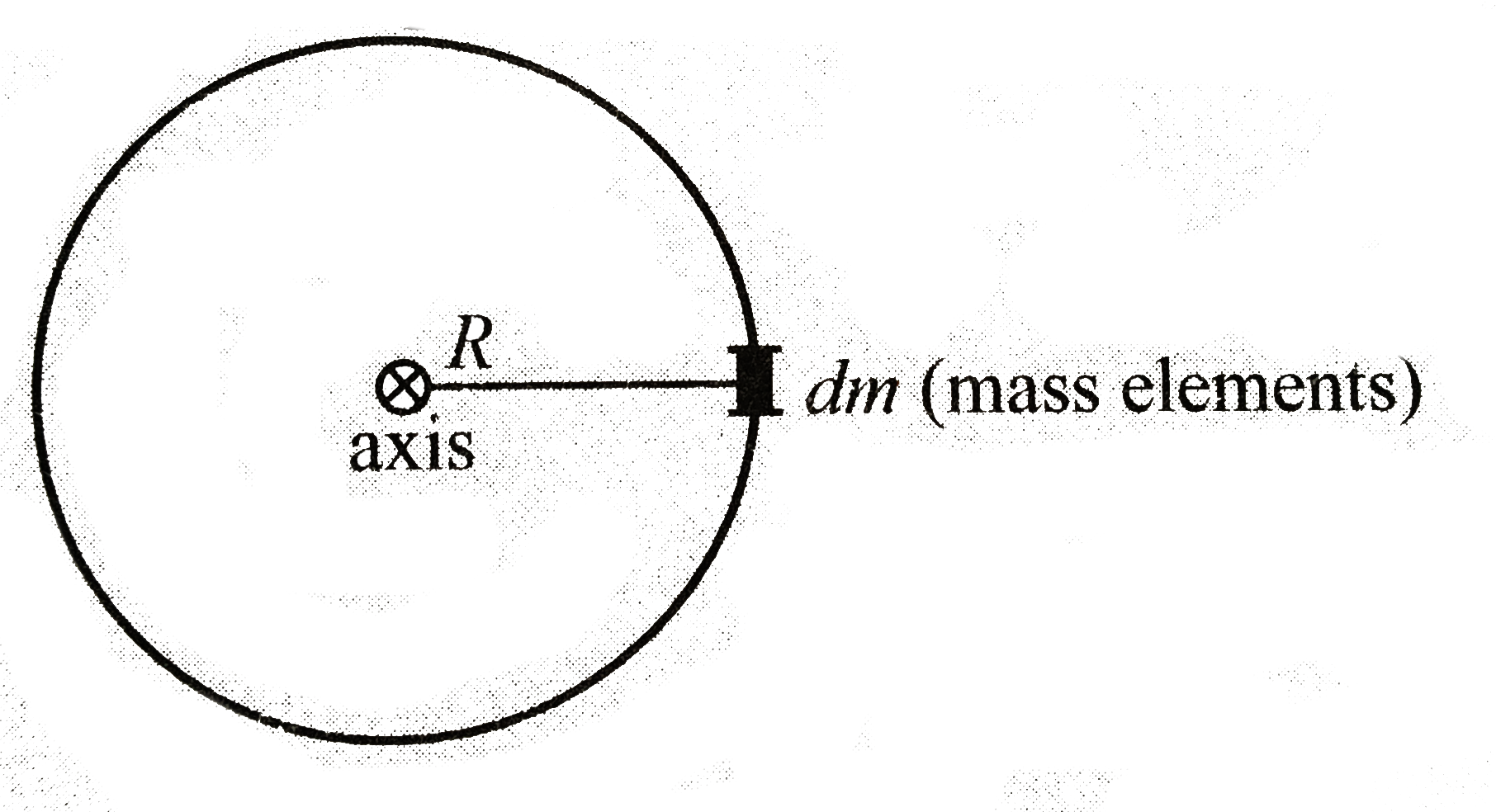
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be :-

View Solution
