A motor rotates a pulley of radius 25cm at 20rpm. A rope around the pulley lifts a 50kg block, What is the power output of the motor?
The tension in the rope is equal to the weight since there in no acceleration. Thus T=500N therefore, τ=TR=(500)(0.25)=125Nm
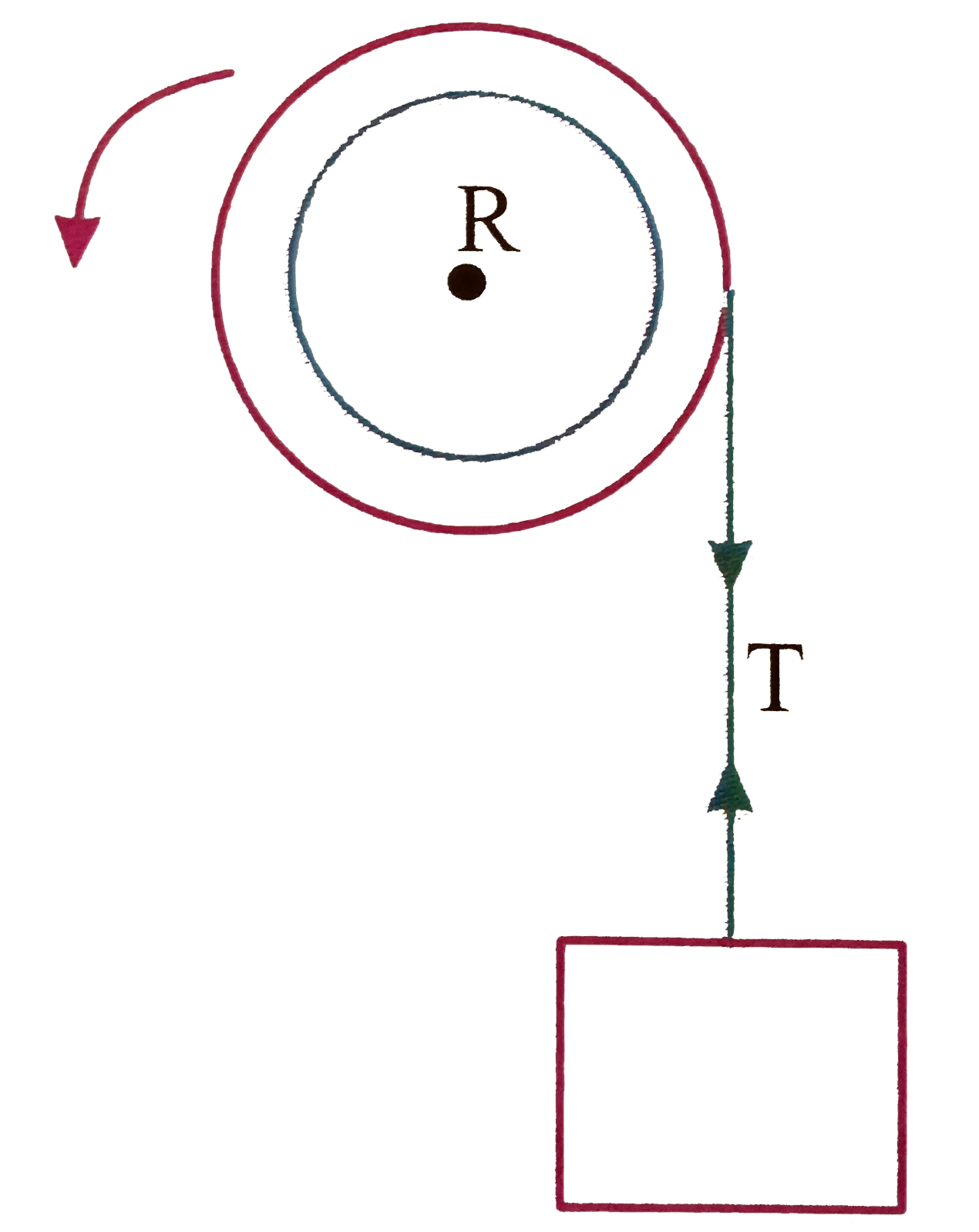
Angular velocity, ω=2πN602π(20)60=2π3rads−1
The power required is
P=τω=(125Nm−1)(2π3rads−1)=261W
Similar Questions
A bucket is being lowered down into a well through a rope passing over a fixed pulley of radius 10 cm. Assume that the rope does not slip on the pulley. Find the angular velocity and angular acceleration of the pulley at an instant when the bucket is going down at at speed of 20 cm/s adn has an accelertion of 4.0ms2.
View SolutionA motor generates an output power of 220 W at an angular velocity of 2100 rpm. Calculate the torque ( in Nm ) produced by the motor ? [ Take π=227]
View Solution
Knowledge Check
As shown in the given figure, two blocks are connected by a rope that passes over a set of pulleys. One block has a weight of 412 N, and the other has a weight of 908 N. The rope and the pulleys are massless and there is no friction. What is the acceleration of the lighter block ?
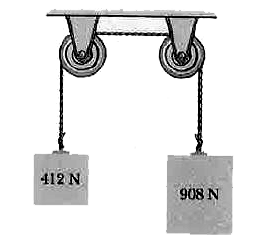 A4.44 m/s2B6.34 m/s2C2.66 m/s2D3.68 m/s2
A4.44 m/s2B6.34 m/s2C2.66 m/s2D3.68 m/s2Ten litre of water per second is lifted from well through 20m and delivered with a velocity of 10 m/s, then the power of the motor is :
A1.5 kWB2.5 kWC3.5 kWD4.5 kWA 45 N brick is suspended by a light string from a 2.0 kg pulley. The brick is released from rest and falls to the floor below as the pulley rotates through 5.0 rad. The pulley may be considered a solid disk of radius 1.5 m. What is the angular speed of the pulley?
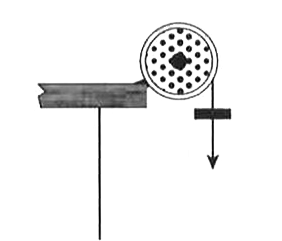 A17 rad/sB8.1 rad/sC15 rad/sD7.3 rad/s
A17 rad/sB8.1 rad/sC15 rad/sD7.3 rad/s
The force needed to pull the tape through an audio cassette player is 0.98 N. In operation the tape travels at a constant speed of 2.5 cm/s The motor consumes a power of 1.8 W. what percentage of the power input to the motor is required to pull the tape at its operating speed ?
View SolutionSuppose the smaller pulley of the previous problem has its radius 5.0 cm and moment of inertia 0.10 kg-m^2. Find the tension in the part of the string joiningk the pulleys.
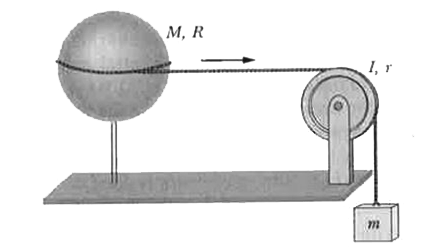
View SolutionA uniform spherical shell of mass M = 4.5 kg and radius R = 8.5 cm can rotate about a vertical axis on frictionless bearings (Fig. 10-69). A massless cord passes around the equator of the shell, over a pulley of rotational inertia I=3.0×10−3kg⋅m2 and radius r = 5.0 cm, and is attached to a small object of mass m = 0.60 kg. There is no friction on the pulley's axle, the cord does not slip on the pulley. What is the speed of the object when it has fallen 82 cm after being released from rest? Use energy considerations.
View SolutionA motor is to be used to lift water from a depth of 10 m & discharging it on ground level at a speed 10m/s if 2 kg of water is lifted per second & the efficiency of motor is 40% The minimum power of the motor should be nearly :
View Solution
A small pulley of radius 20 cm and moment of inertia 0.32kg−m2 is used to hang a 2 kg mass with the help of massless string. If the block is released, for no slipping condition acceleration of the block will be
View Solution
