Find the value of the thermal resistance of the non-uniform cylindrical rod of thermal conductivity K and length I as shown in
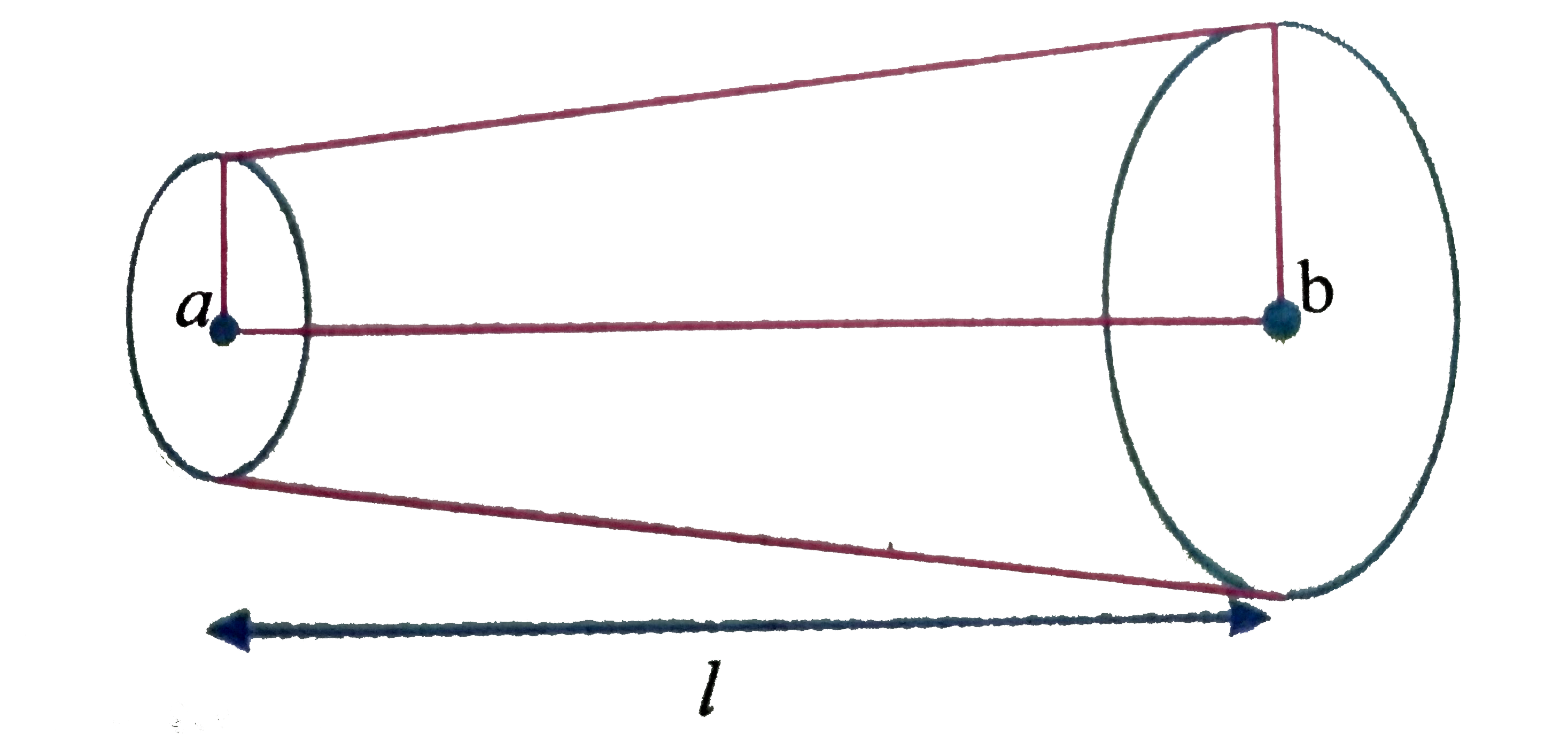 .
.
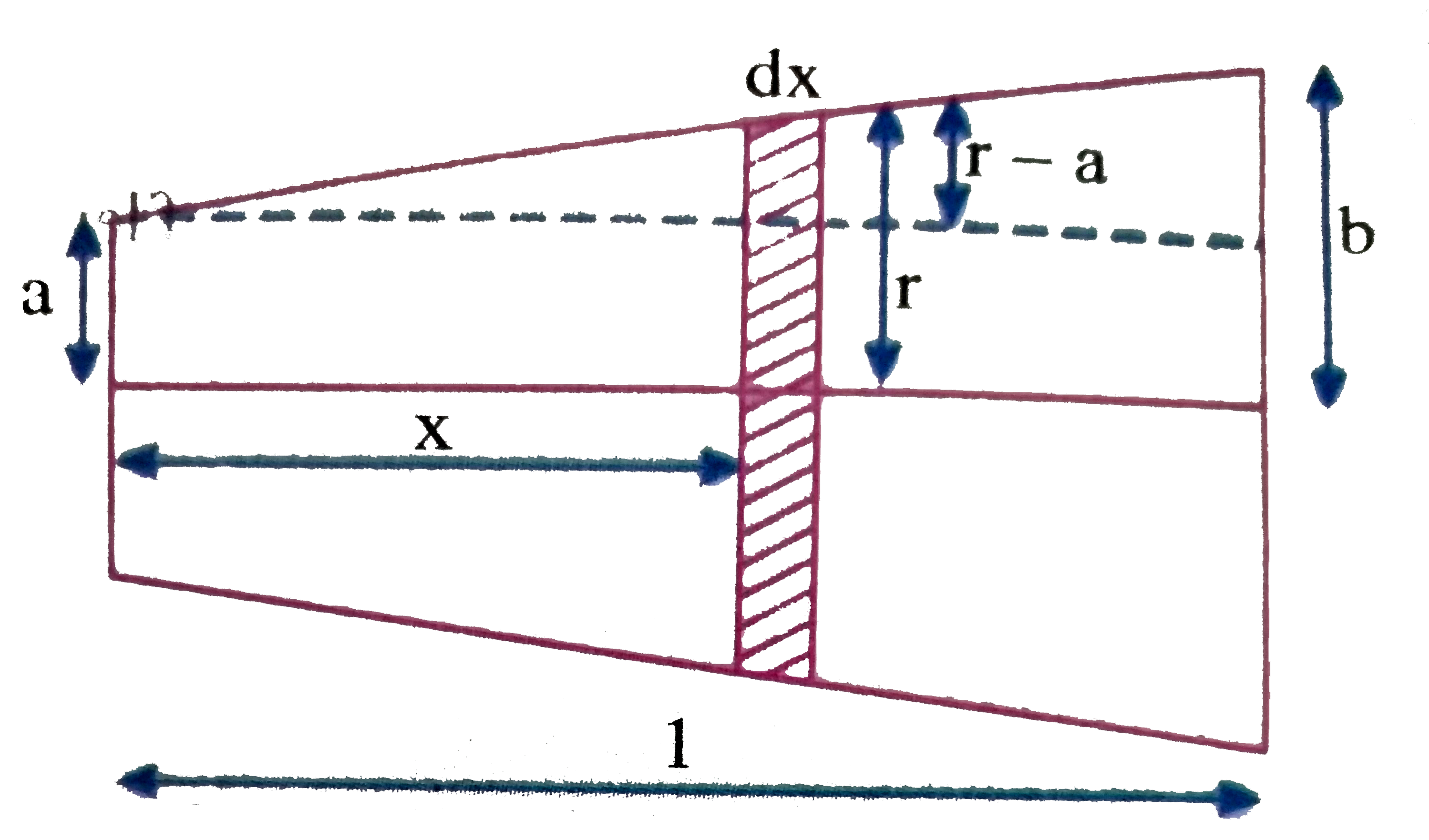
Consider a small element of width dx at a distance x from one end as shown in From similar triangles
r−ax=b−ar⇒r=a+(b−al)x
Thermal resistance of the cylinder is
R=l∫0dxKπr2=1Kπl∫0[a+(b−al)x]2−dx
=1Kπ⎡⎢
⎢
⎢⎣(a+(b−al)x)−1−(b−al)⎤⎥
⎥
⎥⎦l0
=lKπ(a−b)[1b−1a]=lKπab
 .
.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - C .U .Q89videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL -1 (C.W)1videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise187videos
THERMODYNAMICS
- BOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - STATEMENT TYPE QUESTION23videos
UNITS AND MEASUREMENTS
Similar Questions
Knowledge Check
- Question 1 - Select One
S.I unit of thermal conductivity is
AJsm−1K−1BJs−1m−1K−1CJs−1m−2K,DJs−1m−1K. - Question 2 - Select One
The thermal conductivity of a rod depends on
AlengthBmassCarea of cross sectionDmaterial of the rod. - Question 3 - Select One
Steady state temperature are shown in the diagram. Find ratio of thermal conductivity k1k2 if length of all rods are same
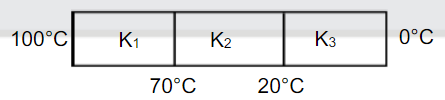 A0.12638888888889B0.12847222222222C0.16805555555556D0.20972222222222
A0.12638888888889B0.12847222222222C0.16805555555556D0.20972222222222
Thermal resistance R of a conductor of length l cross section A and conuctivity K is
View SolutionFind equivalent thermal conductivity in the following cases:
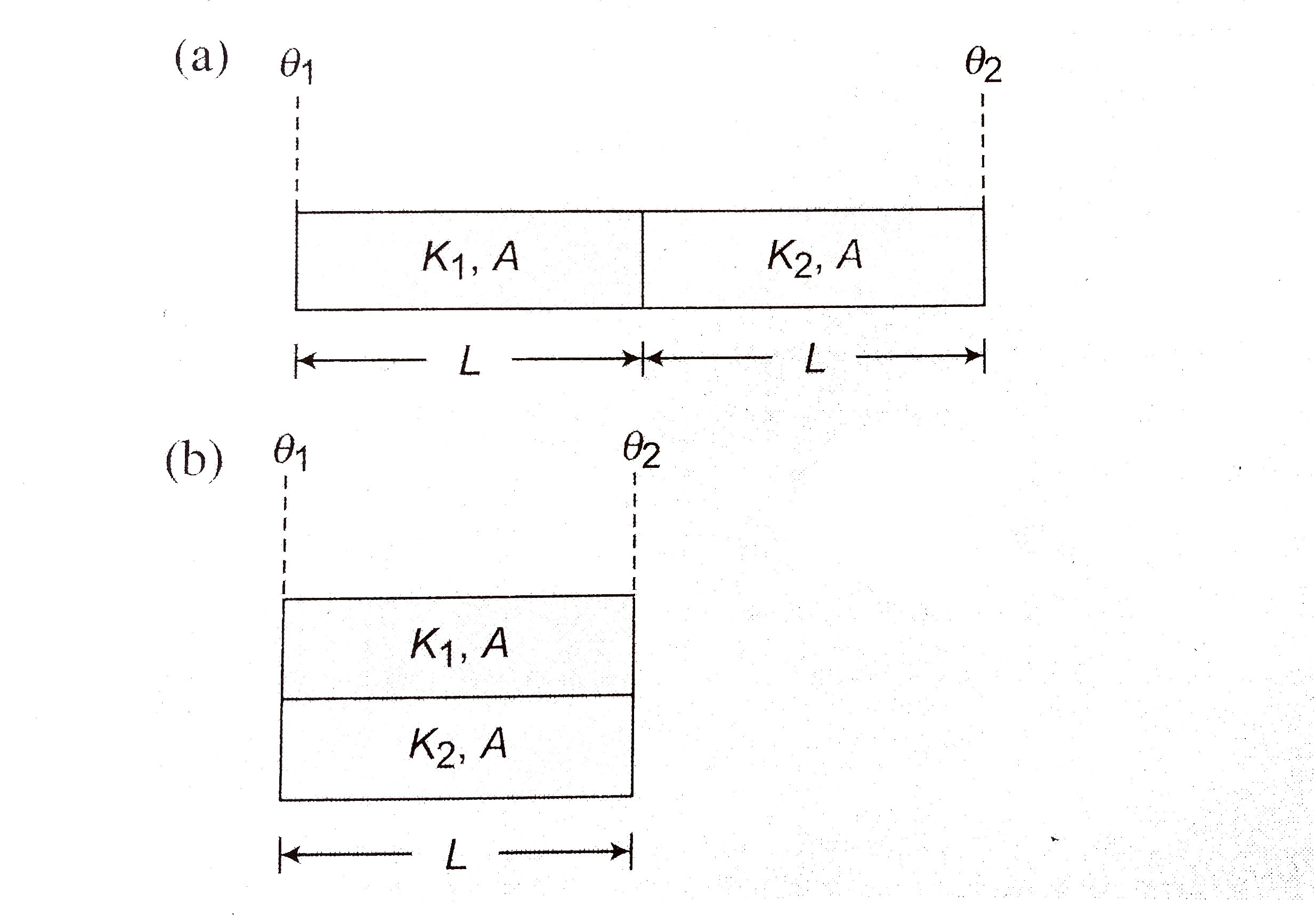
 View Solution
View SolutionWhat is the S.I. Unit of thermal conductivity?
View SolutionFour rods identical in all respect other than their thermal conductivity are joined to form a square loop.The thermal conductivity of rods are shown in the figure.The ratio of temperatures of point A and B (in steady state) is k35 . Find the value of k. Other two ends are maintained at 100∘C and 20∘C
View Solution[" Four rods identical in all respect "],[" other than their thermal "],[" conductivity are joined to form a "],[" square loop.The thermal "],[" conductivity of rods are shown in "],[" the figure.The ratio of "],[" temperatures of point "A" and "B" (in "],[" steady state) is "(k)/(35)." Find the value "],[" of "k" .[Other two ends are "],[" maintained at "100^(@)C" and "20^(@)C]]
View Solution

