Two solid spheres are heated to the same temperature allowed to cool under identical conditions Compare (i) initial rates of fall of temperatre, and (ii) initial rates of loss of heat Assume that all the surfaces have the same emissivity and ratios of their radii specific heats and densities are respectively 1:α1:β,1:γ .
αβγ:1
1:α2
β=αγ
1:α3
The correct Answer is:A, B
To solve the problem, we will analyze the cooling of two solid spheres under identical conditions, focusing on the initial rates of fall of temperature and the initial rates of loss of heat.
Step 1: Understanding the Cooling Process
Both spheres are initially at the same temperature and are cooling down. According to Newton's law of cooling, the rate of change of temperature of an object is proportional to the difference between its temperature and the surrounding temperature.
Step 2: Initial Rate of Fall of Temperature
The initial rate of fall of temperature can be expressed as:
dTdt=−dQms
where dQ is the heat lost, m is the mass of the sphere, and s is the specific heat capacity.
Step 3: Heat Loss by Radiation
The rate of heat loss by radiation can be given by:
dQdt=EσA(T4−T40)
where E is the emissivity, σ is the Stefan-Boltzmann constant, A is the surface area, T is the temperature of the sphere, and T0 is the surrounding temperature.
Step 4: Surface Area and Mass
For spheres A and B:
- Let the radius of sphere A be rA and that of sphere B be rB.
- The surface area A of a sphere is given by A=4πr2.
- The mass m of a sphere can be expressed in terms of its density ρ and volume V:
m=ρV=ρ(43πr3)
Step 5: Comparing the Initial Rates of Fall of Temperature
For sphere A:
dQAdt=Eσ(4πr2A)(T4−T40)
For sphere B:
dQBdt=Eσ(4πr2B)(T4−T40)
Dividing the two:
dTAdtdTBdt=mBsBmAsA⋅ABAA
Substituting the expressions for mass and area:
dTAdtdTBdt=ρB(43πr3B)sBρA(43πr3A)sA⋅4πr2B4πr2A
This simplifies to:
dTAdtdTBdt=ρBr2BsBρAr2AsA
Given the ratios of densities and specific heats as 1:α and 1:β, and the radii as 1:γ, we can express:
dTAdt:dTBdt=βγ2α
Step 6: Initial Rates of Loss of Heat
Using the same heat loss equations:
dQAdt=Eσ(4πr2A)(T4−T40)
dQBdt=Eσ(4πr2B)(T4−T40)
Thus,
dQA/dtdQB/dt=AAAB=r2Ar2B=1α2
Final Results
1. Initial rates of fall of temperature:
dTAdt:dTBdt=βγ2α
2. Initial rates of loss of heat:
dQA/dtdQB/dt=1α2
Both spheres are initially at the same temperature and are cooling down. According to Newton's law of cooling, the rate of change of temperature of an object is proportional to the difference between its temperature and the surrounding temperature.
The initial rate of fall of temperature can be expressed as:
dTdt=−dQms
where dQ is the heat lost, m is the mass of the sphere, and s is the specific heat capacity.
The rate of heat loss by radiation can be given by:
dQdt=EσA(T4−T40)
where E is the emissivity, σ is the Stefan-Boltzmann constant, A is the surface area, T is the temperature of the sphere, and T0 is the surrounding temperature.
For spheres A and B:
- Let the radius of sphere A be rA and that of sphere B be rB.
- The surface area A of a sphere is given by A=4πr2.
- The mass m of a sphere can be expressed in terms of its density ρ and volume V:
m=ρV=ρ(43πr3)
For sphere A:
dQAdt=Eσ(4πr2A)(T4−T40)
For sphere B:
dQBdt=Eσ(4πr2B)(T4−T40)
dTAdtdTBdt=mBsBmAsA⋅ABAA
Substituting the expressions for mass and area:
dTAdtdTBdt=ρB(43πr3B)sBρA(43πr3A)sA⋅4πr2B4πr2A
This simplifies to:
dTAdtdTBdt=ρBr2BsBρAr2AsA
Given the ratios of densities and specific heats as 1:α and 1:β, and the radii as 1:γ, we can express:
dTAdt:dTBdt=βγ2α
Using the same heat loss equations:
dQAdt=Eσ(4πr2A)(T4−T40)
dQBdt=Eσ(4πr2B)(T4−T40)
Thus,
dQA/dtdQB/dt=AAAB=r2Ar2B=1α2
1. Initial rates of fall of temperature:
dTAdt:dTBdt=βγ2α
2. Initial rates of loss of heat:
dQA/dtdQB/dt=1α2
Topper's Solved these Questions
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-I(H.W)
19 Videos
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-II(C.W)
27 Videos
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - SINGLE ANSWER QUESTIONS Passage -1
1 Videos
- THERMODYNAMICSBOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise
187 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - STATEMENT TYPE QUESTION
23 Videos
Similar Questions
A solid copper cube and sphere, both of same mass & emissivity are heated to same initial temperature and kept under identical conditions. What is the ratio of their initial rate of fall of temperature ?
View SolutionTwo spheres of same material and radius r and 2r are heated to same temperature and are kept in identical surroundings, ratio of their rate of loss of heat is
View Solution
Knowledge Check
Two solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
AR1R2BR2R1CR21R22DR22R21Rate of loss of heat of two spheres at same temperature having radii in ratio 1 : 2 is
A1/2B2/1C1/4D4/1A hollow and a solid sphere of same material and identical outer surface under identical condition are heated to the same temperature at the same time (both have same e, a):
A) In the beginning both will emit equal amount of radiation per unit timeBIn the beginning both will absorb equal amount of radiation per unit timeCBoth spheres will have same rate of fall of temperature (dT)/dt)DBoth spheres will have equal temperatures at any moment
A solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperatures. Assume that the emissivity of both the spheres is the same. Find ratio of (a) the rate of heat loss from the aluminium sphere to the rate of all of temperature of the copper sphere. The specific heat capacity of aluminium =900Jkg−1^(@)C^(-1).andtˆofcopper=390Jkg^(-1)^(∘)C−1 . The density of copper =3.4 times the correct wattage.
View SolutionTwo solid spheres of radii 5cm and 3cm are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. The ratio of rate of fall of temperature is
View SolutionA solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperature. Assume that the emissivity of both the spheres is the same. Find the ratio of
(a) the rate of heat from the aluminium sphere to the rate of heat loss from the copper sphere and
(b) the rate of fall of temperature of the the aluminium sphere to the rate of fall temperature of teh copper sphere. The specific heat capacity of aluminium =900J/kg−∘C and that of copper =390J/kg−∘C. The density of copper is 3.4 times the density of aluminium.
View Solution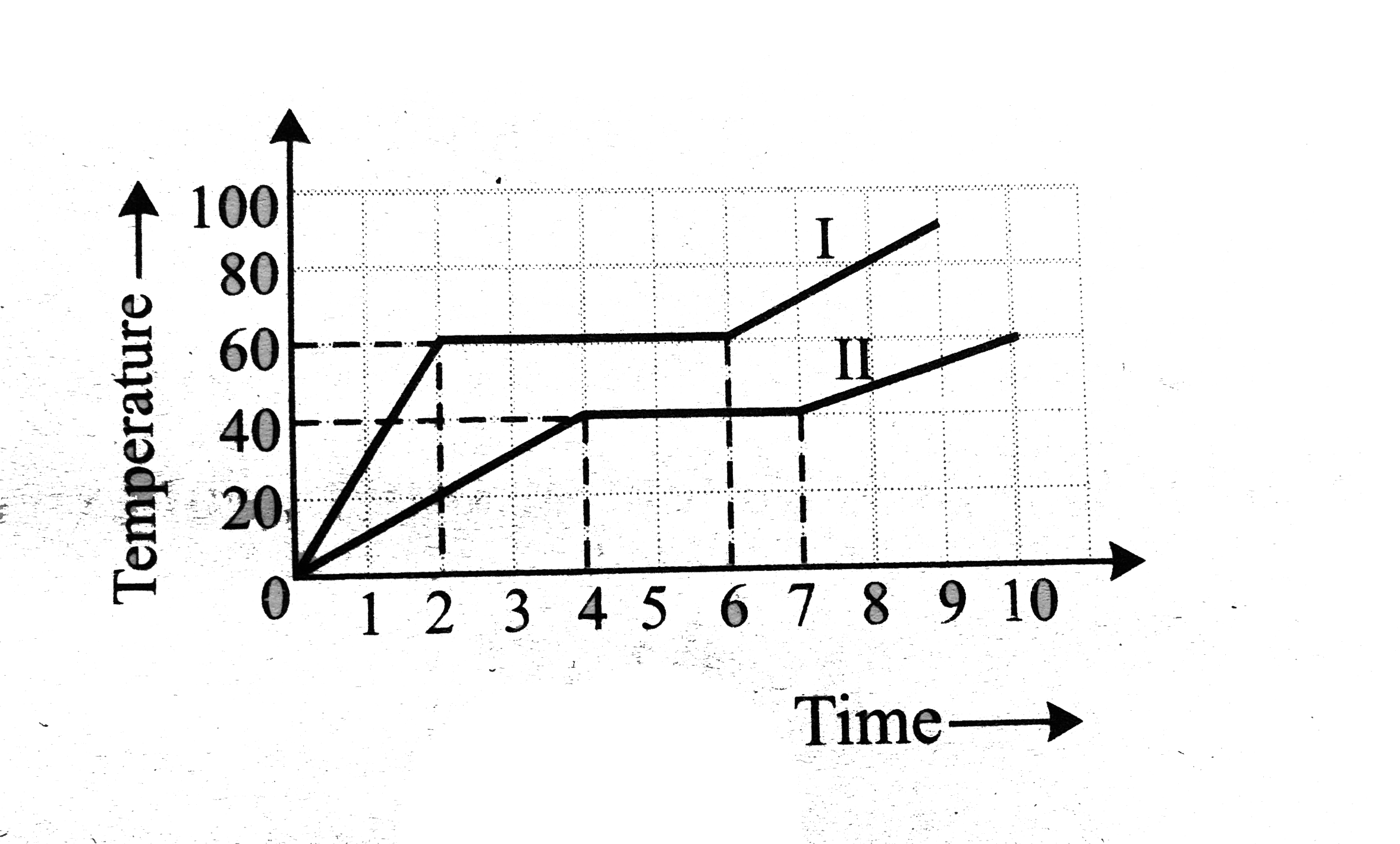
Two bodies of equal masses are heated at a uniform rate under identical conditions. The change in temperature in the two cases in shown graphically. What are their melting points?
Find the ratio of their specific heats and latent heats.
View SolutionTwo solid spheres of radii R1 and R2 are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. Assuming spheres to be perfect conductors of heat, their initial ratio of rates of loss of heat is:
View Solution
