A solid sphere of radius R is floating in a liquid of density σ with half of its volume submerged. If the sphere is slightly pushed and released , it starts axecuting simple harmonic motion. Find the frequency of these oscillations.
To find the frequency of oscillations of a solid sphere floating in a liquid, we will follow these steps:
Step 1: Understand the System
A solid sphere of radius R is floating in a liquid of density σ with half of its volume submerged. The volume of the sphere is given by:
V=43πR3
Since half of the sphere is submerged, the submerged volume Vs is:
Vs=12V=12(43πR3)=23πR3
Step 2: Calculate the Weight of the Sphere
The weight W of the sphere can be expressed as:
W=mg
where m is the mass of the sphere. Since the sphere is floating, the buoyant force Fb acting on it is equal to the weight of the liquid displaced by the submerged volume:
Fb=Vs⋅σ⋅g=(23πR3)σg
At equilibrium, we have:
mg=Fb
Step 3: Analyze the Motion When Pushed Down
When the sphere is pushed down by a small distance x, the new submerged volume V′s becomes:
V′s=Vs+additional submerged volume=23πR3+πR2x
The new buoyant force F′b is:
F′b=V′s⋅σ⋅g=(23πR3+πR2x)σg
Step 4: Calculate the Net Force
The net force Fnet acting on the sphere when it is displaced by x is:
Fnet=F′b−mg=(23πR3+πR2x)σg−mg
Substituting mg=23πR3σg:
Fnet=(23πR3σg+πR2xσg)−23πR3σg
This simplifies to:
Fnet=πR2xσg
Step 5: Relate to Simple Harmonic Motion
For simple harmonic motion, the net force is proportional to the displacement:
Fnet=−kx
where k is the spring constant. Here, we have:
Fnet=−πR2σgx
Thus, we can identify:
k=πR2σg
Step 6: Find the Frequency of Oscillation
The angular frequency ω for simple harmonic motion is given by:
ω=√km
Substituting k and m:
ω=
⎷πR2σg23πR3σ=√3g2R
The frequency f is related to the angular frequency by:
f=ω2π=12π√3g2R
Final Answer
The frequency of oscillations of the sphere is:
f=12π√3g2R
A solid sphere of radius R is floating in a liquid of density σ with half of its volume submerged. The volume of the sphere is given by:
V=43πR3
Since half of the sphere is submerged, the submerged volume Vs is:
Vs=12V=12(43πR3)=23πR3
The weight W of the sphere can be expressed as:
W=mg
where m is the mass of the sphere. Since the sphere is floating, the buoyant force Fb acting on it is equal to the weight of the liquid displaced by the submerged volume:
Fb=Vs⋅σ⋅g=(23πR3)σg
At equilibrium, we have:
mg=Fb
When the sphere is pushed down by a small distance x, the new submerged volume V′s becomes:
V′s=Vs+additional submerged volume=23πR3+πR2x
The new buoyant force F′b is:
F′b=V′s⋅σ⋅g=(23πR3+πR2x)σg
The net force Fnet acting on the sphere when it is displaced by x is:
Fnet=F′b−mg=(23πR3+πR2x)σg−mg
Substituting mg=23πR3σg:
Fnet=(23πR3σg+πR2xσg)−23πR3σg
This simplifies to:
Fnet=πR2xσg
For simple harmonic motion, the net force is proportional to the displacement:
Fnet=−kx
where k is the spring constant. Here, we have:
Fnet=−πR2σgx
Thus, we can identify:
k=πR2σg
The angular frequency ω for simple harmonic motion is given by:
ω=√km
Substituting k and m:
ω=
⎷πR2σg23πR3σ=√3g2R
The frequency f is related to the angular frequency by:
f=ω2π=12π√3g2R
The frequency of oscillations of the sphere is:
f=12π√3g2R
Topper's Solved these Questions
Similar Questions
A solid sphere of mass M and Radius R is attached to a massless spring of force constant k as shown in the figure. The cylinder is pulled towards right slightly and released so that it executes simple harmonic motion of time period. If the cylinder rolls on the horizontal surface without slipping, calculate its time period of oscillation.
 View Solution
View SolutionA cylindrical plastic bottle of negligible mass is filled with 310 ml of water and left floating in a pond with still water. If pressed downward slightly and released, it starts performing simple harmonic montion angular frequency ω. If the radius of the bottle is 2.5 cm then ω is close to: (density of water =103kg/m3)
View Solution
Knowledge Check
- Question 1 - Select One or More
A solid sphere of density σ with one -third of tis volume submerged . When the sphere is pressed down slightly and released , it executes S.H.M of time period T. Then (viscous effect is ignored)
Ap=σ3Bp=3σC4π3√RgD3π2√Rg - Question 2 - Select One
A pendulum of mass m and length L is connected to a spring as shown in figure. If the bob is displaced slightly from its mean position and released, it performs simple harmonic motion. The angular frequency of the oscillation of bob is
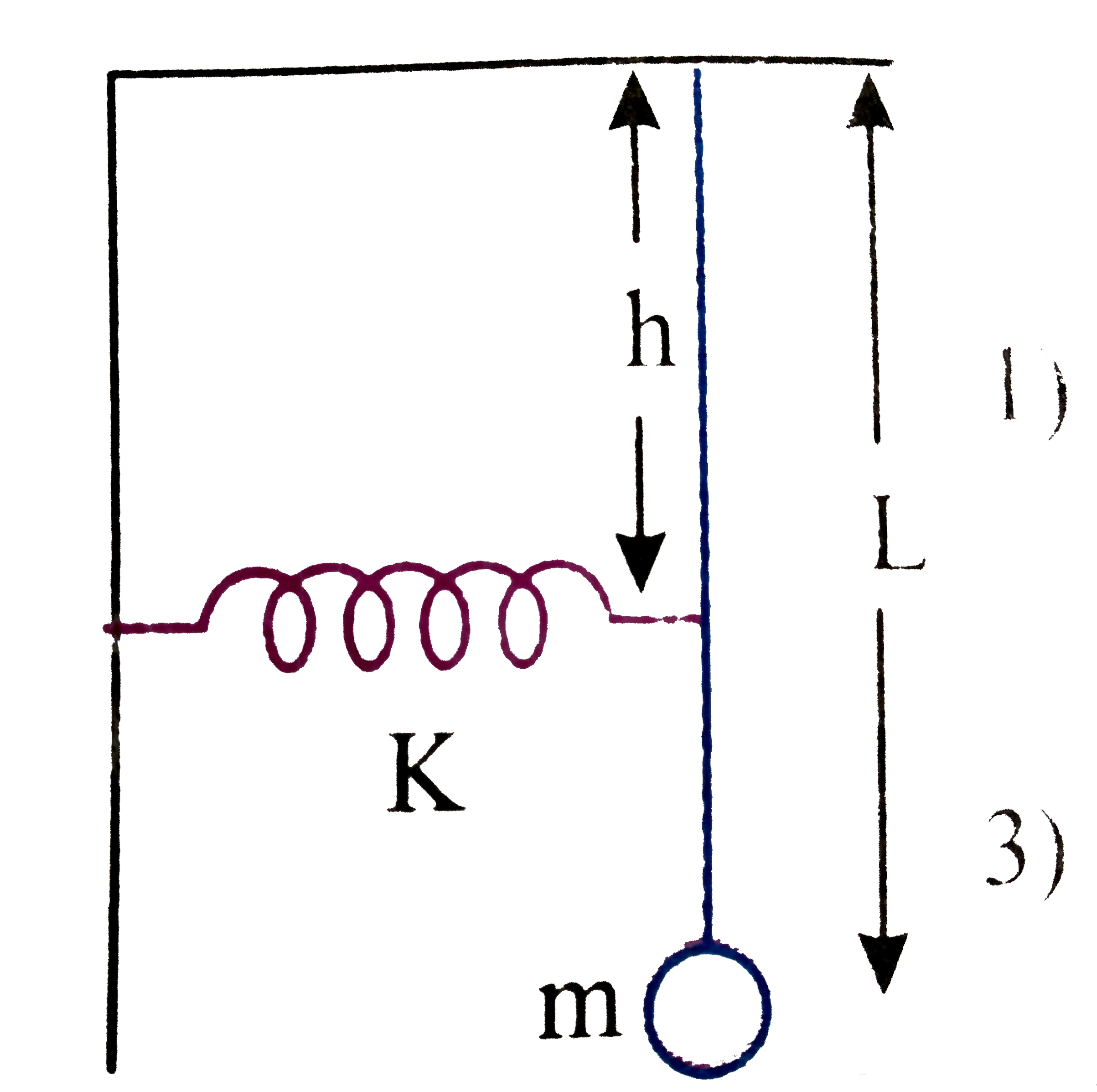 A√gLB√Kh2+mgLmL2C√3gLD√Kh2mL2
A√gLB√Kh2+mgLmL2C√3gLD√Kh2mL2 - Question 3 - Select One
A uniform solid bar of wood floats vertically in water with 10 cm of its height immersed in the water. When it is slightly depressed and released, it oscillates with a frequency of
Aπ7HzB√2π7HzC7πHzD7√2πHz
A wooden cube (density of wood ρ3 ') of side l 'floats in a liquid of density ' ρ with its upper and lower surfaces horizontal. If the cube is pushed slightly down and released, it performs simple harmonic motion of period T. Then, T is equal to 2π√xlyg. Find (x+y).
View SolutionA wooden cube (density of wood 'd') of side 'l' flotes in a liquid of density 'ρ' with its upper and lower surfaces horizonta. If the cube is pushed slightly down and released, it performs simple harmonic motion of period 'T'. Then, 'T' is equal to :-
View SolutionA wooden cylinder of length L is partly submerged in a liquid of specific gravity ρ1 with nth (n<1) part of it inside the liquid. Another immiscible liquid of density ρ2 is poured to completely submerge the cylinder. Density of cylinder ρ is the geometric mean of the densities of the two liquid.
When the cylinder is slightly depressed and released, it oscillates. let there be a mean position. find the time period of small oscillations below thee mean positionView SolutionA solid cube of side a and density ρ0 floats on the surface of a liquid of density ρ. If the cube is slightly pushed downward, then it oscillates simple harmonically with a period of
View SolutionA sphere of radius R and density ρ1 is dropped in a liquid of density σ. Its terminal velocity is v1. If another sphere of radius R and density ρ2 is dropped in the same liquid, its terminal velocity will be:
View Solution

