The pressure of a gas changes linearly with volume from 10 kPa, 200 cc to 50 kPa, 50 cc.
(a) Calculate the work done by the gas.
(b) If no heat is supplied or extracted from the gas, what is the changes in the internal energy of the gas?
To solve the problem step by step, we will first calculate the work done by the gas and then determine the change in internal energy.
1. Identify the pressure and volume points:
- Initial pressure (P1) = 10 kPa at volume (V1) = 200 cc
- Final pressure (P2) = 50 kPa at volume (V2) = 50 cc
2. Convert units:
- Convert the pressures from kPa to Pa (1 kPa = 1000 Pa):
- P1 = 10 kPa = 10,000 Pa
- P2 = 50 kPa = 50,000 Pa
- Convert volumes from cc to m³ (1 cc = 1 x 10^-6 m³):
- V1 = 200 cc = 200 x 10^-6 m³ = 0.0002 m³
- V2 = 50 cc = 50 x 10^-6 m³ = 0.00005 m³
3. Draw the pressure-volume (P-V) graph:
- The graph will be a straight line connecting the points (V1, P1) and (V2, P2).
4. Calculate the area under the curve (work done):
- The work done (W) by the gas during the expansion/compression can be calculated as the area under the P-V curve.
- The shape formed is a trapezium.
- The formula for the area of a trapezium is:
W=12×(P1+P2)×(V2−V1)
- Plugging in the values:
W=12×(10,000+50,000)×(0.00005−0.0002)
W=12×60,000×(−0.00015)
W=−4.5 Joules
(b) Calculate the change in internal energy of the gas.
1. Use the first law of thermodynamics:
- The first law states:
ΔQ=ΔU+ΔW
- Given that no heat is supplied or extracted:
ΔQ=0
- Therefore:
0=ΔU+(−4.5)
- Rearranging gives:
ΔU=4.5 Joules
Final Answers:
- (a) The work done by the gas is -4.5 Joules.
- (b) The change in internal energy of the gas is 4.5 Joules.
- Initial pressure (P1) = 10 kPa at volume (V1) = 200 cc
- Final pressure (P2) = 50 kPa at volume (V2) = 50 cc
- Convert the pressures from kPa to Pa (1 kPa = 1000 Pa):
- P1 = 10 kPa = 10,000 Pa
- P2 = 50 kPa = 50,000 Pa
- Convert volumes from cc to m³ (1 cc = 1 x 10^-6 m³):
- V1 = 200 cc = 200 x 10^-6 m³ = 0.0002 m³
- V2 = 50 cc = 50 x 10^-6 m³ = 0.00005 m³
- The graph will be a straight line connecting the points (V1, P1) and (V2, P2).
- The work done (W) by the gas during the expansion/compression can be calculated as the area under the P-V curve.
- The shape formed is a trapezium.
- The formula for the area of a trapezium is:
W=12×(P1+P2)×(V2−V1)
- Plugging in the values:
W=12×(10,000+50,000)×(0.00005−0.0002)
W=12×60,000×(−0.00015)
W=−4.5 Joules
- The first law states:
ΔQ=ΔU+ΔW
- Given that no heat is supplied or extracted:
ΔQ=0
- Therefore:
0=ΔU+(−4.5)
- Rearranging gives:
ΔU=4.5 Joules
- (a) The work done by the gas is -4.5 Joules.
- (b) The change in internal energy of the gas is 4.5 Joules.
Topper's Solved these Questions
Similar Questions
the pressure of a gas change linearly with volume from 10kPa, 200 cc to 50 kPa, 50 cc. (a) calculate the work done by the gas, (b) what is the change in the internal energy of the gas ?
View Solution400 J of work is done on a gas to reduce its volume by compression adiabatically. What is the change in internal energy of the gas?
View Solution
Knowledge Check
- Question 1 - Select One
In an adiabatic process 90 J of work is done on the gas. The change in internal energy of the gas is
A−90jB+90jC0 jDDepends on initial temperature - Question 2 - Select One
A gas receives an amount of heat equal to 110 J and performs 40 J of work. The change in the internal energy of the gas is
A70 JB150 JC110 JD40 J - Question 3 - Select One
If 21 J of heat energy is supplied to an ideal diatomic gas at constant pressure, then the change in its energy is
A10 JB12 JC15 JD18 J
Method 2 of ΔU
Work done by a gas in a given process is −20J. Heat given to the gas is 60J. Find change in internal energy of the gas.View SolutionA gas is taken along the path AB as shown in , if 70 cal of heat is extracted from the gas in the process, calculate the change in the internal energy of the system.
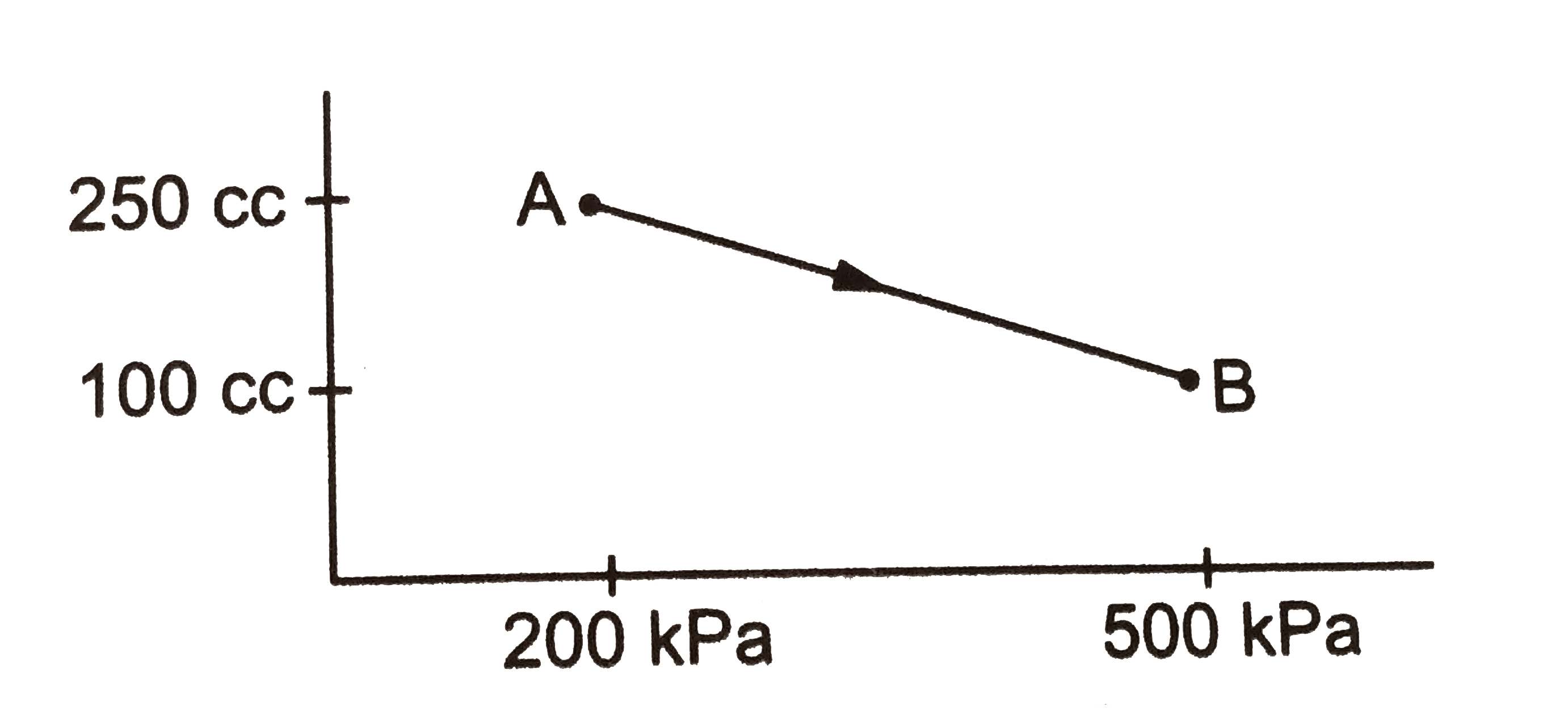 View Solution
View SolutionA sample of ideal gas (γ=1.4) is heated at constant pressure. If an amount of 140 J heat is supplied to the gas, then change in internal energy of the gas
View SolutionIn a thermodynamics process, 300 joule of heat is supplied to a gas and 200 joule of work is done by the gas. What is the change in internal energy of the system?
View SolutionThe volume of a monatomic ideal gas increases linearly with pressure, as shown in Fig. Calculate (a) increase in internal energy, (b) work done by the gas and (c ) heat supplied to the gas.
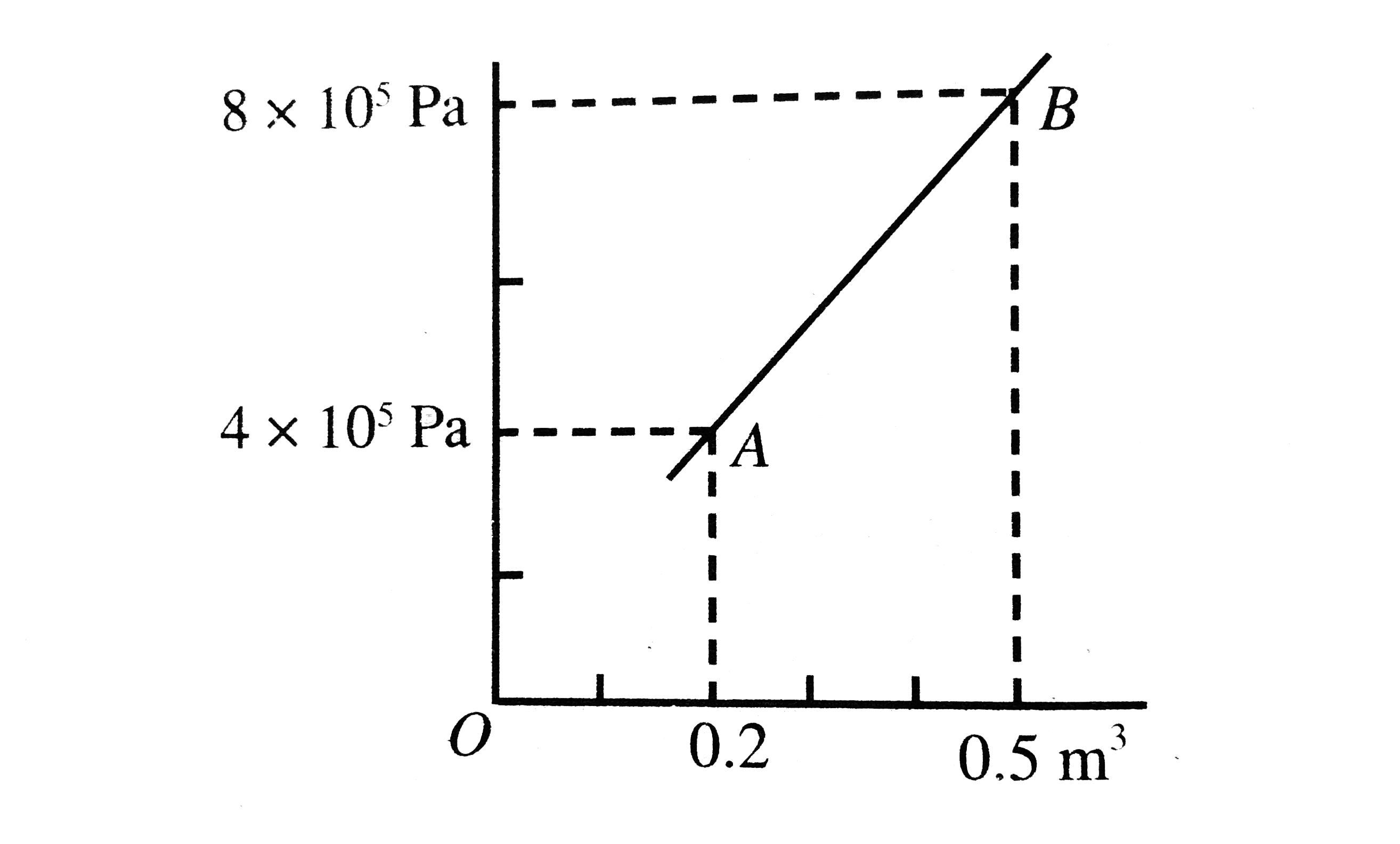 View Solution
View Solution

