The fraction of the volume of a glass flask must be filled with mercury so that the volume of the empty space may be the same at all temperature is
(αglass=9×10−6/0C,γHg=18.9×10−5/0C)
12
17
14
15
The correct Answer is:B
To solve the problem, we need to determine the fraction of the volume of a glass flask that must be filled with mercury so that the volume of the empty space remains constant at all temperatures. We are given the coefficients of linear expansion for glass and volumetric expansion for mercury.
1. Understand the Coefficients of Expansion:
- The coefficient of linear expansion of glass is given as αglass=9×10−6∘C−1.
- The volumetric expansion coefficient of mercury is given as γHg=18.9×10−5∘C−1.
2. Relate the Volumes and Expansions:
- Let Vg be the volume of glass and Vm be the volume of mercury.
- The change in volume due to temperature change ΔT can be expressed as:
ΔVg=Vg⋅3αglass⋅ΔT
ΔVm=Vm⋅γHg⋅ΔT
- Since we want the empty space to remain constant, we need the changes in volumes to be equal:
ΔVg=ΔVm
3. Set Up the Equation:
- From the above relationships, we can set up the equation:
Vg⋅3αglass=Vm⋅γHg
4. Substitute the Values:
- Substituting the values of αglass and γHg:
Vg⋅3⋅(9×10−6)=Vm⋅(18.9×10−5)
- Simplifying this gives:
Vg⋅27×10−6=Vm⋅18.9×10−5
5. Express the Volumes in Terms of Each Other:
- Rearranging the equation to find the ratio of the volumes:
VmVg=27×10−618.9×10−5
- Simplifying the right side:
VmVg=27189=17
6. Conclusion:
- The fraction of the volume of the glass flask that must be filled with mercury is 17.
Final Answer:
The fraction of the volume of the glass flask that must be filled with mercury is 17.
- The coefficient of linear expansion of glass is given as αglass=9×10−6∘C−1.
- The volumetric expansion coefficient of mercury is given as γHg=18.9×10−5∘C−1.
- Let Vg be the volume of glass and Vm be the volume of mercury.
- The change in volume due to temperature change ΔT can be expressed as:
ΔVg=Vg⋅3αglass⋅ΔT
ΔVm=Vm⋅γHg⋅ΔT
- Since we want the empty space to remain constant, we need the changes in volumes to be equal:
ΔVg=ΔVm
- From the above relationships, we can set up the equation:
Vg⋅3αglass=Vm⋅γHg
- Substituting the values of αglass and γHg:
Vg⋅3⋅(9×10−6)=Vm⋅(18.9×10−5)
- Simplifying this gives:
Vg⋅27×10−6=Vm⋅18.9×10−5
- Rearranging the equation to find the ratio of the volumes:
VmVg=27×10−618.9×10−5
- Simplifying the right side:
VmVg=27189=17
- The fraction of the volume of the glass flask that must be filled with mercury is 17.
The fraction of the volume of the glass flask that must be filled with mercury is 17.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)19videos
THERMAL PROPERTIES OF MATTER
- BOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - VI4videos
THERMAL PROPERTIES OF MATTER
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV39videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise187videos
THERMODYNAMICS
Similar Questions
A glass beaker holds exactly 1Lat0∘C
(a) What is its volume at 50∘C ?
(b) If the beaker is filled with mercury at 0∘C, what volume of mercury overflows ehen the temperature is 50∘C ? αg=8.3×10−6per∘C andγHg=1.82×10−4per∘C.View SolutionA bakelite beacker has volume capacity of 500ccat30∘C. When it is partially filled with Vm volumne (at 30∘C) of mercury, it is found that the unfilled volume of the beaker remains constant as temperature is varied. If γbeaker=6×10−6.∘C−1 and γmercury=1.5×10−4.∘C−1 , where γ is the coefficient of volume expansion , then Vm (in cc) is close to __________.
View Solution
Knowledge Check
- Question 1 - Select One
A one litre flask contains some mercury. It is found that at different temperatures the volume of air inside the flask remains the same. The volume of mercury in the flask is (αglass=9×10−6/∘C,γHg=180×10−6/∘C)
A150cm3B225cm3C300cm3D450cm3 - Question 2 - Select One
A flask is filled with mercury at temperature 28∘C . If the flask and the contents are heated to 48∘C , the volume of mercury above the mark will be (αglass=9×10−6/∘C , γHg=180×10−6/∘C)
A0.15cm3B0.25cm3C0.3cm3D0.5cm3 - Question 3 - Select One
A two litre glass flask contains some mercury. It is found that at all temperatures the volume of the air inside the flask remains the same. The volume of the mercury inside the flask is (αfor glass =9×10−6/∘C,γ for mercury 1.8×10−4/∘C)
A1500 ccB150 ccC3000 ccD300 cc
In design of a compensated pendulum, a light metal rod of length L0 = 1.0 m is attached to a glass tube filled with mercury. Neglecting the mass of the glass tube as well, calculate the height of mercury column in the glass tube so that centre of mass of this system does not rise or fall with temperature Given : λ)Hg)=1.82×10−4K−1,αglass')=9xx10^(-6),alpha_(mηl=1.2×10−5K−1
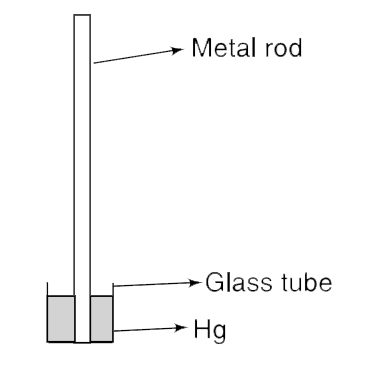 View Solution
View Solutiona glass tube of length 133cm and of uniform cross-section is to be filled with mercury so that the volume of the unoccupied by mercury remains the same at all temperatures. If cubical coefficient for glass and mercury are respectively 0.000026/∘C and 0.000182/∘C , calculate the length of mercury column.
View SolutionA glass tube is attached to the bottom of a thin iron rod of length 1m. To what height must the glass tube be filled with mercury so that the centre of mass of the pendulum formed will not rise or all with changes in temperature?
(Linear expansivity of glass =9×10−6K−1, that of iron =12x10−6K−1 and cubical edxpansivity of mercury =18.2×10−5K−1)View SolutionA glass tube of length l0 and of uniform cross-section is to be filled with mercury so that the length of the tube unoccoupied by mercury remains same at all temperatures. If γg=3α , calculate length of mercury column.
View SolutionA glass flask of volume 200cm3 is just filled with mercury at 20∘C. The amount of mercury that will overflow when the temperature of the system is raised to 100∘C is (γglass=1.2×10−5/C∘,γmercury=1.8×10−4/C∘)
View Solution

