Three forces →F1,→F2 and →F3 are simultaneously acting on a particle of mass 'm' and keep it in equlibrium. If →F1 force is reversed in direction only, the acceleration of the particle will be.
¯¯¯F1/m
2¯¯¯F1/m
−¯¯¯F1/m
−2¯¯¯F1/m
The correct Answer is:D
To solve the problem step by step, we will analyze the forces acting on the particle and how reversing the direction of one of the forces affects the net force and consequently the acceleration of the particle.
Step 1: Understand the Initial Condition
Initially, the particle is in equilibrium under the action of three forces: →F1, →F2, and →F3. In equilibrium, the net force acting on the particle is zero.
Equation:
→F1+→F2+→F3=0
Step 2: Rearranging the Forces
From the equilibrium condition, we can express one of the forces in terms of the others. Rearranging the equation gives us:
→F1=−(→F2+→F3)
Step 3: Reverse the Direction of →F1
Now, we reverse the direction of →F1. The new force can be represented as −→F1. Therefore, the new net force acting on the particle becomes:
−→F1+→F2+→F3
Step 4: Substitute for →F1
Substituting →F1 from our earlier equation into the new net force equation gives:
−(−(→F2+→F3))+→F2+→F3
This simplifies to:
→F2+→F3+→F2+→F3=2→F2+2→F3
Step 5: Calculate the Net Force
The net force acting on the particle after reversing →F1 is:
→Fnet=2(→F2+→F3)
Step 6: Apply Newton's Second Law
According to Newton's second law, the acceleration →a of the particle is given by:
→a=→Fnetm
Substituting the expression for net force:
→a=2(→F2+→F3)m
Final Result
Thus, the acceleration of the particle when the direction of →F1 is reversed is:
→a=2(→F2+→F3)m
Initially, the particle is in equilibrium under the action of three forces: →F1, →F2, and →F3. In equilibrium, the net force acting on the particle is zero.
→F1+→F2+→F3=0
From the equilibrium condition, we can express one of the forces in terms of the others. Rearranging the equation gives us:
Now, we reverse the direction of →F1. The new force can be represented as −→F1. Therefore, the new net force acting on the particle becomes:
Substituting →F1 from our earlier equation into the new net force equation gives:
The net force acting on the particle after reversing →F1 is:
According to Newton's second law, the acceleration →a of the particle is given by:
Thus, the acceleration of the particle when the direction of →F1 is reversed is:
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - CIRCULAR MOTIONEXERCISE - LEVEL II(H.W)51videos
CIRCULAR MOTION
- BOOK - NARAYNACHAPTER - CIRCULAR MOTIONEXERCISE - IIT QUES.4videos
CIRCULAR MOTION
- BOOK - NARAYNACHAPTER - CALORIMETRYEXERCISE - Level- II (H.W)11videos
CALORIMETRY
- BOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Level-II (H.W)54videos
COLLISION
Similar Questions
If the four forces as shown are in equilibrium Express →F1&→F2 in unit vector form.
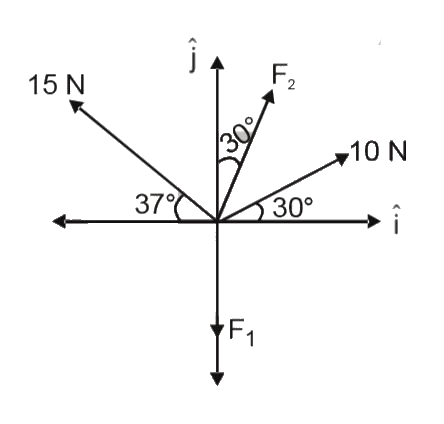 View Solution
View SolutionIf three forces −→F1,+−→F2and−→F3 are represented by three sides of a triangle and −→F1+−→F2=−−→F3 , then these three forces are concurrent forces and satisfy the condition for equilibrium .
Statement II : A triangle made up of three forces →F1,→F2and→F3 as sides taken in the same order ,satisfy the condition for translatory equilibrium . In the light of the above statements ,choose the most appropriate answer from the options given below :View Solution
Knowledge Check
- Question 1 - Select One
Three forces ¯¯¯¯F1,¯¯¯¯F2and¯¯¯¯F3 are simultaneously acting on a particle of mass 'm' and keep it in equilibrium. If ¯¯¯¯F1 force is reversed in direction only, the acceleration of the particle will be.
A¯¯¯¯F1/mB2¯¯¯¯F1/mC−¯¯¯¯F1/mD−2¯¯¯¯F1/m - Question 2 - Select One
Five forces →F1,→F2,→F3,→F4 , and →F5 , are acting on a particle of mass 2.0kg so that is moving with 4m/s2 in east direction. If →F1 force is removed, then the acceleration becomes 7m/s2 in north, then the acceleration of the block if only →F1 is action will be:
A16ms−2B√65ms−2C√260ms−2D√33ms−2 - Question 3 - Select One
Three forces →F1=a(ˆi−ˆj+ˆk),→F2=2ˆi−3ˆj+4ˆk and →F3=8ˆi−7ˆj+6ˆk act simultaneously on a particle. If the particle is in equilibrium, the value of α is
A10B−10C8D2
If the four forces as shown are in equilibrium Express →F1&→F2 in unit vector form.
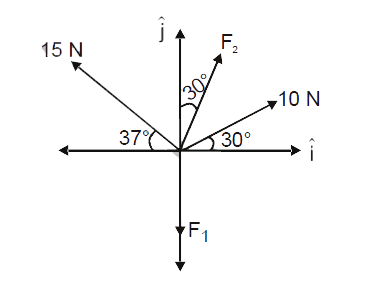 View Solution
View SolutionA force →F=(2xˆi+3ˆj+3z2ˆk) is acting on the particle of mass 2kg which is displaces from point A(2,3,0) to point B(3,2,1). the work done by the force on the particle will be
View SolutionThree forces →F1=(3ˆi+2ˆj−ˆk) dynes, →F2=(3ˆi+4ˆj−5ˆk) dynes and →F3=a(ˆi+ˆj−ˆk) dynes act simultaneously on a particle. What should be the value of 'a' so that the particle may remain in equilibrium?
View SolutionTwo forces →F1and→F2 are acting at right angles to each other, find their resultant ?
View SolutionThe resultant of the forces →F1=4ˆi−3ˆj and →F2=6ˆi+8ˆj is
View Solution

