A particle (A) of mass m1 elastically collides with another stationary particle (B) of mass m2. Then :
m1m2=12 and the particles fly a part in the opposite direction with equal velocities.
m1m2=13 and the particles fly apart in the opposite direction with equal velocities.
m1m2=21 and the collision angle between the particles is 60∘ symmetrically.
m1m2=21 and the particles fly apart symmetrically at an angle 90∘
The correct Answer is:B, C
m1u+0=−m1v+m2v ……….(1)
From kinetic energy conservation
12m1u2=12m1v2+12m2v2 ………..(2)
From eq. (1) and and eq. (2) we get , m1m2=13
So, choice (b) is correct.
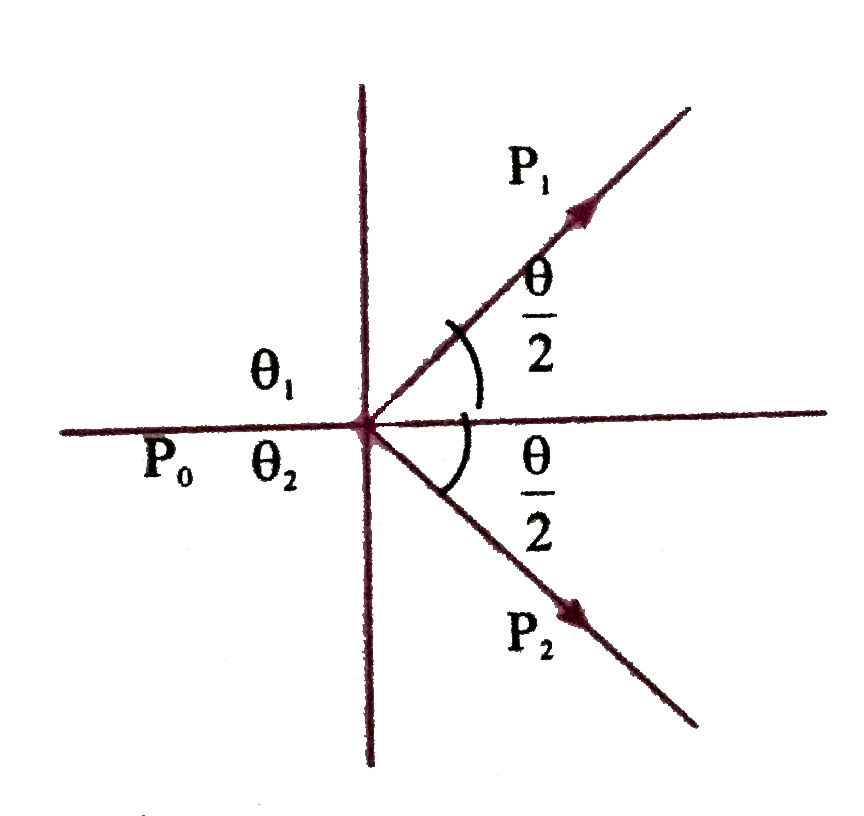
P2=P21+P22+2P1P2cosθ .........(1)
Also from conservation of kinetic energy
P22m1=P212m1+P222m2 ............(2)
Since particles fly symmetrically
P1sin(theta)/(2) = P_(2) sin θ2 ..........(3)
Solving eq. (1), (2) and (3) we get
m1m2=21
Topper's Solved these Questions
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Comprehension type
35 Videos
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Subjective type Questions
9 Videos
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - INTEGER TYPE QUESTIONS
8 Videos
- CIRCULAR MOTIONBOOK - NARAYNACHAPTER - CIRCULAR MOTIONEXERCISE - LEVEL II(H.W)
51 Videos
- FRICTIONBOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I
6 Videos
Similar Questions
A particle of mass m1 moves with speed v and collides head on with a stationary particles of mass m2 . The first particle continues to move in the same direction
If m1m2 is (e = coefficient of restitution )
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entires in column I to all the entires in column II.
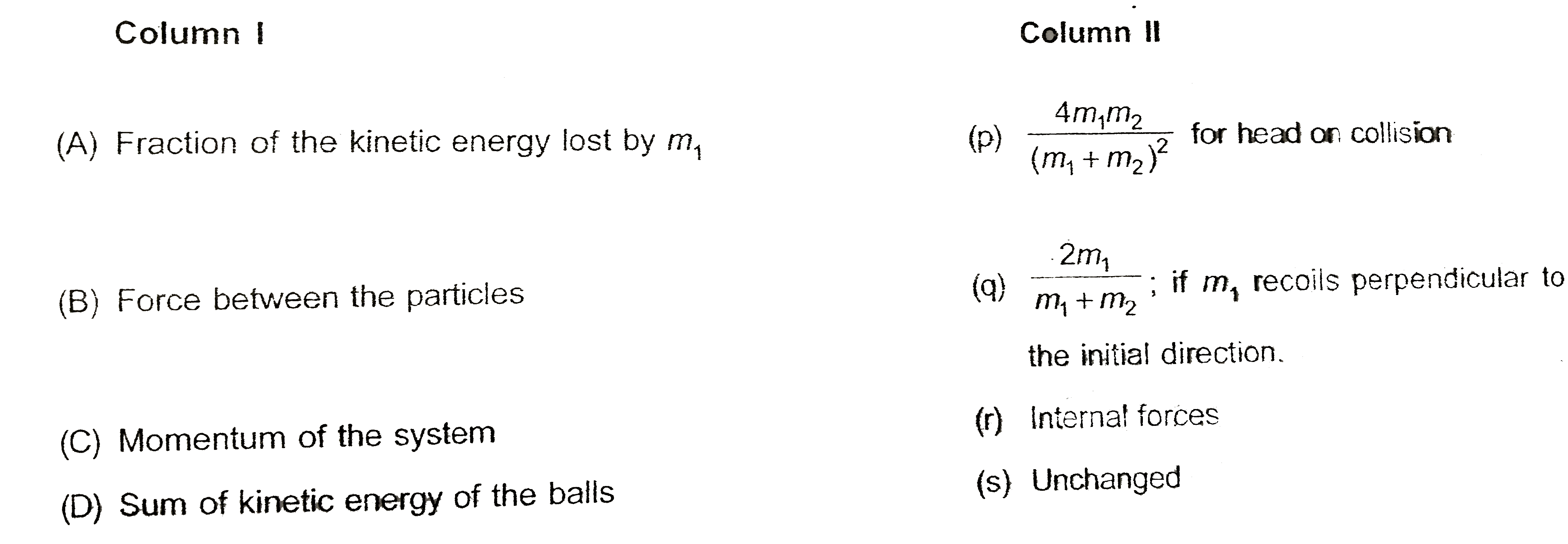
View Solution
Knowledge Check
A particle of mass m1 makes an elastic, one-dimensional collision with a stationary particle of mass m2.What fraction of the kinetic energy of m1 is carried away by m2?
Am1m2Bm2m1C2m1m2(m1+m2)2D4m1m2(m1+m2)2A particle of mass m1 makes an elastic one dimensional collision with a stationary particle of mass m2.What fraction of the kinetic energy of m1 is carried away by m2?
Am1m2Bm2m1C2m1m2(m1+m2)2D4m1m2(m1+m2)2A ball of mass m1 makes an elastic , one-dimensional collision with a stationary particle of mass m2. The fraction of the kinetic energy of m1 transferred to m2 is
A2m1m2(m1+m2)2B4m1m2(m1+m2)2Cm1m2(m1+m2)2D12m12m2(m1+m2)2
A particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
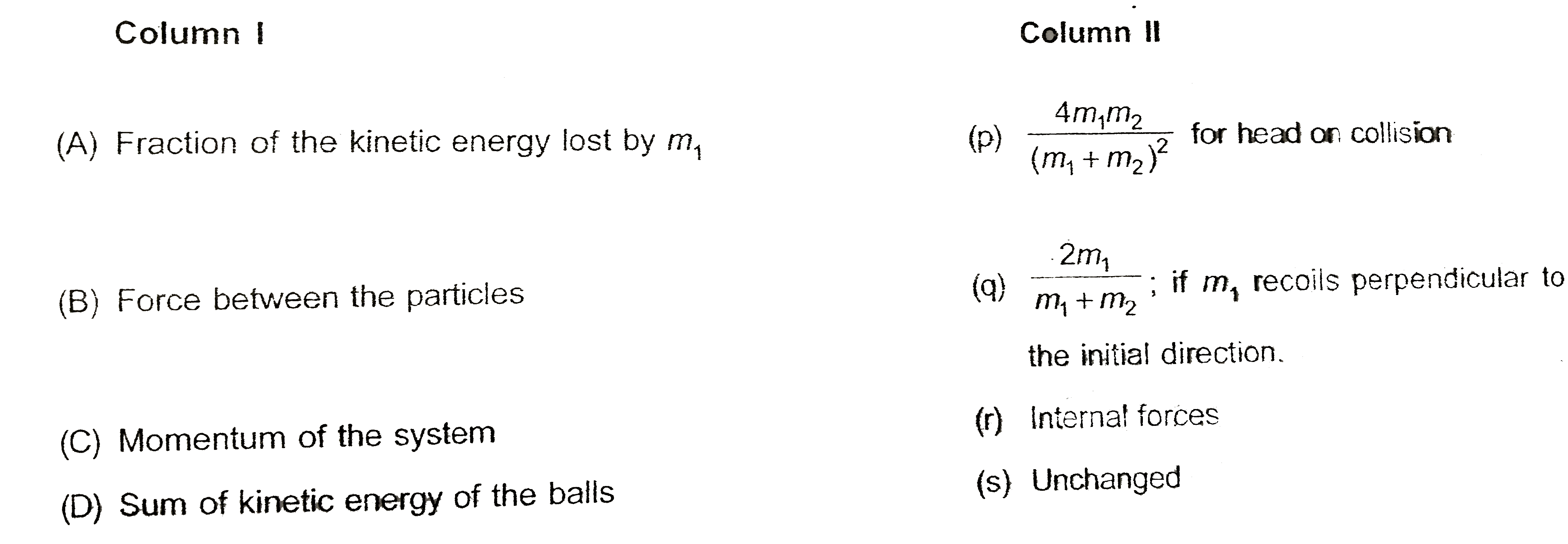
View SolutionA particle of mass m1 collides elastically with a stationary particle of mass m2(m1>m2). Find the maximum angle through which the striking particle may deviate as a result of the collision.
View SolutionA particle of mass m1 makes a head on elastic collision with another stationary body of mass m2, If m1 rebounds with half of its original speed then (m1m2) is
View SolutionA particle of mass m collides with another stationary particle of mass M such that the second particle starts moving and the first particle stops just after the collision. Then which of the following conditions must always be valid ?
View SolutionA particle of mass m1 collides head on with a stationary particles of mass m2. If m1m2 gte, where e is the coefficient of restitution, then :
View Solution
