Consider a ray of light incident from air onto a slab of glass (refr active index n) of width d, at an angle θ . The phase difference between the ray reflected by the top surface of the glass and the bottom surface is
4πdλ(1−1n2sin2θ)1/2+π
4πdλ(1−1n2sin2θ)1/2
4πdλ(1−1n2sin2θ)1/2+π2
4πdλ(1−1n2sin2θ)1/2+2π
The correct Answer is:A

" Consider diagram, the ray " ()P " is incident at an angle " θ " and gets reflected in the direction P' and reflected in the direction P . Due to reflection from the glass medium, there is a phase change of " π. " Time taken to travel along OP "
Δt=OP v=d/ cos r c//n =ndc cos r
From Snell's, law n=sinθsinr
Phase difference,
Δϕ=2πT×Δt⇒Δϕ=2πndλ(1−sin2θn2)−1/2
So, net phase difference =Δϕ+π
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - V37videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - VI40videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - III30videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - SEMICONDUCTOR ELECTRONICSEXERCISE - ADDITIONAL EXERCISE (ASSERTION AND REASON TYPE QUESTIONS :)19videos
SEMICONDUCTOR ELECTRONICS
Similar Questions
A ray of light incident from air on a glass plate of refractive index n is partly reflected and partly refracted at the two surfaces of the glass. The displacement y0 in the figure is
 View Solution
View SolutionA ray of light is incident on the surface of a glass plate of refractive index 1.6 at polarzing angle. Calculate the angles of reflection and refraction.
View Solution
Knowledge Check
- Question 1 - Select One
A ray of light is incident on the surface of plate of glass of refractive index 1.5 at the polarising angle. The angle of refraction of the ray will be
A33.7∘B23.7∘C43.7∘D53.7∘ - Question 2 - Select One
A ray of light is incident on a glass slab of refractive index 1.5, making an angle of 40∘ with the surface. The angle of refraction in glass is
A40∘B30∘42'C50∘D60∘ - Question 3 - Select One
A ray of light incident from air on a glass plate of refractive index n is partly reflected and partly refracted at the two surfaces of the glass. The displacement y0 in the figure is
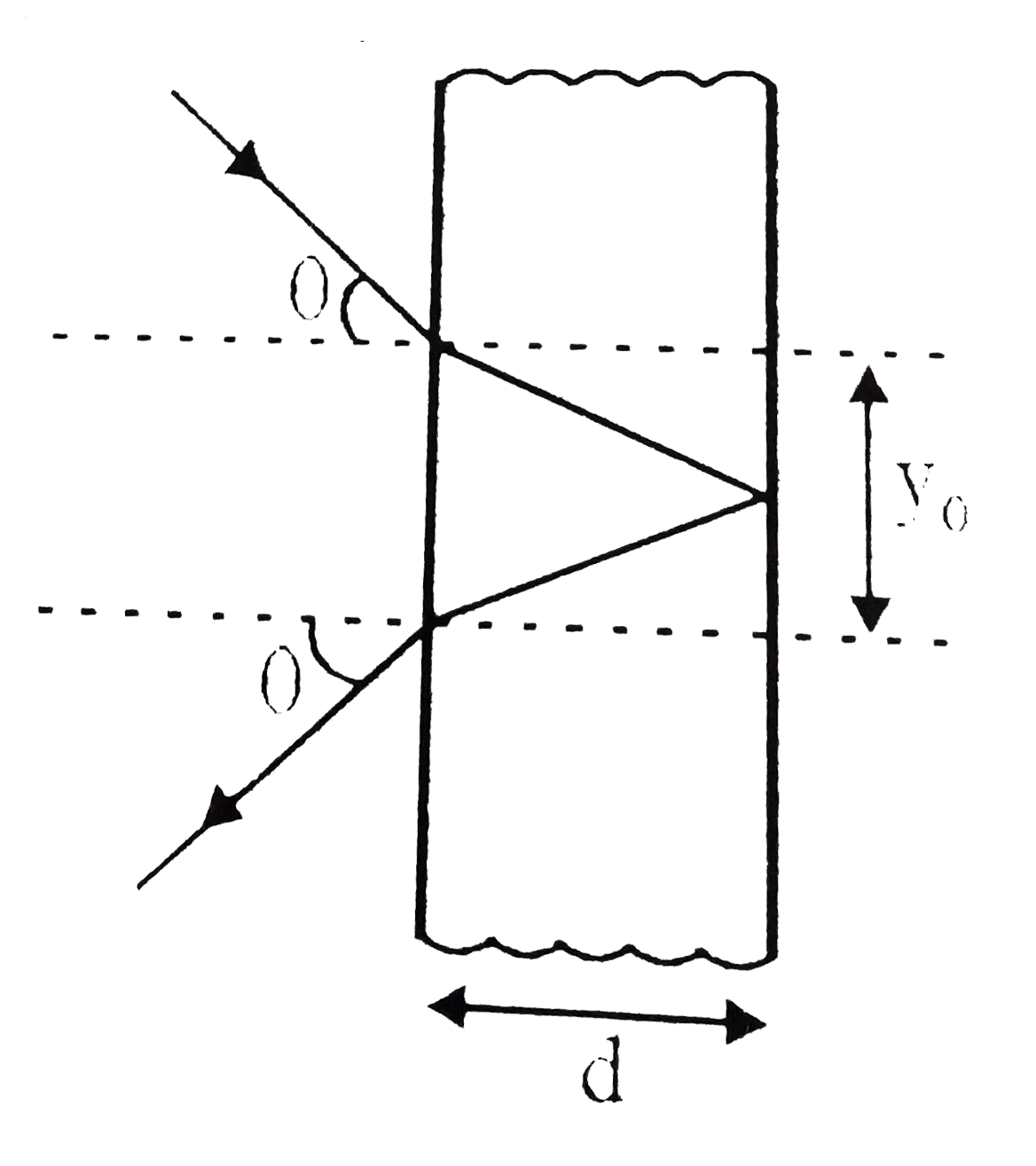 A2dsinθ√n2−sin2θB2dsinθ√sin2θ−1n2C2d√n2−sin2θsinθDNone of these
A2dsinθ√n2−sin2θB2dsinθ√sin2θ−1n2C2d√n2−sin2θsinθDNone of these
A ray of light falls on a glass plate of refractive index μ=1.5.
What is the angle of incidence of the ray if the angle between the reflected and
refracted rays is 90∘?View SolutionA slab of water is on the top of a glass slab of refractive index 2. At what angle to the normal must a ray be incident on the top sureface of th eglass slab so that it is reflected from the bottom surface as shown in figure ?
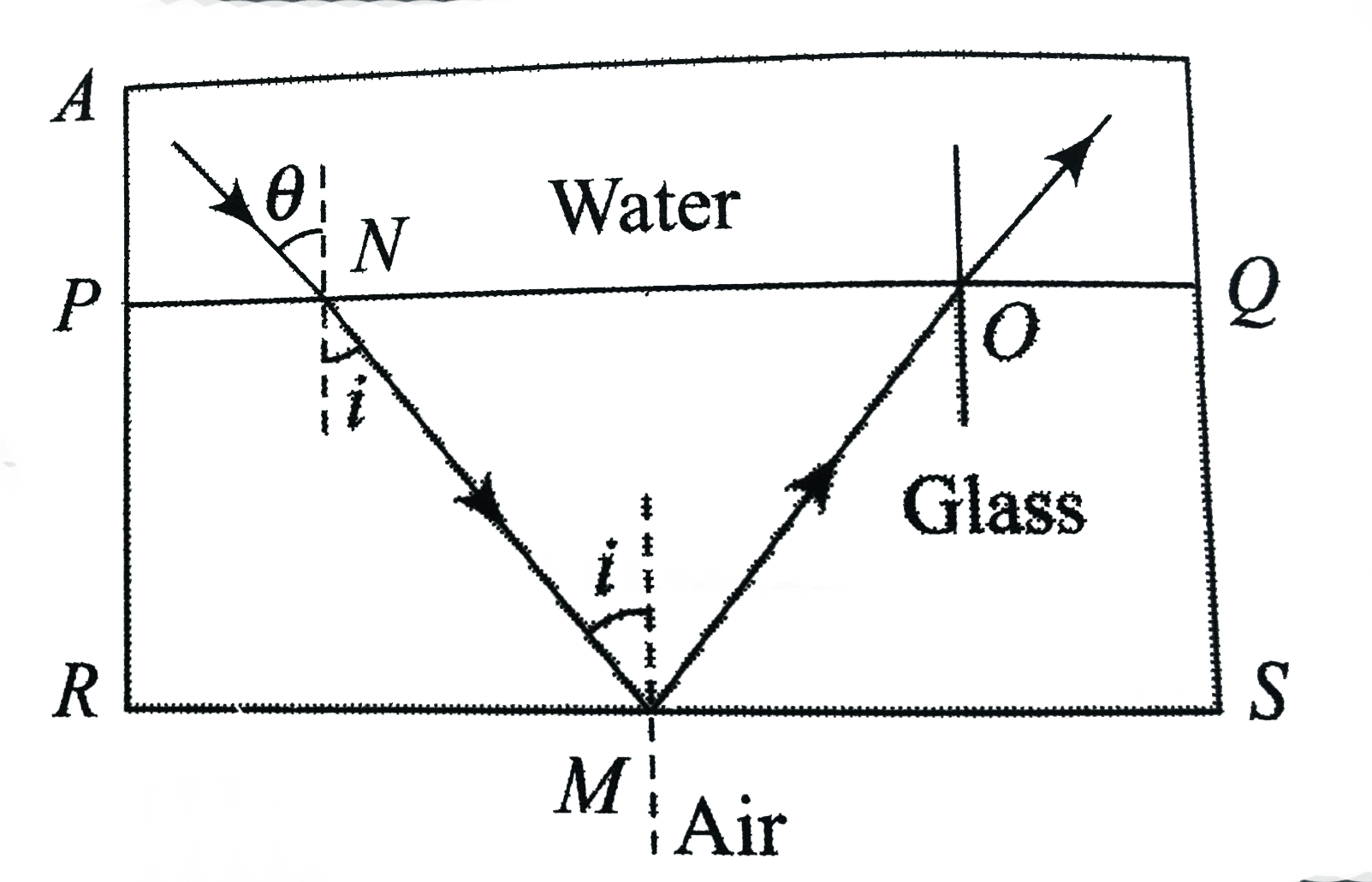 View Solution
View SolutionA ray incident at a point at an angle of incidence of 60∘ enters a glass sphere with refractive index √3 and it is reflected and refracted at the farther surface of the sphere. The angle between the reflected and refracted rays at this surface is:
View SolutionA ray of light is incident on a glass slab at an angle of 45∘. If refractive index of glass be 1.6, what is the angle of refraction ?
View SolutionA ray of light passes throught a plane glass slab of thickness t and refractive index μ=1.5. The angle between incident ray and emergent ray will be
View Solution

