A particle starts from rest and moves under constant acceleration in a straight line. Find the ratio of displacement (a) in successive second and (b) in successive time interval t0.
To solve the problem, we will break it down into two parts: (a) finding the ratio of displacement in successive seconds, and (b) finding the ratio of displacement in successive time intervals t0.
Part (a): Ratio of Displacement in Successive Seconds
1. Understanding the motion: The particle starts from rest and moves under constant acceleration. The initial velocity u=0 and the acceleration is a.
2. Displacement in the first second:
The displacement s1 during the first second can be calculated using the formula:
s1=ut+12at2
Substituting u=0 and t=1:
s1=0⋅1+12a(12)=12a
3. Displacement in the second second:
The displacement s2 during the second second can be calculated as:
s2=ut+12at2
For the second second, the total time is 2 seconds, so we need to find the displacement from 0 to 2 seconds and subtract the displacement from 0 to 1 second:
stotal_2=12a(22)=2a
Now, subtract the displacement in the first second:
s2=stotal_2−s1=2a−12a=32a
4. Finding the ratio:
Now, we find the ratio of displacements in the second second to the first second:
Ratio=s2s1=32a12a=31=3
Part (b): Ratio of Displacement in Successive Time Intervals
1. Displacement in the first time interval t0:
We already calculated this as s1=12a.
2. Displacement in the second time interval t0:
The second time interval is from t=1 to t=2, which we calculated as s2=32a.
3. Finding the ratio:
The ratio of displacements in the two intervals is:
Ratio=s2s1=32a12a=31=3
Final Answers:
(a) The ratio of displacement in successive seconds is 3.
(b) The ratio of displacement in successive time intervals t0 is also 3.
The displacement s1 during the first second can be calculated using the formula:
s1=ut+12at2
Substituting u=0 and t=1:
s1=0⋅1+12a(12)=12a
The displacement s2 during the second second can be calculated as:
s2=ut+12at2
For the second second, the total time is 2 seconds, so we need to find the displacement from 0 to 2 seconds and subtract the displacement from 0 to 1 second:
stotal_2=12a(22)=2a
Now, subtract the displacement in the first second:
s2=stotal_2−s1=2a−12a=32a
Now, we find the ratio of displacements in the second second to the first second:
Ratio=s2s1=32a12a=31=3
We already calculated this as s1=12a.
The second time interval is from t=1 to t=2, which we calculated as s2=32a.
The ratio of displacements in the two intervals is:
Ratio=s2s1=32a12a=31=3
(a) The ratio of displacement in successive seconds is 3.
(b) The ratio of displacement in successive time intervals t0 is also 3.
Topper's Solved these Questions
Similar Questions
A particle starts from rest and moves under constant acceleration in a straight line. Find the ratio of time in successive displacement d.
View SolutionA partical is moving in a straight line under constant acceletation. If the motion starts from rest, Find the ratio of displacement in n second to that in the nth second.
View Solution
Knowledge Check
- Question 1 - Select One
A particle starts from rest and moves with constant acceleration. Then velocity displacement curve is:
A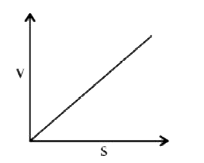 B
B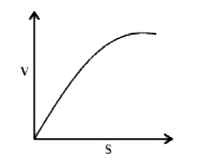 C
C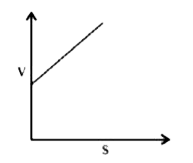 D
D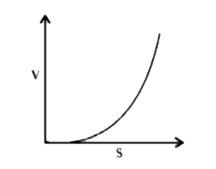
- Question 2 - Select One
A particle starting from rest moves along a straight line with constant acceleration for this velocity displacement graph will have the form-
A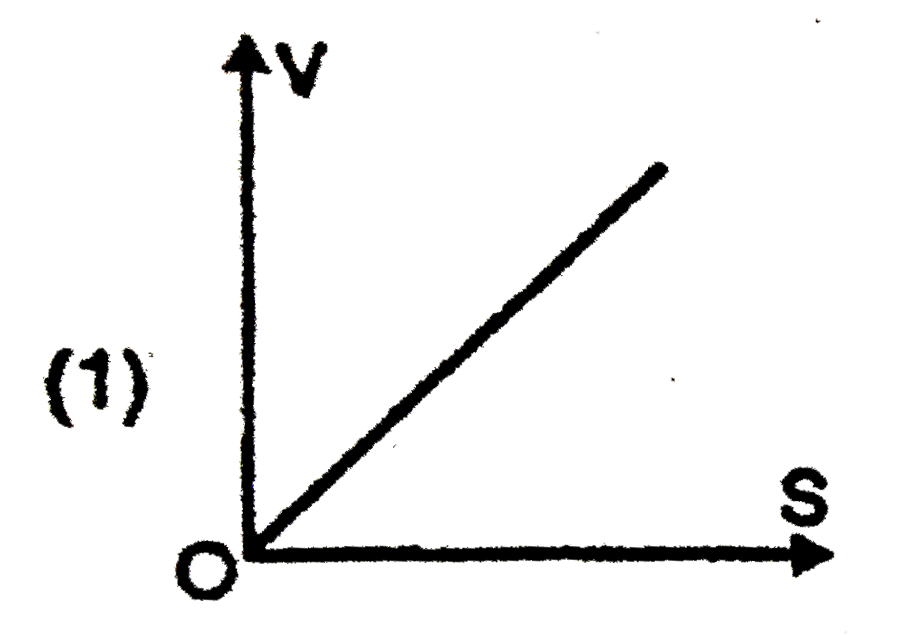 B
B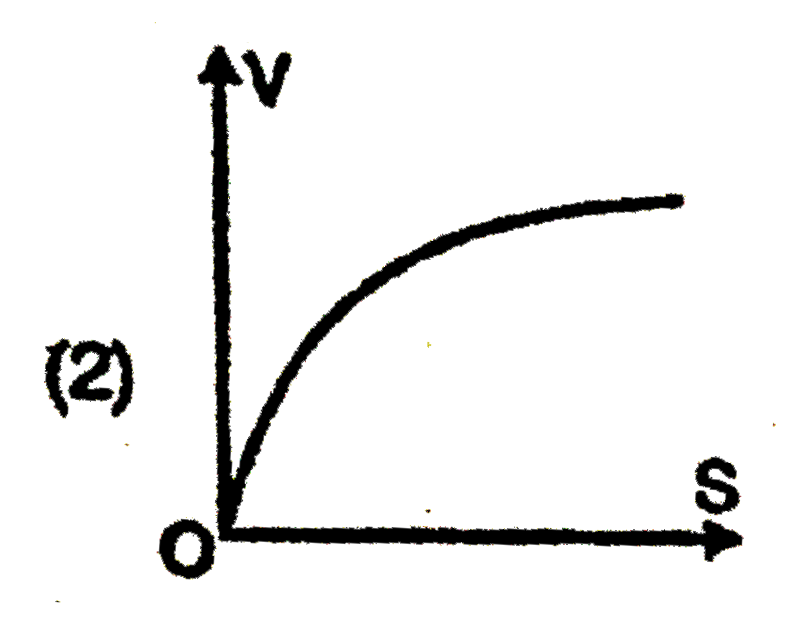 C
C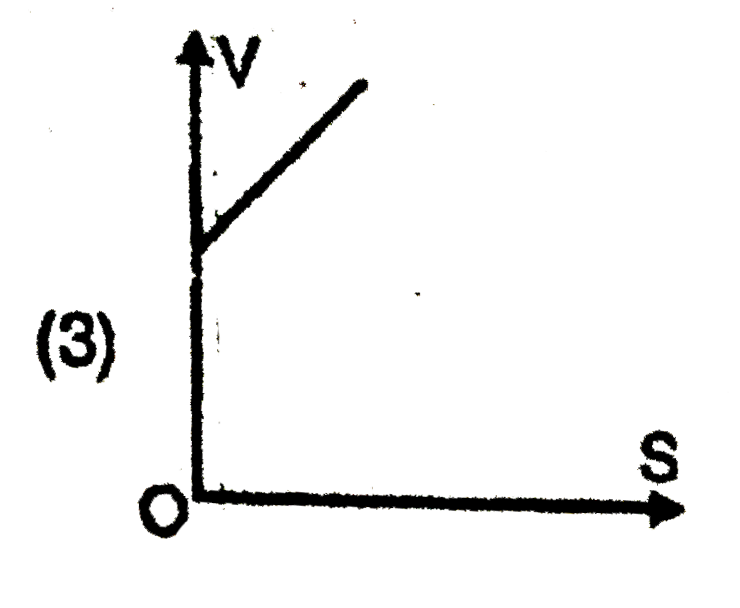 D
D
- Question 3 - Select One
A body starts from rest and moves with constant acceleration. The ratio of distance covered by the body in nth second to that covered in n second is.
A1nB2n−1n2Cn22n−1D2n−12n2
A wheel rotates under constant angular acceleration starting from rest. Find the ratio of angle rotated in nsecond and nthsecond.
View SolutionA particle starts from rest at t=0 and undergoes an acceleration as shown in figure.
Draw the displacement-time graph of the motion of the particle during the same time interval. In both the above cases, explain the various steps.
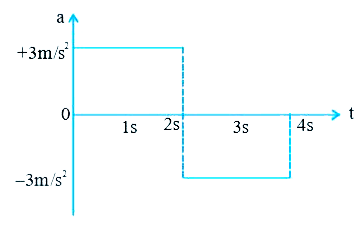 View Solution
View SolutionA particle starts moving from position of rest under a constant acceleration if it travels a distance x in t second, what distance will it travel in next t second?
View SolutionA particle, starting from rest, moves in a straight line with constant acceleration a0 for a time interval t0.Then is accelerates at a constant rate 2a0 for time interval 2t0 .The distance travelled by the particle in time 3t0 from the starts is S . Then Sa0t20=
View SolutionA particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is
 View Solution
View Solution

