A particle moves in a straight line as s=α(t−4)+β(t−4)2. Find the initial velocity and acceleration.
s=α(t−4)+β(t−4)2
=α(t−4)+β(t2−8t+16)
v=dsdt=α(1−0)+β(2t−8)
a=dvdt=β(2.1)=2β
At t=0,v=α−8β
a=2β
Topper's Solved these Questions
Similar Questions
The displacement of a particle moving along a straight line is given by
s=3t^(3)+2t^(2)-10t. find initial velocity and acceleration of particle.View SolutionA partical moves in a straight line as s=α(t−2)3+β(2t−3)4, where α and β are constants. Find velocity and acceleration as a function of time.
View Solution
Knowledge Check
- Question 1 - Select One
The acceleration of a particle, moving in a straight line, at time t is (2t+1)m/sec2 . If 4m/sec is the initial velocity of the particle then its velocity after 2 sec. is
A10cm/secB8cm/secC4cm/secDNone of these - Question 2 - Select One
For a particle moving in a straight line assume s,v,a and t represents displacement, velocity, acceleration and time respectively.
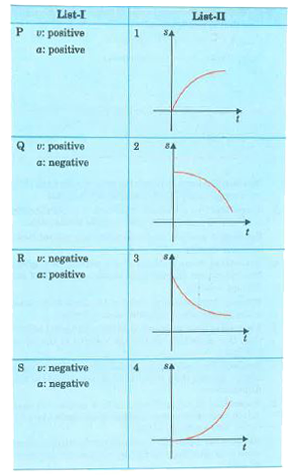 APQRS3241BPQRS1324CPQRS2134DPQRS4132
APQRS3241BPQRS1324CPQRS2134DPQRS4132
The displacement of a particle moving in a straight line is given by s=t3−6t2+3t+4 meters.The velocity when acceleration is zero is "
View SolutionThe acceleration after t seconds of a particle which starts from rest and moves in a straight line is (8−t5)cms2. Find the velocity of the particle when the acceleration is zero.
View SolutionA particle is moving in a straight line with initial velocity u and uniform acceleration f. If the sum of the distances travelled in tthand(t+1)th seconds is 100cm, then its velocity after t seconds, in cm/s, is.
View SolutionA particle moves in a straight line with a uniform acceleration a. Initial velocity of the particle is zero. Find the average velocity of the particle in first 's' distance.
View SolutionThe space s described in time t by a particle moving in a straight line is given by s=t5−40t3+30t2+80t−250 . Find the minimum value of acceleration.
View SolutionFigure shows the displacement-time graph of a particle moving in a straight line. Find the signs of velocity and acceleration of particle at time t=t1andt=t2.
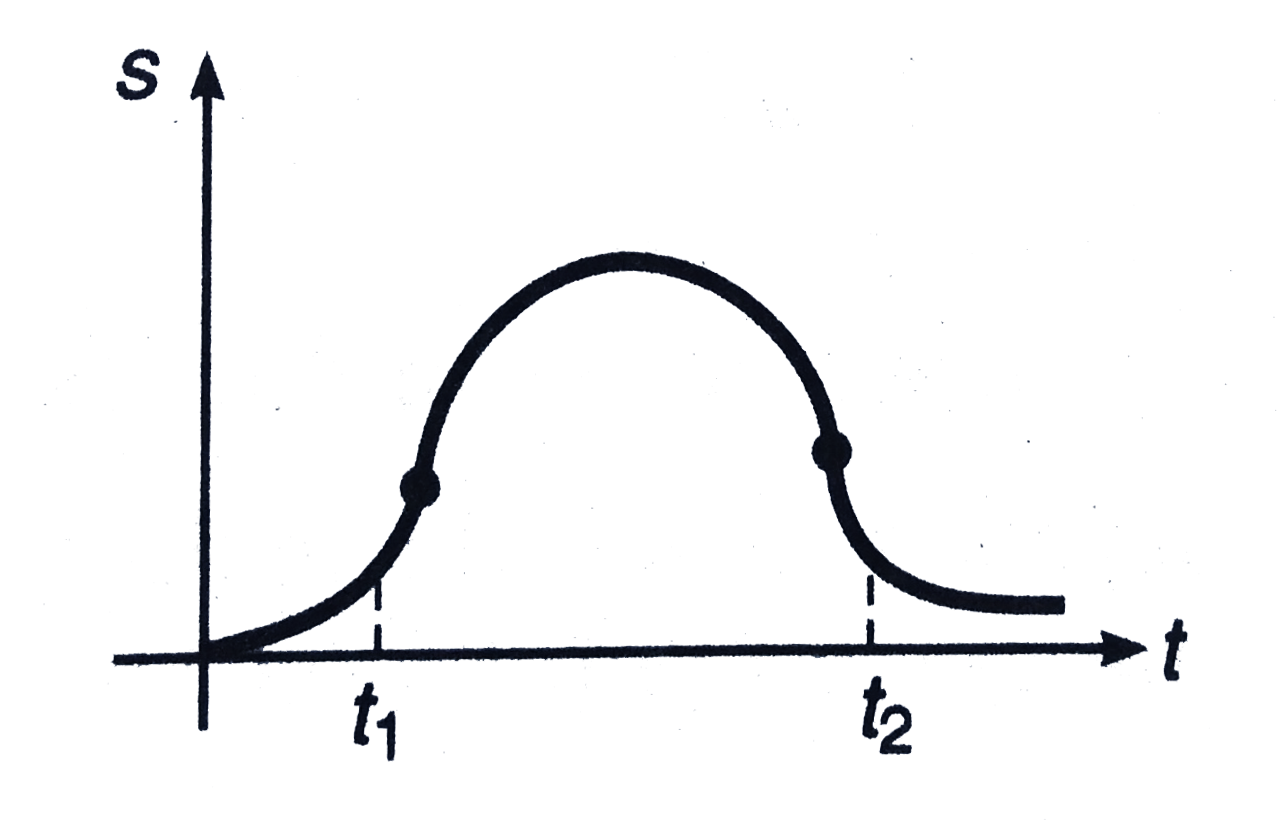 View Solution
View Solution

