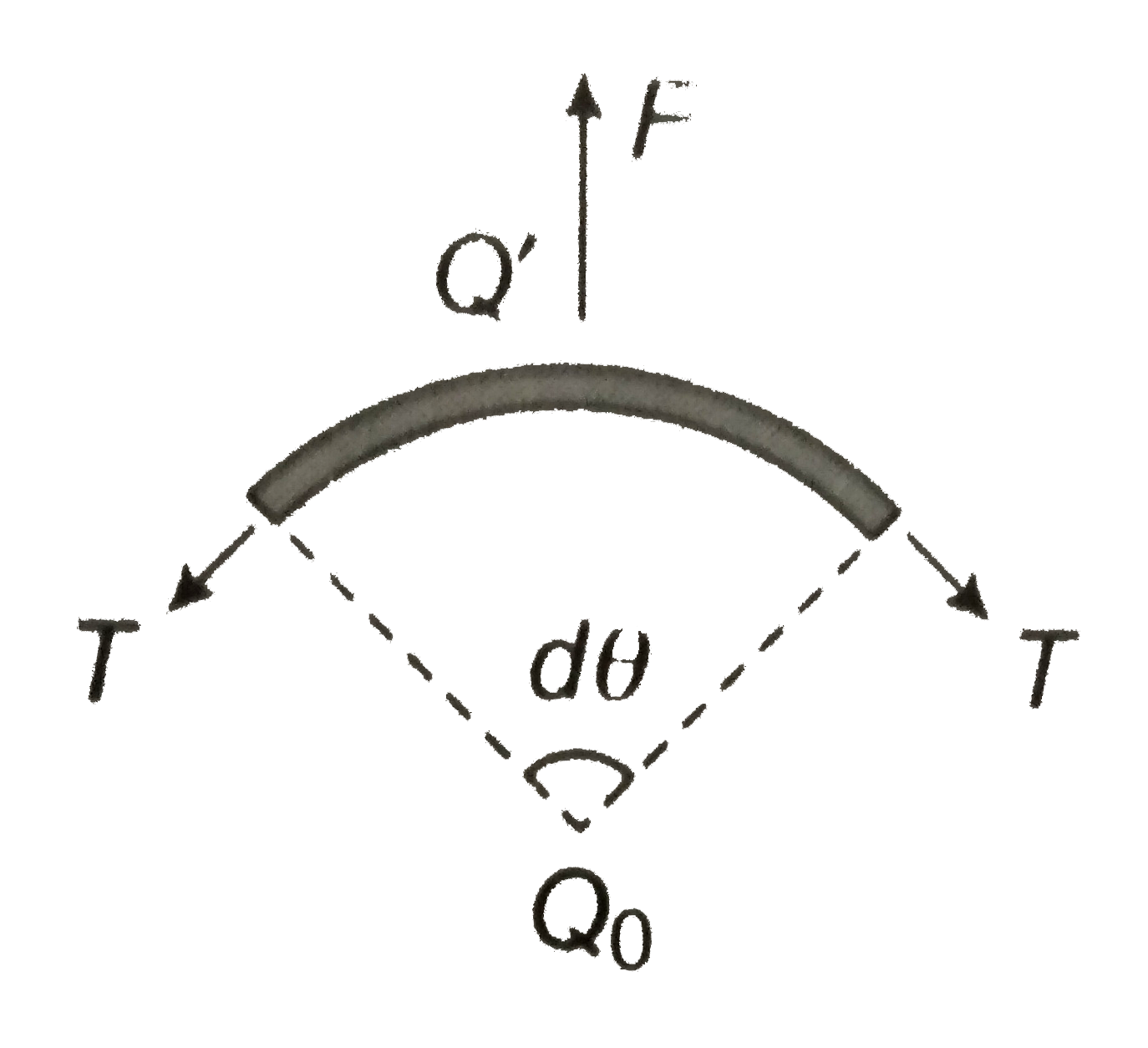
A ring of radius R is having charge Q uniformly distributed. A point charge Q0 is placed at the centre of the ring. Find tension developed in the ring. If radius of cross - section of the ring is a and Young's modulus of wire is Y, find increase in the radius of ring.
To find tension//compression in the ring, find the force on small length of angle dθ and remove dθ from the expression (refer to Circular Motion , Example 33 , page 351 Vol. 1).
Charge on small length of angle dθ
Q'=Q2πRdl=Q2πRRdθ=Qdθ2π
Electric force on Q' ,
F=14π∈0Q'Q0R2=14π∈0.QQ02πR2dθ
Tension in the string
T=QQ08π2∈0R2
Let increase in the radius of ring is ΔR.
Longitudinal strain
∈=2π(R+ΔR)−2πR2πR=ΔRR
Longitudinal stress
σ=TA=Tπd2
where a: radius of cross - section of ring.
Y=σ∈=T/πa2ΔR/R
ΔR=TRπa2Y=QQ08π2∈0R2.Rπa2Y
=QQ08π3∈0RYa2
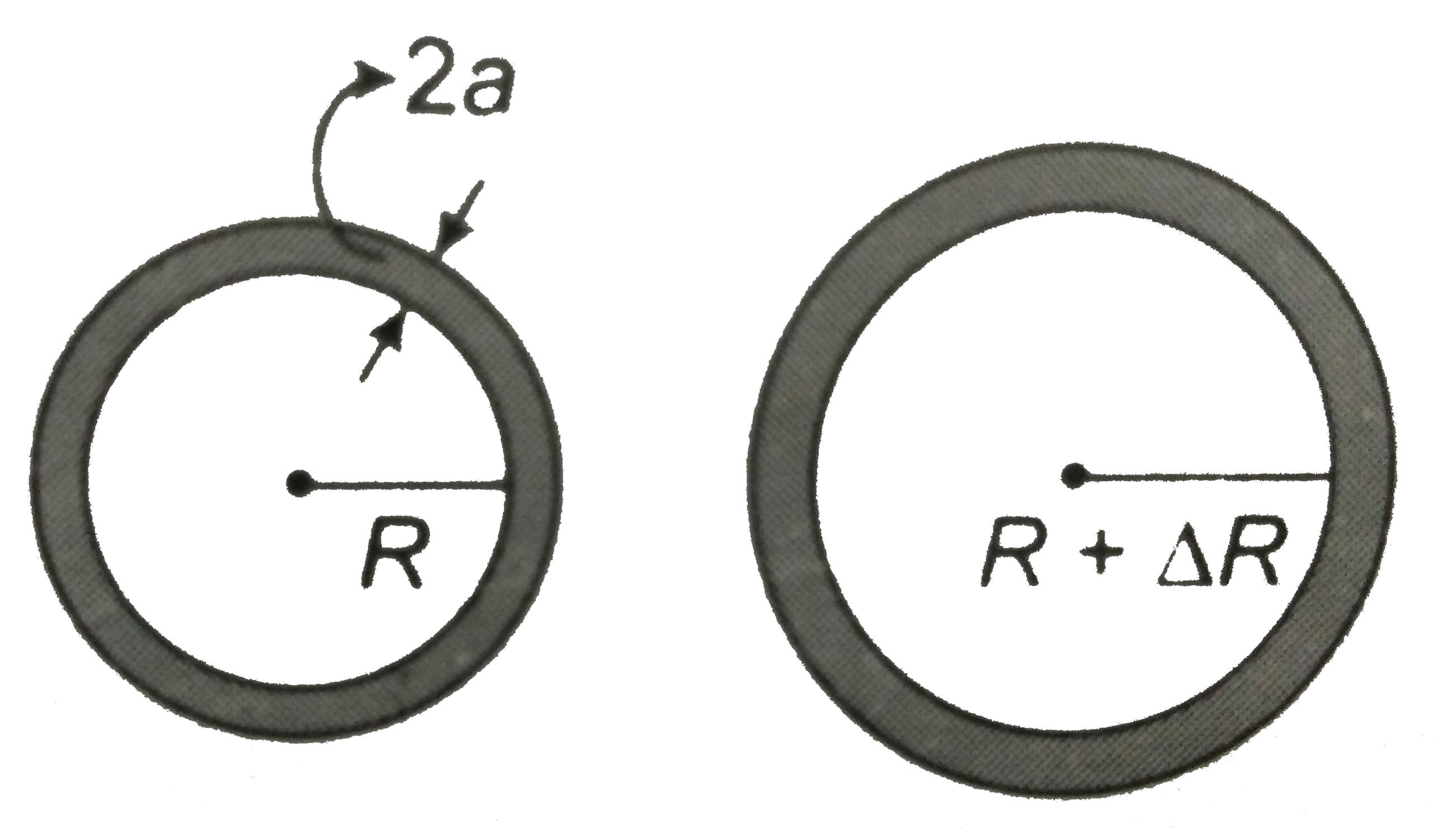
Topper's Solved these Questions
Similar Questions
A ring of radius R is with a uniformly distributed charge Q on it .A charge q is now placed in the centre of the ring .Find the increment in tension in the ring.
View SolutionA ring of radius R with a uniformly distributed charge q as shown in figure. A charge q0, is now placed at the centre of the ring. Find the increment in-the tension in ring.
View Solution
Knowledge Check
Charges q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
Aq2π2ε0R2Bq4π2ε0R2Cq4πε0R2Dq2πε0R2
A thin circular wire of radius r has a charge Q. If a point charge q is placed at the centre of the ring, then find the increase in tension in the wire.
View SolutionA thin circular wire of radius r has a charge Q. If a point charge q is placed at the centre of the ring, then find the increase in tension in the wire.
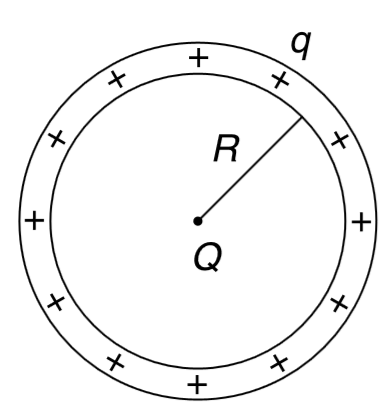
View SolutionA ring of radius R has uniformly distributed charge q. A point charge Q is placed at the centre of the ring. (a) Find the increase in tension in the ring after the point charge is placed at its centre. (b) Find the increase in force between the two semicircular parts of the ring after the point charge is placed at the centre. (c) Using the result found in part (b) find the force that the point charge exerts on one half of the ring.
View SolutionCharge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.

View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The

View SolutionA quarter ring of radius R is having uniform charge density λ. Find the electric field and potential at the centre of the ring.
View Solution
