A ring of radius R is with a uniformly distributed charge Q on it .A charge q is now placed in the centre of the ring .Find the increment in tension in the ring.
To solve the problem of finding the increment in tension in the ring when a charge q is placed at the center, we can follow these steps:
Step 1: Understand the Setup
We have a ring of radius R with a uniformly distributed charge Q on it. A point charge q is placed at the center of the ring.
Step 2: Calculate the Linear Charge Density
The linear charge density λ of the ring can be calculated as:
λ=Q2πR
This is because the total charge Q is distributed uniformly over the circumference of the ring, which is 2πR.
Step 3: Consider a Small Element of the Ring
Take a small element of the ring dθ subtending an angle at the center. The charge on this small element is:
dQ=λ⋅R⋅dθ=Q2πR⋅R⋅dθ=Q2πdθ
Step 4: Calculate the Force on Charge q
The force dF exerted by the small charge dQ on the charge q at the center can be expressed using Coulomb's law:
dF=kq⋅dQR2=kq⋅Q2πdθR2
where k is Coulomb's constant.
Step 5: Resolve the Tension Components
The tension T in the ring has components acting at an angle due to the symmetry of the ring. The vertical components of tension from two opposite segments of the ring will balance the radial force due to dF.
The vertical component of tension from each side of the small element is:
Tsin(dθ2)
Since there are two such components, the total vertical component is:
2Tsin(dθ2)
Step 6: Equate the Forces
For equilibrium, the total vertical component of tension must balance the force dF:
2Tsin(dθ2)=dF
Substituting dF:
2Tsin(dθ2)=kq⋅Q2πdθR2
Step 7: Approximate for Small Angles
For small angles, sin(dθ2)≈dθ2:
2T⋅dθ2=kq⋅Q2πdθR2
This simplifies to:
Tdθ=kqQ2πR2dθ
Step 8: Solve for Tension Increment
Dividing both sides by dθ (assuming dθ≠0):
T=kqQ2πR2
This gives us the increment in tension in the ring due to the charge q placed at the center.
Final Answer
The increment in tension in the ring is:
ΔT=kqQ2πR2
We have a ring of radius R with a uniformly distributed charge Q on it. A point charge q is placed at the center of the ring.
The linear charge density λ of the ring can be calculated as:
λ=Q2πR
This is because the total charge Q is distributed uniformly over the circumference of the ring, which is 2πR.
Take a small element of the ring dθ subtending an angle at the center. The charge on this small element is:
dQ=λ⋅R⋅dθ=Q2πR⋅R⋅dθ=Q2πdθ
The force dF exerted by the small charge dQ on the charge q at the center can be expressed using Coulomb's law:
dF=kq⋅dQR2=kq⋅Q2πdθR2
where k is Coulomb's constant.
The tension T in the ring has components acting at an angle due to the symmetry of the ring. The vertical components of tension from two opposite segments of the ring will balance the radial force due to dF.
Tsin(dθ2)
Since there are two such components, the total vertical component is:
2Tsin(dθ2)
For equilibrium, the total vertical component of tension must balance the force dF:
2Tsin(dθ2)=dF
Substituting dF:
2Tsin(dθ2)=kq⋅Q2πdθR2
For small angles, sin(dθ2)≈dθ2:
2T⋅dθ2=kq⋅Q2πdθR2
This simplifies to:
Tdθ=kqQ2πR2dθ
Dividing both sides by dθ (assuming dθ≠0):
T=kqQ2πR2
This gives us the increment in tension in the ring due to the charge q placed at the center.
The increment in tension in the ring is:
ΔT=kqQ2πR2
Topper's Solved these Questions
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - C.U.Q
133 Videos
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Level 1(C.W)
24 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - ADDITIONAL PROBLEMS
14 Videos
- EXPERIMENTAL PHYSICSBOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Comprehension type
6 Videos
Similar Questions
A ring of radius R with a uniformly distributed charge q as shown in figure. A charge q0, is now placed at the centre of the ring. Find the increment in-the tension in ring.
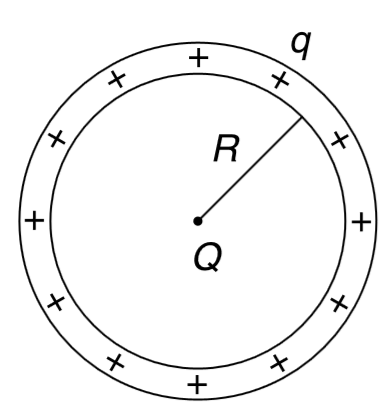
View SolutionA ring of radius R has uniformly distributed charge q. A point charge Q is placed at the centre of the ring. (a) Find the increase in tension in the ring after the point charge is placed at its centre. (b) Find the increase in force between the two semicircular parts of the ring after the point charge is placed at the centre. (c) Using the result found in part (b) find the force that the point charge exerts on one half of the ring.
View Solution
Knowledge Check
Which statement is true :-
(i) A ring of radius R carries a uniformly distributed charge +Q. A point charge -q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a smiple harmonic motion along the axis of the ring.
(ii) Electrons move from a region of higher potential to that of lower potentialAonly (i)Bonly (ii)C(i), (ii)Dnone of themCharges q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
Aq2π2ε0R2Bq4π2ε0R2Cq4πε0R2Dq2πε0R2A positive charge Q is uniformly distrubuted along a circular ring of radius R.a small test chage q is placed at the centre of the ring .The
 Aif q>0 and is displaced away from the centre in the plane of the ring.it will be pushed back towards the centre.Bif q<0 and is displaced away from the cnetre in the plane of the ring .it will never return to the centre and will continue moving till it hits the ring.Cif q<0 it will perform SHM for small displacement along the axisDq at the centre of the ring is in an unstable equilibrium within the plane of the ring for q<0
Aif q>0 and is displaced away from the centre in the plane of the ring.it will be pushed back towards the centre.Bif q<0 and is displaced away from the cnetre in the plane of the ring .it will never return to the centre and will continue moving till it hits the ring.Cif q<0 it will perform SHM for small displacement along the axisDq at the centre of the ring is in an unstable equilibrium within the plane of the ring for q<0
A ring of radius R is having charge Q uniformly distributed. A point charge Q0 is placed at the centre of the ring. Find tension developed in the ring. If radius of cross - section of the ring is a and Young's modulus of wire is Y, find increase in the radius of ring.
View SolutionA ring of radius R carries a uniformly distributed charge +Q. A point charge −q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a ismple harmonic motion along the axis of the ring.
View SolutionA thin circular wire of radius r has a charge Q. If a point charge q is placed at the centre of the ring, then find the increase in tension in the wire.
View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View SolutionA ring of radius R having total charge Q uniformly distributed over the ring has a charge particle q kept at its centre. If a transverse pulse is created on the ring, then find the time when pulse completes one revolution.(Total mass of ring is m)
View Solution
