The volume charge density inside of sphere of radius $R$ is given by $ρ = ρ_{0} (1 - \frac{r}{R})$ ,where $ρ_{0}$ is constant and $r$ distance from centre.
(a) Find total charge $Q$ inside sphere.
Electric field outside sphere at distance $r$ from centre.
(b) Electric field outside sphere at distance $r$ from centre.
(c) Electric field inside sphere at distance $r$ from centre.
(d) At what distance from centre electric field is maximum and its value?
(e ) Sketch $E$ versus $r$ graph.

Text Solution

Verified by Experts

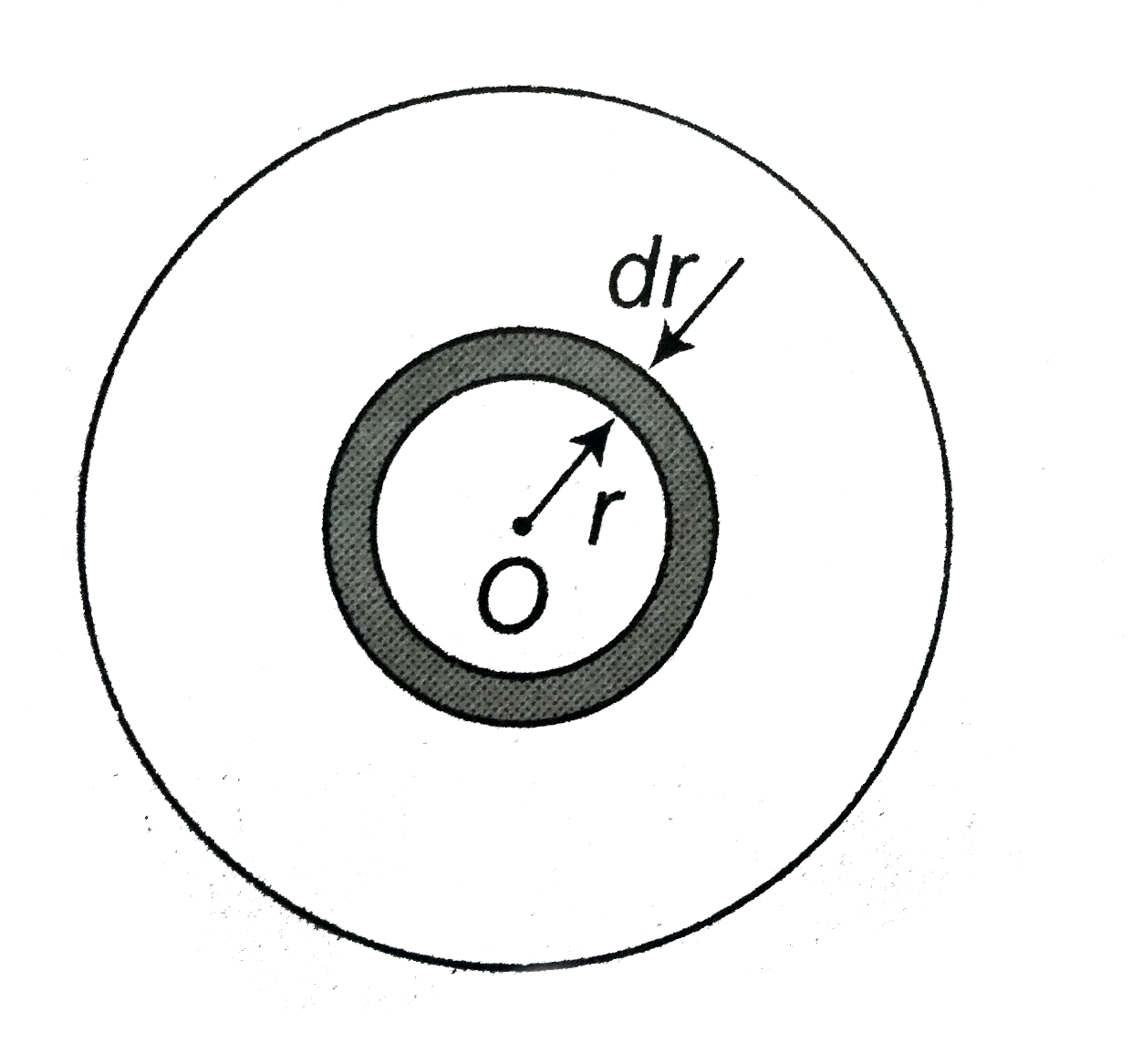
To take element on spherical volume , we choose a spherical shell of radius $r$ and thickness $d r$
Volume of element
$d V = \frac{4}{3} π {(r + d r)}^{3} - \frac{4}{3} π r^{3} = 4 π r^{2} d r$
(a) Small charge in small volume
$d q = ρ d V = ρ_{0} (1 - \frac{r}{R}) 4 π r^{2} d r$
$Q = 4 π ρ_{0} \int_{0}^{2} (r^{2} = \frac{r^{3}}{R}) d r$
$= 4 π ρ_{0} {∣ ∣ ∣ \frac{r^{3}}{3} - \frac{r^{4}}{4 R} ∣ ∣ ∣}_{0}^{R} = 4 π ρ_{0} (\frac{R^{3}}{3} - \frac{R^{4}}{4 R})$
$= \frac{4 π ρ_{0} R^{3}}{12} = \frac{π ρ_{0} R^{3}}{3}$
(b) For a point $(r > R)$ , whole charge is assumed to be concentrated at centre
$E = \frac{1}{4 π \in_{0}} \frac{Q}{r^{2}} = \frac{1}{4 π \in_{0}} . \frac{π ρ_{0} R^{3}}{3 r^{2}} = \frac{ρ_{0} R^{3}}{12 \in_{0} r^{2}}$ .
$E$ versus $r$ will be rectangular hyperbola type .
At $r = R, E = \frac{ρ_{0} R}{12 \in_{0}}$
(c ) For a point $(r < R)$ , change inside sphere of radius $r$
$q = \int_{0}^{r} ρ d V = \int_{0}^{r} ρ_{0} (1 - \frac{r}{R}) 4 π r^{2} d r = 4 π ρ_{0} \int_{0}^{2} (r^{2} - \frac{r^{3}}{R}) d r$
$= 4 π ρ_{0} {∣ ∣ ∣ \frac{r^{3}}{3} - \frac{r^{4}}{4 R} ∣ ∣ ∣}_{0}^{R} = 4 π ρ_{0} (\frac{r^{3}}{3} - \frac{r^{4}}{4 R})$
$E = \frac{1}{4 π \in_{0}} . \frac{q}{r^{2}} = \frac{1}{4 π \in_{0}} . 4 π ρ_{0} (\frac{r}{3} - \frac{r^{2}}{4 R})$
$= \frac{ρ_{0}}{\in_{0}} (\frac{r}{3} - \frac{r^{2}}{4 R})$ ,
$E$ versus $r$ will be parabola open downward.
At $r = R, E = \frac{ρ_{0}}{\in_{0}} (\frac{R}{3} - \frac{R^{2}}{4 R}) = \frac{ρ_{0} R}{12 \in_{0}}$
(d) If $r > R$ i.e. outside sphere , electric field is decreasing continuously as
$E = \frac{ρ_{0} R^{3}}{12 \in_{0} r^{2}}$
The electric field will be maximum inside sphere $(r < R)$ ,
$E = \frac{ρ_{0}}{\in_{0}} (\frac{r}{3} - \frac{r^{2}}{4 R})$
For $E$ to be maximum
$\frac{d E}{d r} = \frac{ρ_{0}}{\in_{0}} (\frac{1}{3} - \frac{2 r}{4 R}) = 0 \Rightarrow r = \frac{2 R}{3}$
$E_{max} = \frac{ρ_{0}}{\in_{0}} [\frac{2 R}{9} - \frac{R}{9}] = \frac{ρ_{0} R}{9 \in_{0}}$
(e ) $E$ versus $r$ graph

A hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
AIncreases as r increases for $r < R and$ for $r > R$
BZero as r increases for $r < R$ , decreases as r increases for $r > R$
CZero as r increases for $r < R$ , increases as r increases for $r > R$
DDecreases as r increases for $r < R and$ for $r > R$
A solid sphere of radius R has a volume charge density $ρ = ρ_{0} r^{2}$ (where $ρ_{0}$ is a constant ans r is the distance from centre). At a distance x from its centre (for $x < R$ ), the electric field is directly proportional to :
A $1 / x^{2}$
B $1 / x$
C $x^{3}$
D $x^{2}$
A hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
Adecreases as r increases for $r < R$ and $r > R$
Bincreases as r increases for $r < R$ and $r > R$
Czero as r increases for $r < R$ , decreases as r increases for $r > R$
Dzero as r increases for $r < R$ , increases for $r > R$