No. of Direct and Transverse Common Tangents When $| C_{1} C_{2} | = r_{1} + r_{2}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To determine the number of direct and transverse common tangents when the distance between the centers of two circles $| C_{1} C_{2} |$ is equal to the sum of their radii $r_{1} + r_{2}$ , we can follow these steps:
1. Understanding the Condition:
We are given that the distance between the centers of the circles $| C_{1} C_{2} |$ is equal to the sum of their radii $r_{1} + r_{2}$ . This means the two circles are externally tangent to each other.
2. Direct Common Tangents:
- When two circles are externally tangent, there are always two direct common tangents.
- These tangents do not intersect the line segment joining the centers of the circles and touch each circle at one point each.
- Therefore, for our case, we conclude that there are 2 direct common tangents.
3. Transverse Common Tangents:
- For transverse common tangents, we consider the tangents that intersect the line segment joining the centers of the circles.
- When the circles are externally tangent, there is only 1 transverse common tangent.
- This tangent touches both circles at the point where they are tangent to each other.
4. Final Count:
- Thus, when $| C_{1} C_{2} | = r_{1} + r_{2}$ , we have:
- Number of Direct Common Tangents: 2
- Number of Transverse Common Tangents: 1
Summary:
- Direct Common Tangents: 2
- Transverse Common Tangents: 1

Updated on:7/8/2024

There are two circles $C_{1}$ and $C_{2}$ whose radii are $r_{1}, r_{2}$ , respectively. If distance between their centre is $3 r_{1} - r_{2}$ and length of direct common tangent is twice of the length of transverse common tangent. Then $r_{1} : r_{2}$ is:
A $5 : 4$
B $6 : 5$
C $7 : 6$
D $8 : 7$
Instruction : Answer the questions given below on the basis following figure.

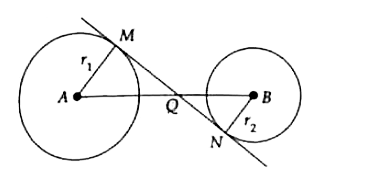
MN is a transverse common tangent. A and B are centres of the circles whose radii are respectively $r_{1} and r_{2}$ . Length of AB is d.
Length of MN is
A $\sqrt{d^{2} - {(r_{1} + r_{2})}_{1}^{2}}$
B $\sqrt{d^{2} - {(r_{1} - r_{2})}_{1}^{2}}$
C $\sqrt{d^{2} + {(r_{1} + r_{2})}_{1}^{2}}$
D $\sqrt{d^{2} + {(r_{1} + r_{2})}_{1}^{2}}$