For a small angled prism, angle of prism $A$ of minimum deviation $(δ)$ varies with the refractive index of the prism as shown in the graph
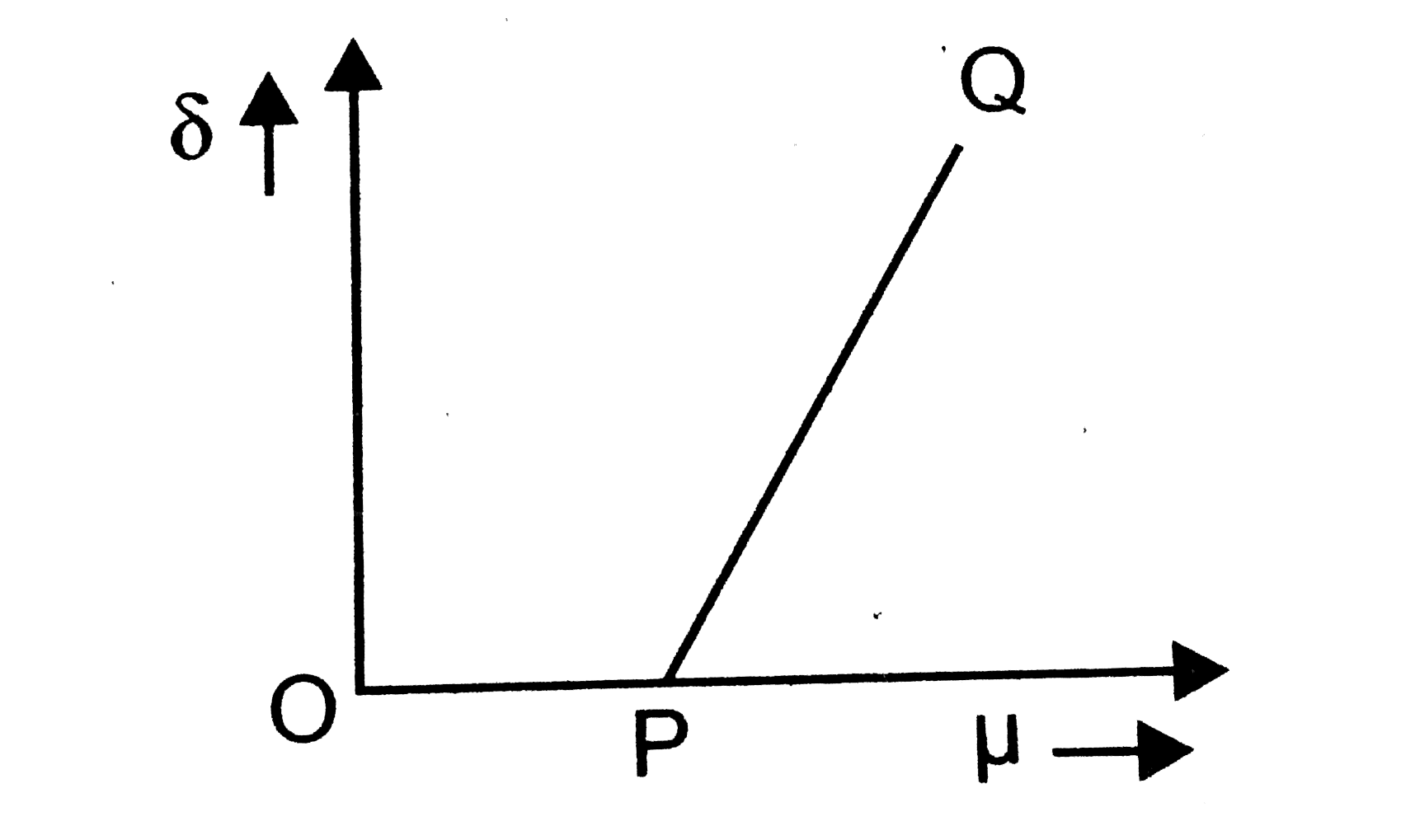
$For a small angled prism, angle of prism A of minimum deviation(delta) varies with the refractive index of the prism as shown in the graph$

A

Point P corresponds to $μ = 1 / 2$

B

Slope of the line $P Q = A / 2$

C

Slope=A

D

None of the above statements is true

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$δ = (μ - 1) A \Rightarrow y = (x - 1) A = x A - A$
Comparing with $y = m x + C$
Slope: $m = A$

|

Updated on:21/07/2023

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
For refraction through a small angled prism, the angle of minimum deviation
Aincreases with increase in refractive index of a prism
Bwill be $2 δ$ for a ray of refractive index $2.4$ if it is delta for a ray of refractive index $1.2$
Cis directly proportional to the angle of the prism
Dwill decrease with increase in refractive index of the prism
Question 2 - Select One
The angle of minimum deviation produced by an equilateral prism is $46^{\circ}$ The refractive index of material of the prism.
A1.6
B1.5
C1.4
D1.8
Question 3 - Select One
The angle of minimum deviation is an equilateral prism of refractive index $1.414$ is
A $60^{\circ}$
B $30^{\circ}$
C $90^{\circ}$
D $45^{\circ}$

CP SINGH-REFRACTION OF LIGHT BY PLANE SURFACES-Exercises

Home
Ask Doubt
Profile