Figure shows a spring fixed at the bottom end of an incline of inclination 370. A small block of mass 2 kg starts slipping down the incline from a point 4.8 m away from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find a. the frictioin coefficient between the plane and the block and b. the spring constant of the spring. Take g=10ms2.
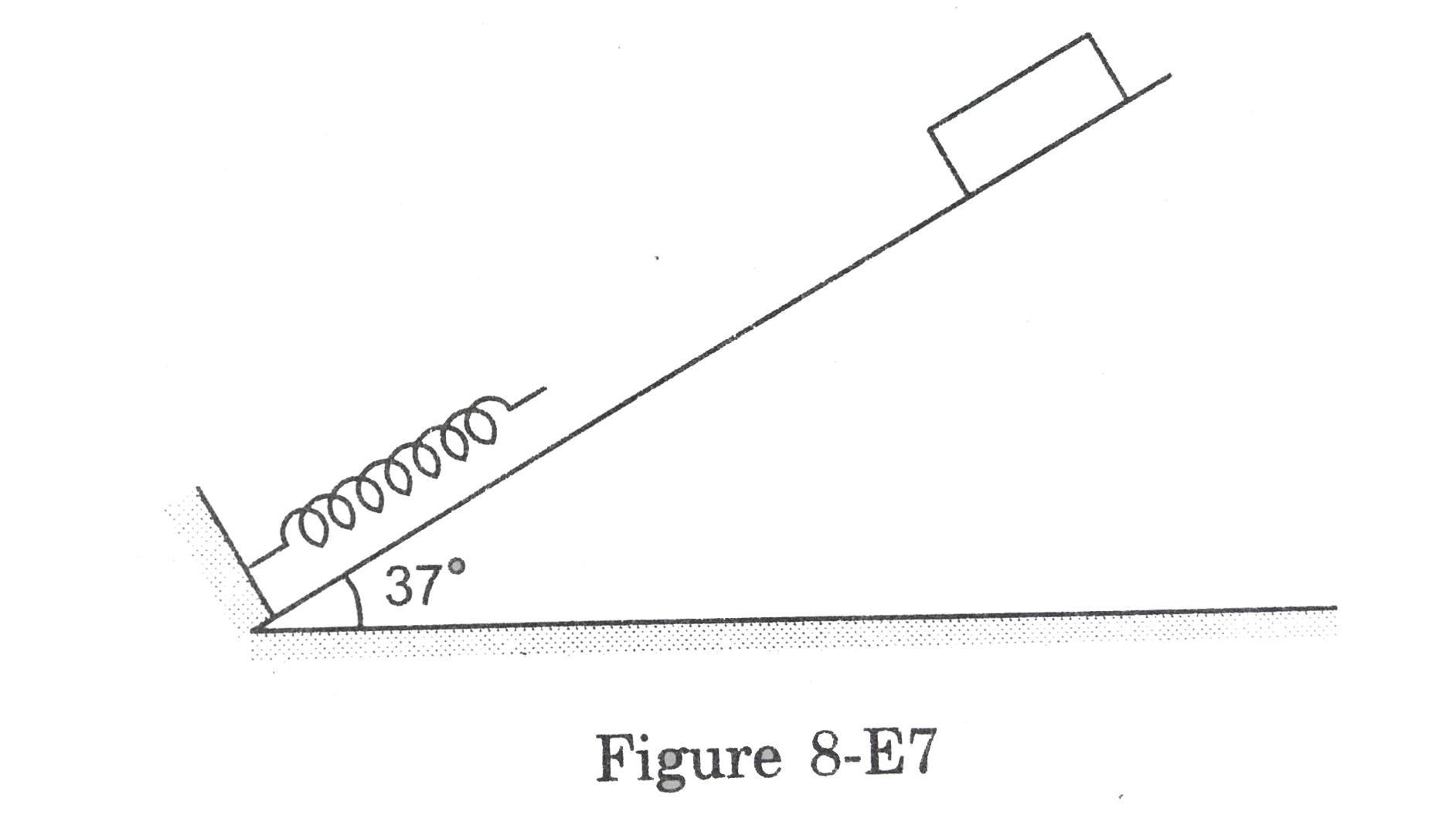
Applying work energy theorem for downward motion of the body W=ΔKE
mgsinθ(x+d)−f×l1−12Kx2=ΔKE
200sin370(5)−μ×20cos370×5−12K(0.2)2=0
80μ+0.02K=60→(1)
For the upward motion of the body
−mgsinθl2+(f×l2)+12Kx2=ΔKE
−2×10sin370×1−μ×20cos370×1+12K(0.2)2=0
16μ−0.02K=−12→(2)
Adding equations (1) and (2), we get
96μ=48⇒μ=0.5
Now, use the value of μ in equation (1),
we get K=1000N/m.
Topper's Solved these Questions
Similar Questions
A block of mass m moves with constant speed down the inclined plane of inclination θ. Find the coefficient of kinetic friction.
 View Solution
View SolutionFigure. shows a rough horizontal plane which ends in a vertical wall, to which a spring is connected, having a force constant k. Initially spring is in its relaxed state. A block of mass m starts with an initial velocity u towards the spring from a distance l0 from the end of spring shown. When block strikes at the end ofthe spring, it compresses the spring and comes to rest. Find the maximum compression in the spring. The friction coefficient between the block and the floor is μ..
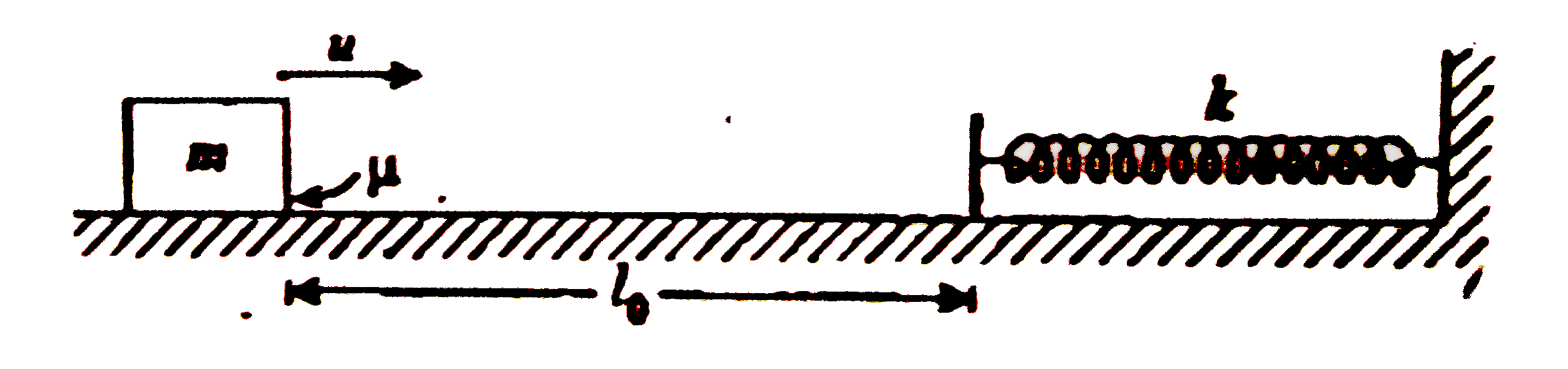 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m slips down an inclined plane as shown in the figure. When it reaches the bottom it presses the spring by a length (spring length ⟨h and spring constant = K)-
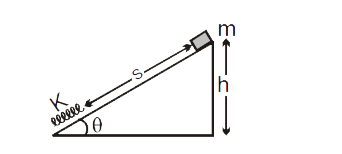 A(2mgh/K)1/2B(mgh/K)1/2C(2gh/mK)1/2D(gh/mK)1/2
A(2mgh/K)1/2B(mgh/K)1/2C(2gh/mK)1/2D(gh/mK)1/2 - Question 2 - Select One
block of mass m slips down an inclined plane as shown in the figure. When it reaches the bottom it presses the spring by a length (spring length ltlth and spring constant = K) - (A) (2mgh/K)1/2
 A(2mgh/K)1/2B(mgh/K)1/2C(2gh/mK)1/2D(gh//mK)^(1//2)
A(2mgh/K)1/2B(mgh/K)1/2C(2gh/mK)1/2D(gh//mK)^(1//2) - Question 3 - Select One
ϕ is the angle of the incline when a block of mass m just starts slipping down. The distance covered by the block if thrown up the incline with an initial speed v0 is :
Av204gsinϕB4v20gsinϕCv20sinϕ4gD4v20sinϕg
Figure shown two blocks in contact sliding down an inclined surface of inclination 30∘. The block of mass 2kgand the incline is μ1=0.20 and the incline is μ2=0.30 .Find the acceleration of 2.0kg block (in ms−2)g=10ms−2
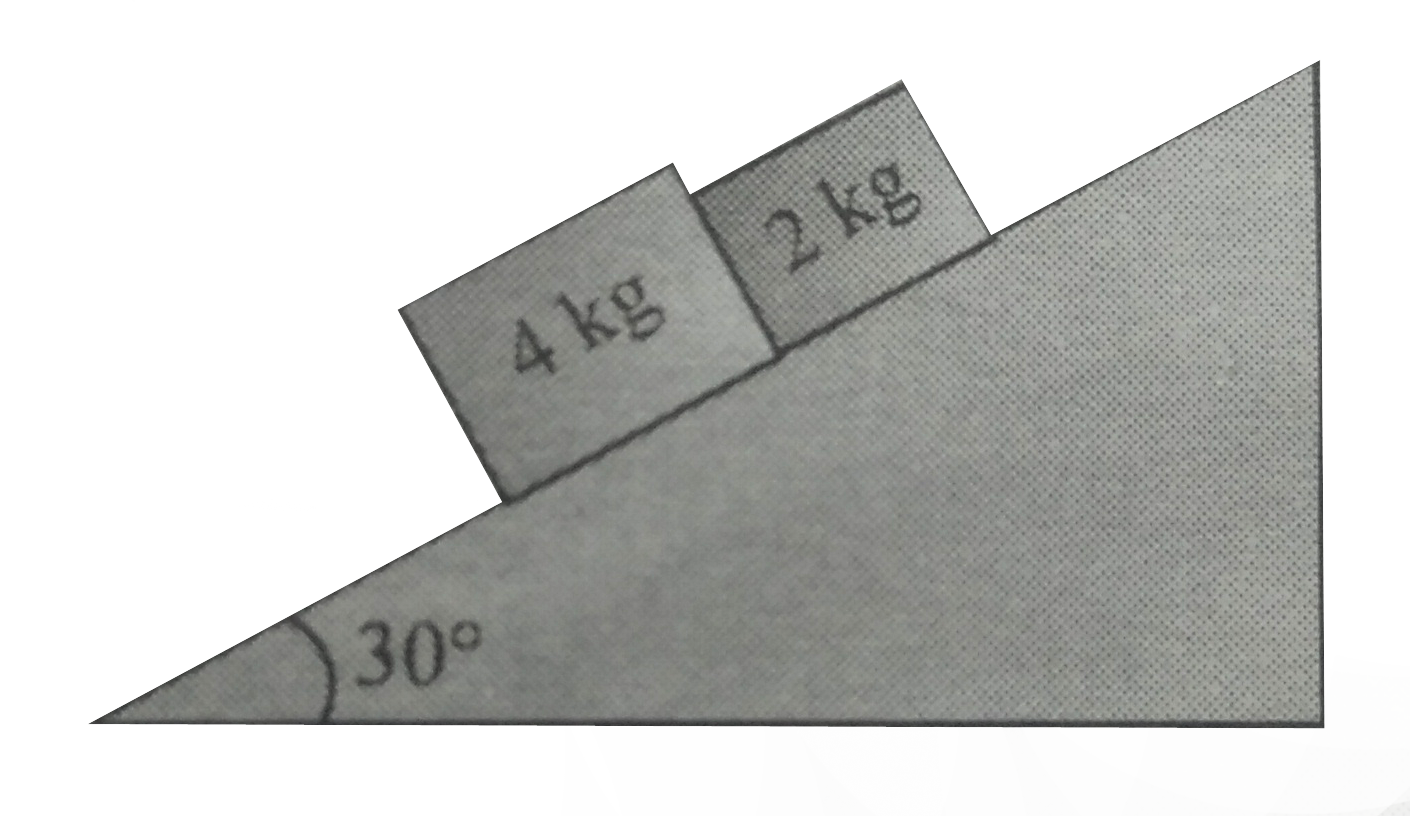 View Solution
View SolutionFigure. shows a rough incline at an angle θ with coefficient of friction μ At the bottom a spring is attached with force constant k. A block of mass m is released from a position at a length l away from the spring. Write down the work energy equations to find the maximum compression in the spring and the distance by which the block rebound up the inclined plane. the distance by which the block rebound up the inclined plane
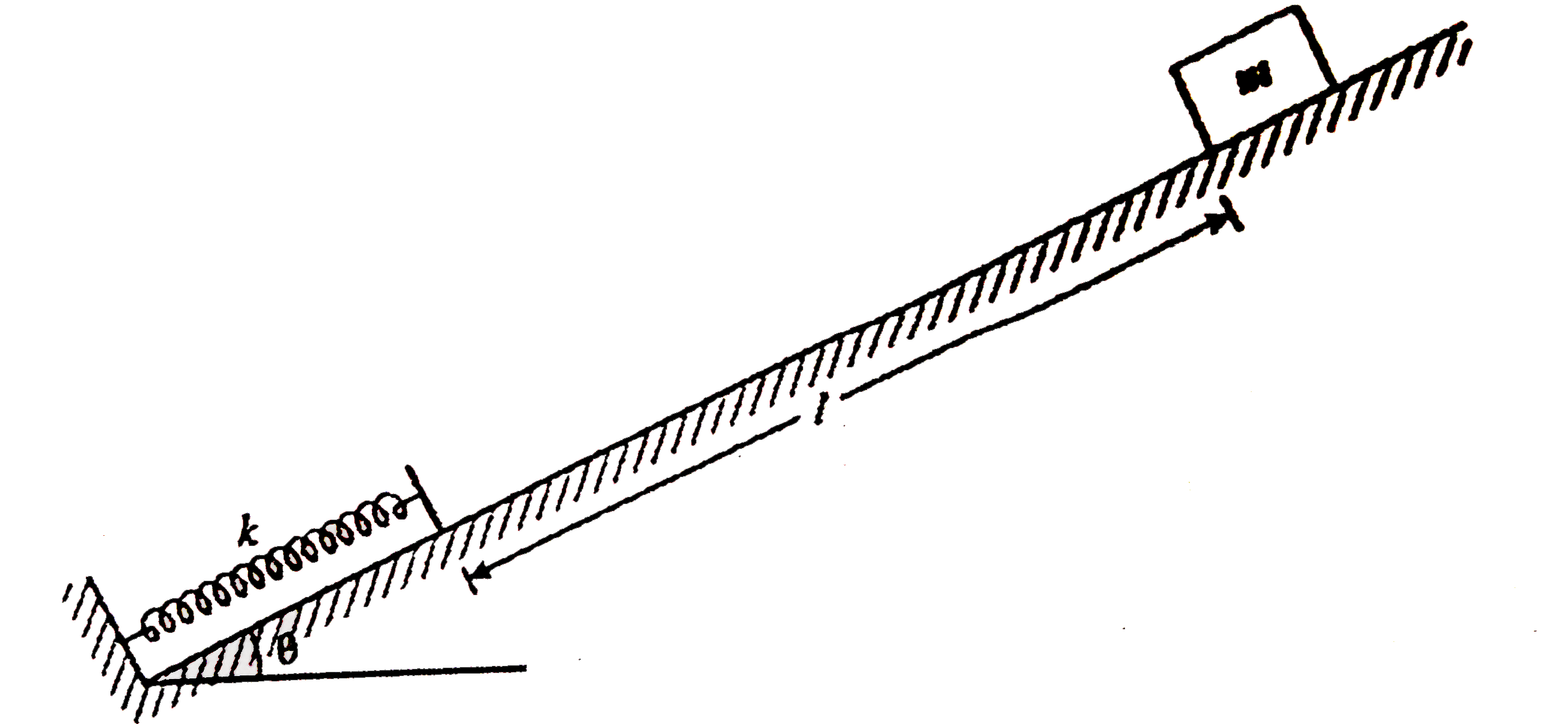 View Solution
View SolutionA block of mass 2.0 kg is pushed down an inclined plane of inclination 370 with a force of 20N cting parallelto the incline. It is found tht the block moves on the incline with an acceleration of 10ms2. If the blcok started from rest, find the work done a. by the applied force in the first second b. by the weight of the block in the first second and c. by the frictional force acting on the block in the first second. Take g=10ms2.
View SolutionA block of mass m compresses a spring iof stifffness k through a distacne l/2 as shown in the figure .If the block is not fixed to the spring the period of motion of the block is
 View Solution
View SolutionA block with mass 0.50kg is forced against a horizontal spring of negligible mass , compressing the spring a distance of 0.20m (figure). When released, the block moves on a horizontal table top for 1.00N/m. What is the coefficient of kinetic friction μk, between the block and the block and the table?
 View Solution
View Solution

