Kinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
is constant
is increasing
is decreasing
first increase and then decreases
The correct Answer is:A
To find the force acting on a particle whose kinetic energy varies with time as K=4t2, we can follow these steps:
Step 1: Relate Kinetic Energy to Velocity
The kinetic energy K of a particle is given by the formula:
K=12mv2
where m is the mass of the particle and v is its velocity.
Step 2: Set Up the Equation
From the problem, we know:
K=4t2
Equating the two expressions for kinetic energy, we have:
12mv2=4t2
Step 3: Solve for Velocity
Rearranging the equation to solve for v2:
mv2=8t2⟹v2=8t2m
Taking the square root to find v:
v=√8t2m=2√2t√m
Step 4: Differentiate Velocity to Find Acceleration
To find the force, we first need to determine the acceleration a. Acceleration is the derivative of velocity with respect to time:
a=dvdt
Differentiating v:
v=2√2√mt
Thus,
a=ddt(2√2√mt)=2√2√m
This shows that the acceleration is constant.
Step 5: Calculate the Force
Using Newton's second law, the force F acting on the particle is given by:
F=ma
Substituting the expression for acceleration:
F=m⋅2√2√m=2√2m
Conclusion
The force acting on the particle is:
F=2√2m
This force is constant since both m and √2 are constants.
---
The kinetic energy K of a particle is given by the formula:
K=12mv2
where m is the mass of the particle and v is its velocity.
From the problem, we know:
K=4t2
Equating the two expressions for kinetic energy, we have:
12mv2=4t2
Rearranging the equation to solve for v2:
mv2=8t2⟹v2=8t2m
Taking the square root to find v:
v=√8t2m=2√2t√m
To find the force, we first need to determine the acceleration a. Acceleration is the derivative of velocity with respect to time:
a=dvdt
Differentiating v:
v=2√2√mt
Thus,
a=ddt(2√2√mt)=2√2√m
This shows that the acceleration is constant.
Using Newton's second law, the force F acting on the particle is given by:
F=ma
Substituting the expression for acceleration:
F=m⋅2√2√m=2√2m
The force acting on the particle is:
F=2√2m
This force is constant since both m and √2 are constants.
Topper's Solved these Questions
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level- III (C.W)
50 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - NCERT (Based Questions)
45 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level- I (C.W)
60 Videos
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE IV
43 Videos
Similar Questions
Velocity of a particle moving in a straight line varies with its displacement as v=(√4+4s)m/s. Displacement of particle at time t=0 is s=0. Find displacement of particle at time t=2s.
View SolutionThe kinetic energy of a body moving along a straight line varies with time as shown in figure. The force acting on the body:
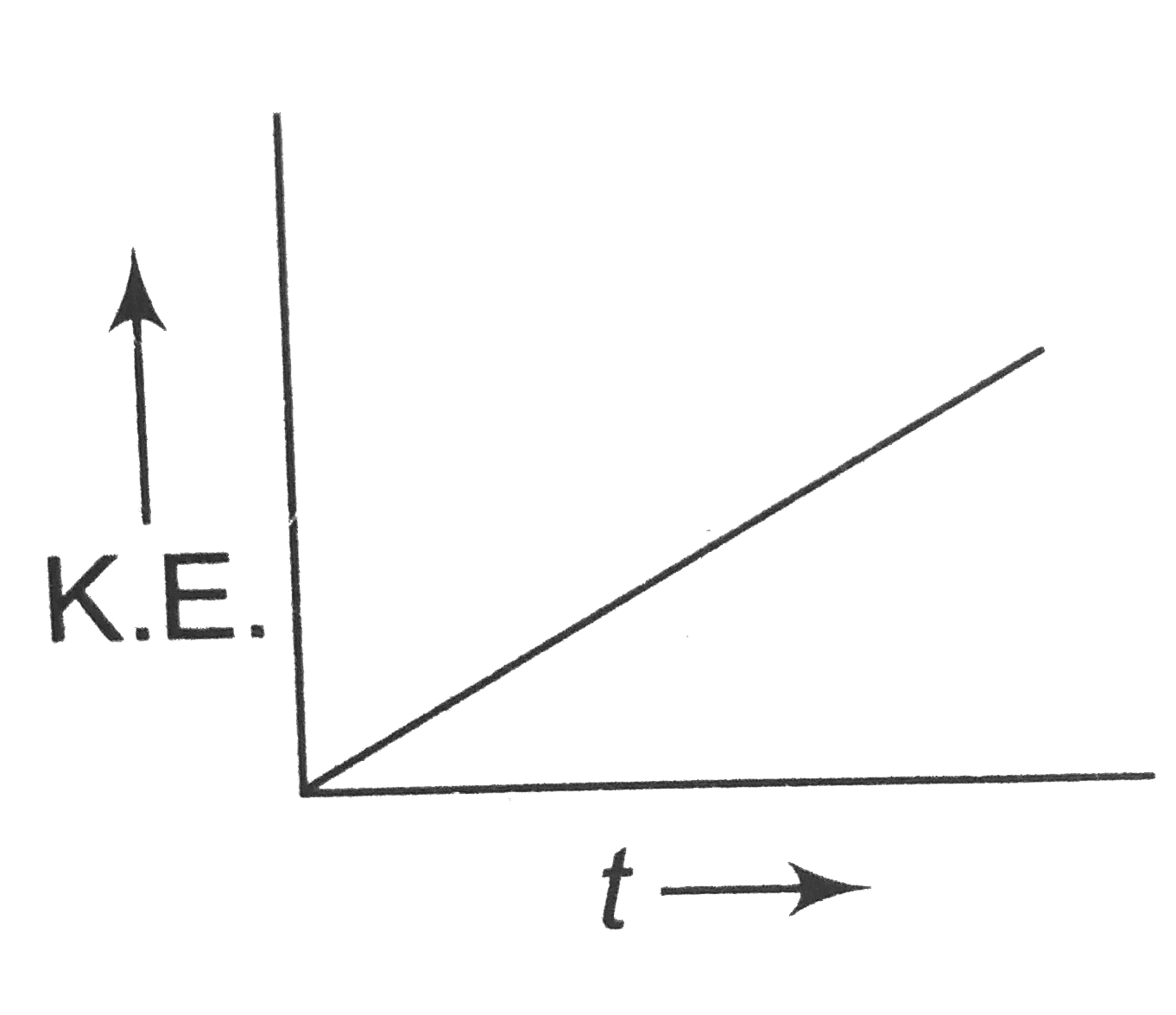
View Solution
Knowledge Check
The kinetic energy K of a particle moving in a straight line depends on the distances as K=as2. The force acting on the particle is
(where a is a constant)A2asBasC2aD√as2The displacement of a particle of mass 2kg moving in a straight line varies with times as x=(2t3+2)m. Impulse of the force acting on the particle over a time interval between t = 0 and t = 1 s is
A10 NsB12 NsC8 NsD6 NsKinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
ADirectly proportional to the speed of the particleBInversely proportional to √tCInversely proportional to the speed of the particleDDirectly proportional to √t
The position of a particle moving on a straight line depends on time t as
x=(t+3)sin(2t)
View SolutionThe coordinates of a particle moving in XY-plane vary with time as x=4t2,y=2t. The locus of the particle is a : -
View SolutionThe kinetic energy K of a particle moving along a circle of radius R depends upon the distance s as K=as2. The force acting on the particle is
View SolutionThe coordinates of a particle moving in XY-plane very with time as x=4t2,y=2t. The locus of the particle is
View SolutionForces acting on a particle moving in a straight line varies with the velocity of the particle as F=αυ where α is constant. The work done by this force in time interval Δt is :
View Solution
