A boat moves relative to water with a velocity $v$ which is $n$ times less than the river flow velocity $u$ . At what angle to the stream direction must the boat move to minimize drifting ?

A

$θ = {sin}^{- 1} (\frac{v}{u})$ from normal direction

B

$θ = {cos}^{- 1} (\frac{v}{u})$ from normal direction

C

$θ = {tan}^{- 1} (\frac{v}{u})$ from normal direction

D

$θ = {sin}^{- 1} (\frac{u}{v})$ from normal direction

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

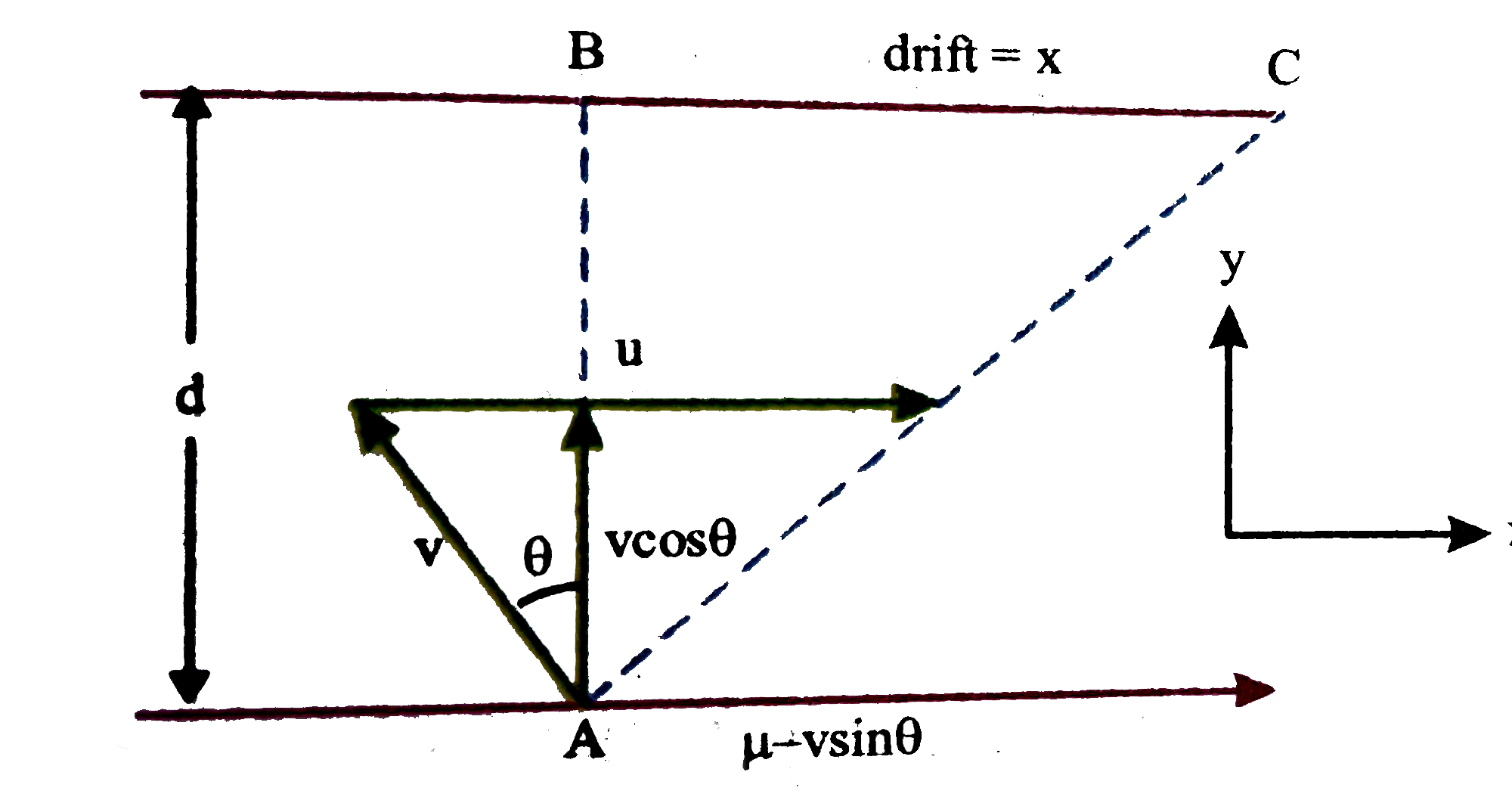
In this problem, one think should be carefully meted that the velocity of boat is less than the river flow velocity.In such a case, boat cannot reach the point directly opposite to its starting point, i.e. drift can never be zero.Thus, to minimize the drift, boat starts at an angle $θ$ from the normal direction up stream as shown Now , again if we find the components of velocity of boat along and perpendicular to the flow, these are, velocity along the river, $v_{x} = u - v sin θ$ .
take taken to cross the river is $t = \frac{d}{v_{y}} = \frac{d}{v cos θ}$ or $x = \frac{u d}{v} sec θ - d tan θ$
The drift $x$ is minimum, when $\frac{d x}{d θ} = 0$ , or $(\frac{u d}{v}) (sec θ . tan θ) - d {sec}^{2} θ = 0$
or $\frac{u}{v} sin θ = 1 or sin θ = \frac{v}{u} = \frac{1}{n} (a s v = \frac{u}{v})$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A boat moves relative to water with a velocity which is $n = 2.0$ times less than the river flow velocity. At what angle to the stream direction must the boat move to minimize drifting?
A $π / 2$
B ${sin}^{- 1} (1 / n)$
C $\frac{π}{2} + {sin}^{- 1} (1 / n)$
D $\frac{π}{2} - {sin}^{- 1} (1 / n)$
Question 2 - Select One
A boat moves relative to water with a velocity v and river is flowing with 2v. At what angle the boat shall move with the stream to have minimum drift?
A $30^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $120^{\circ}$
Question 3 - Select One
A boat crossing a river moves with a velocity v relative to still water. The river is flowing with a velocity v/2 with respect to the bank. The angle with respect to the flow direction with which the boat should move to minimize the drift is–
A $30^{\circ}$
B $60^{\circ}$
C $150^{\circ}$
D $120^{\circ}$

NARAYNA-MOTION IN A PLANE-Level -V Single answer

Home
Ask Doubt
Profile