A sequence is an AP if the sum of its first n terms is of the form An2+Bn where A and B are constants independent of n .In such a case the common difference is 2A
Step by step video & image solution for A sequence is an AP if the sum of its first n terms is of the form An^2+Bn where A and B are constants independent of n .In such a case the common difference is 2A by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Prove that a sequence in an A.P., if the sum of its n terms is of the form An2+Bn,whereA,B are constants.
View SolutionState 'T' for true and 'F' for false .
I. A sequence is an A.P ., if and only if the sum of its n terms is of the form An2+Bn , where A and B are constants .
II. If 18, a, b , −3 are in A.P., then a+b=15 .
III If a,c,b are in A.P., then 2c=a+b .
IV .The nth term from the end of an A.P. is the (m−n+1)th term from the beginning , where m terms are in A.P.
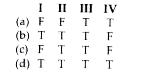
View SolutionIn an AP, the sum of first n terms is n2+2n. Find its 18th term.
View SolutionIf the sum of n terms of an A.P. is (pn+qn2), where p and q are constants, find the common difference.
View SolutionSum of first n terms of an A.P. is of the form An2+Bn i.e. aquadratic expression in n, in such case the common differenceis twice the coefficient of n2. i.e. 2A
View SolutionProve that a sequence is an AP , if its nth term is linear expressions in n i.e. an=An+B where A and B are constants .In such a case the cofficients of n is an is the common difference of AP.
View SolutionIf nth term of A.P is 2n+3, then common difference is
View SolutionIf the sum of n terms of an A.P. is (pn+qn2), where p and q are constants, find the common difference.
View SolutionIf the sum of n terms of an A.P. is nP+12n(n−1)Q,where P and Q are constants, find the common difference.
View SolutionIf the sum of n terms of an AP is Pn+Qn2, where P,Q are constants, then its common differc
View Solution
