A ball A of mass m attached to a string of length L is released when the string is horizontal. It strikes another ball B of mass 2m suspended to another string of length L at rest as shown. Find the maximum angle made by the string if the collision is completely inelastic.
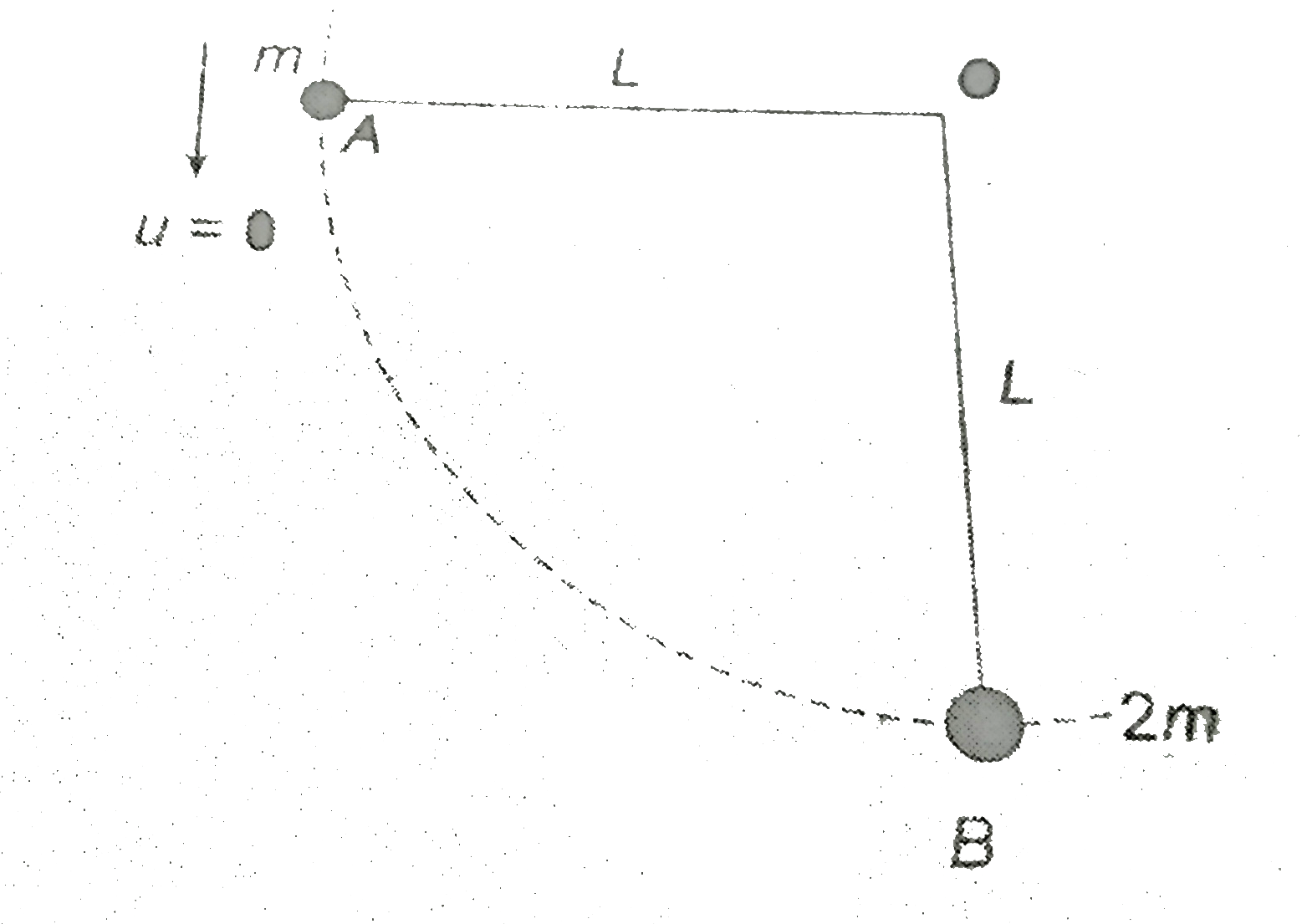
when ball A reaches to ball B , its velocity
u=√2gL
By the momentum conservation
m√2gL+2m×0=(m+2m)V⇒V=13√2gL
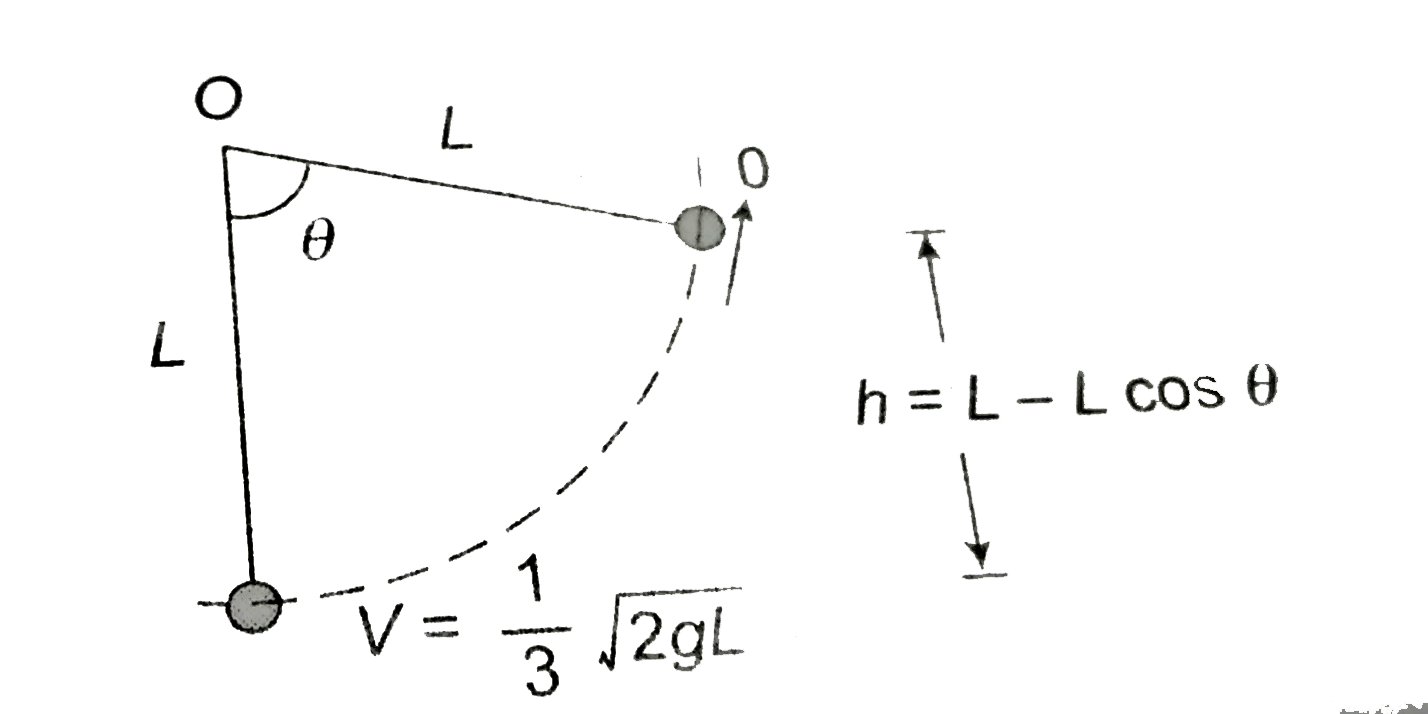
Since V∝√2gL , the combined mass will oscillate like a simple pendulum , hence , the maximum angle made by the string with vertical <90∘.
0=V2−2gh
0=192gL−2gL(1−cosθ)
1−cosθ=19⇒cosθ=89⇒θ=cos6(−1)(89)

Topper's Solved these Questions
Similar Questions
In the previous problem , the block of massM is attached to a string of length L. Find the maximum angle made by the string with vertical . Take veloctiy of buller u=4√gL. Take M=3m.
View SolutionA ball is attached to a string of length L. The ball is released when the string is horizontal. The ball collides with wall and e=2/√5. After how many minimum number of collisions , the amplitude of oscillation becomes less than 60∘.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 2m is attached to a string of length L=5m. The block is released when the string is horizontal , it picks up a particle of 2m kept at rest at the lowest point. The maximum height attached by the combined mass is
A1mB1.25mC1.5mD2.0m - Question 2 - Select One
A bob of mass m attached with a string of length l tied to a point on ceiling is released from a position when its string is horizontal. At the bottom most point of its motion, an identical mass m gently stuck to it. Find the maximum angle from the vertical to which it rotates.
A(a) cos−1(23)B(b) cos−1(34)C(c) cos−1(14)D(d) 60∘ - Question 3 - Select One
A particle of mass m is tied to a string of length L and whirled into a horizontal plan. If tension in the string is T then the speed of the particle will be :
A√TlmB√2TlmC√3TlmD√Tml
The minimum velocity required to complete a full circle in a vertical plane is given to a bob of mass m suspended by a string of length 25 cm. When it reaches the highest point, it collides elastically with another bob of mass m suspended by a string of length l2, which is initially at rest. After the collision, if the second bob acquires the minimum speed required to complete a full circle in the vertical plane, then the length of the string l2 __m. (Both the strings are massless and in-extensible)
View SolutionA small ball of mass 'm' is connected by an intextensible mass less string of length ('l'=10m) with an another ball of mass M=4m. They are released with zero tension in the string from a height h(h=5m) as shown. Find the time when the string becomes taut for the first time after the mass 'M' collides with the ground is _____S. (Take all collisions to be elastic) (g=10m/s2)
 View Solution
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
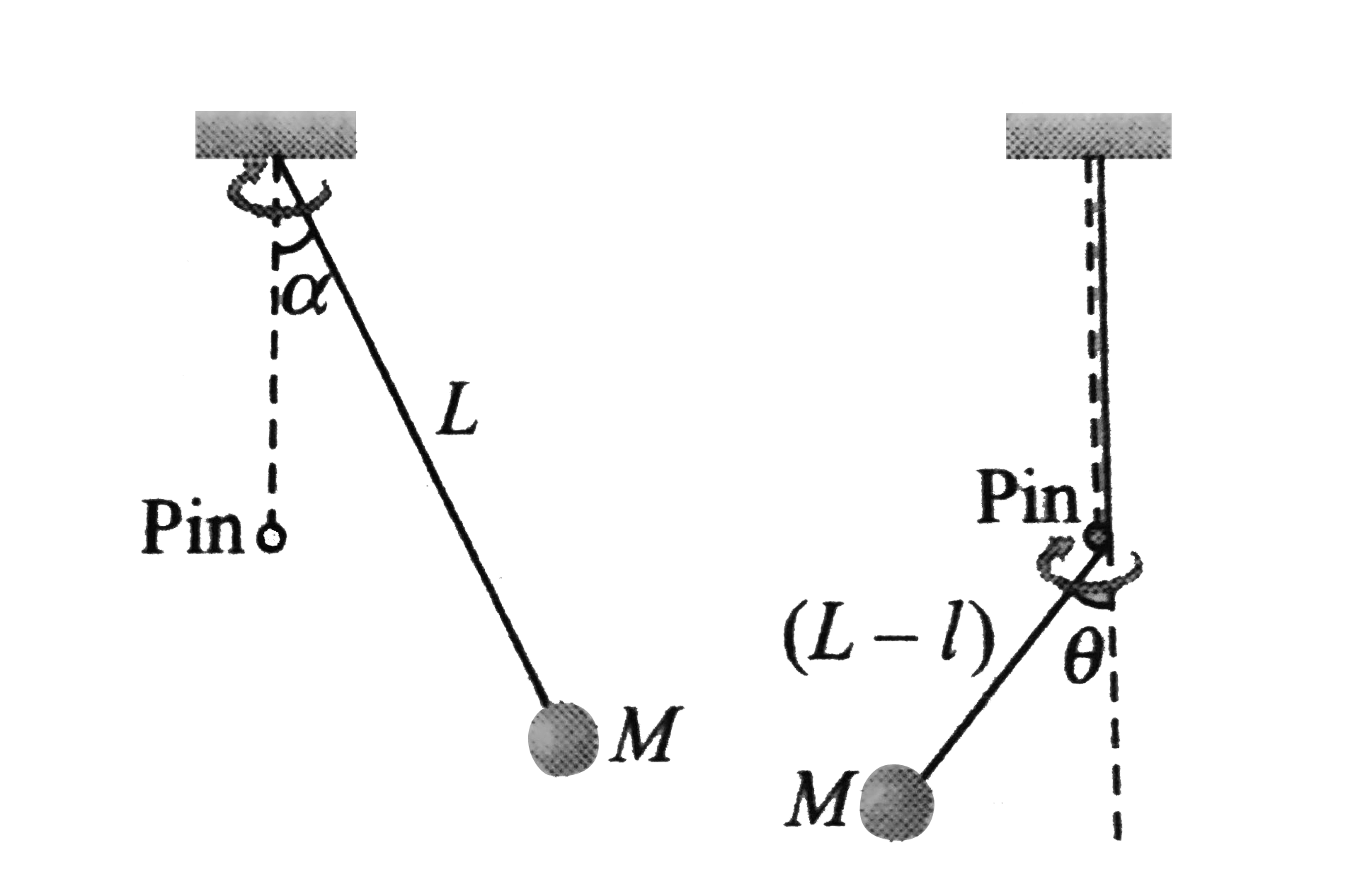 View Solution
View SolutionA stone of mass m is tied to a string of length l and rotated in a circle with a constant speed v . If the string is released, the stone flies
View SolutionA uniform rod of length 3L and mass m is suspended from a horizontal roof by two strings of length L and 2L as shown. Then the tension in the left string of length L is :
 View Solution
View Solution

