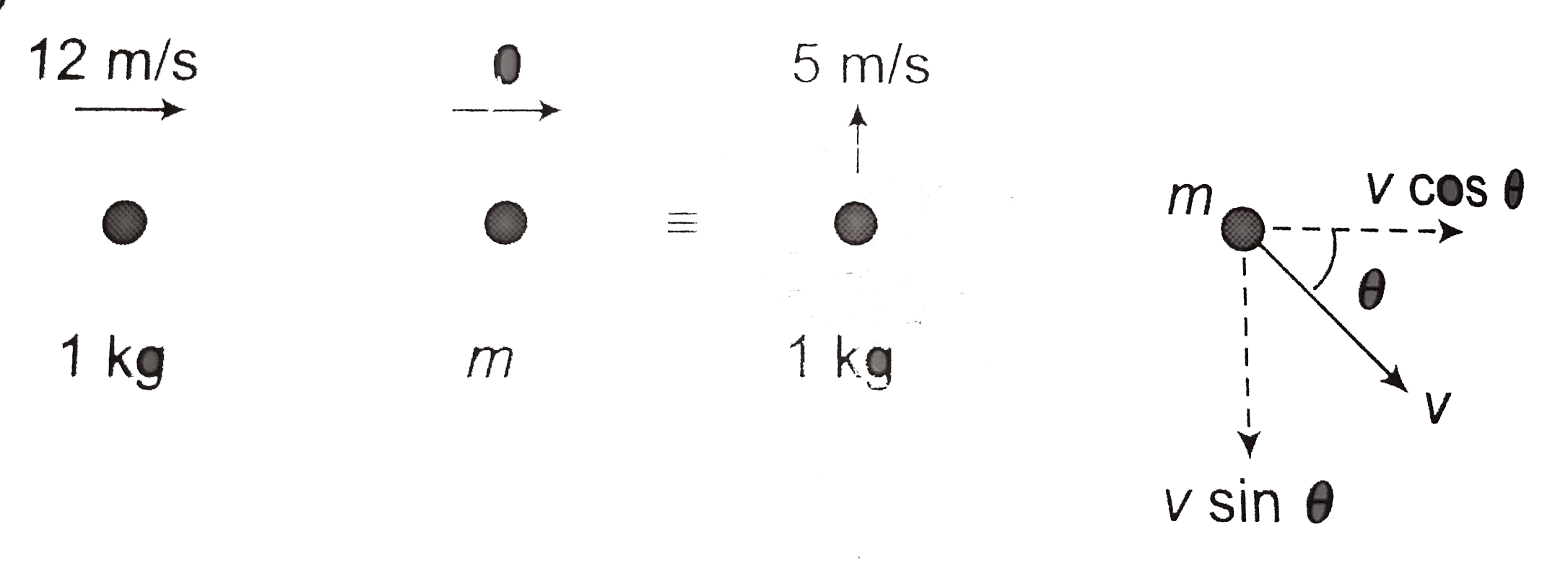
A ball of mass , moving with a speed of , collides obliquely and elasticity with another ball which was initially at rest. Ball then moves off at the right angle to its initial direction with a speed of . The momentum of ball after the collision is
The correct Answer is:C
Momentum conservation :
-direction :
-direction :
Topper's Solved these Questions
Similar Questions
On a frictionless surface,a block of mass M moving at speed v collides elastically with another block of same mass M which is initially at rest.After collision the first block moves at an angle θ to its initial direction and has a speed v3 .The second block's speed after the collision is ?
View SolutionA ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View Solution
Knowledge Check
On a frictionless surface, a block of mass M moving at speed v collides elastically with another block of same mass M which is initially at rest. After collision the first block moves at an angle θ to its initial direction and has a speed v3. The second block.s speed after the collision is
A3√2vB√32vC2√23vD34vOn a friction surface a block a mass M moving at speed v collides elastic with another block of same mass M which is initially at rest . After collision the first block moves at an angle θ to its initial direction and has a speed v3. The second block's speed after the collision is
A√32vB2√23vC34vD3√2vA ball of mass 1 kg moving with a velocity of 0.4 ms−1 collides with another stationary ball. After the collision, the first ball moves with a velocity of 0.3ms1 in a direction making an angle of 90∘ with its initial direction. The momentum of the second ball after the collision will be (in kg ms−1)
A0.1B0.3C0.5D0.7
A ball of mass 1kg moving with a velocity of 15m/s undergoes a head on elastic collision with another ball of unknown mass at rest, and rebound with a velocity of 10m/s. What is the mass of the other ball?
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View SolutionA ball of mass m1 moving with a certain speed collides elastically with another ball of mass m2 kept at rest . If the first ball starts moving in reversed direction after the impact then
View SolutionA ball of mass 5 kg travelling with velocity of 15 cm/s makes a head on collision with another ball of mass 1 kg which is at rest. After the collision, the speed of the lighter ball is
View SolutionA ball of mass M moving with a velocity v collides perfectly inelastically with another ball of same mass but moving with a velocity v in the opposite direction. After collision
View Solution
