A staright wire of length $(π^{2})$ meter is carrying a current of $2 A$ and the magnetic field due to it is measured at a point distant $1 c m$ from it. If the wire is to be bent into a circles and is to carry the same current as before, the ratio of the magnetic field at its centre to that obtained in the first case would be

A

$50 : 1$

B

$: 50$

C

$100 : 1$

D

$1 : 100$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

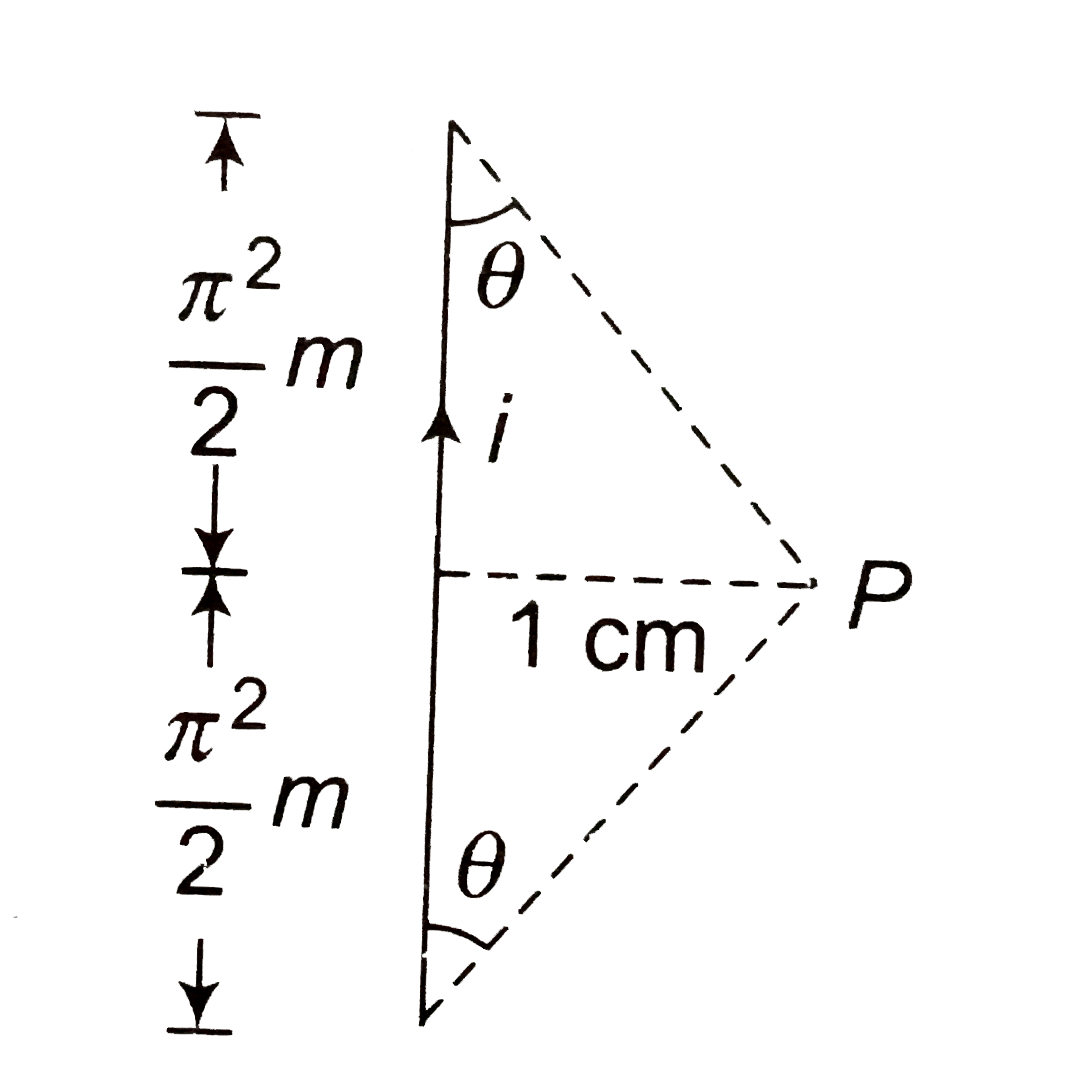
$B_{P} = \frac{μ_{0} i}{4 π d} (cos θ + cos θ) = \frac{μ_{0} i cos θ}{2 π d}$
$= \frac{μ_{0} i}{2 π \times 1 \times 10^{2}} \frac{π^{2} / 2}{\sqrt{{(π^{2} / 2)}^{2} + {(0.01)}^{2}}} = \frac{μ_{0} i}{2 π \times 10^{- 2}}$

$2 π r = L = π^{2} \Rightarrow r = \frac{π}{2} m$
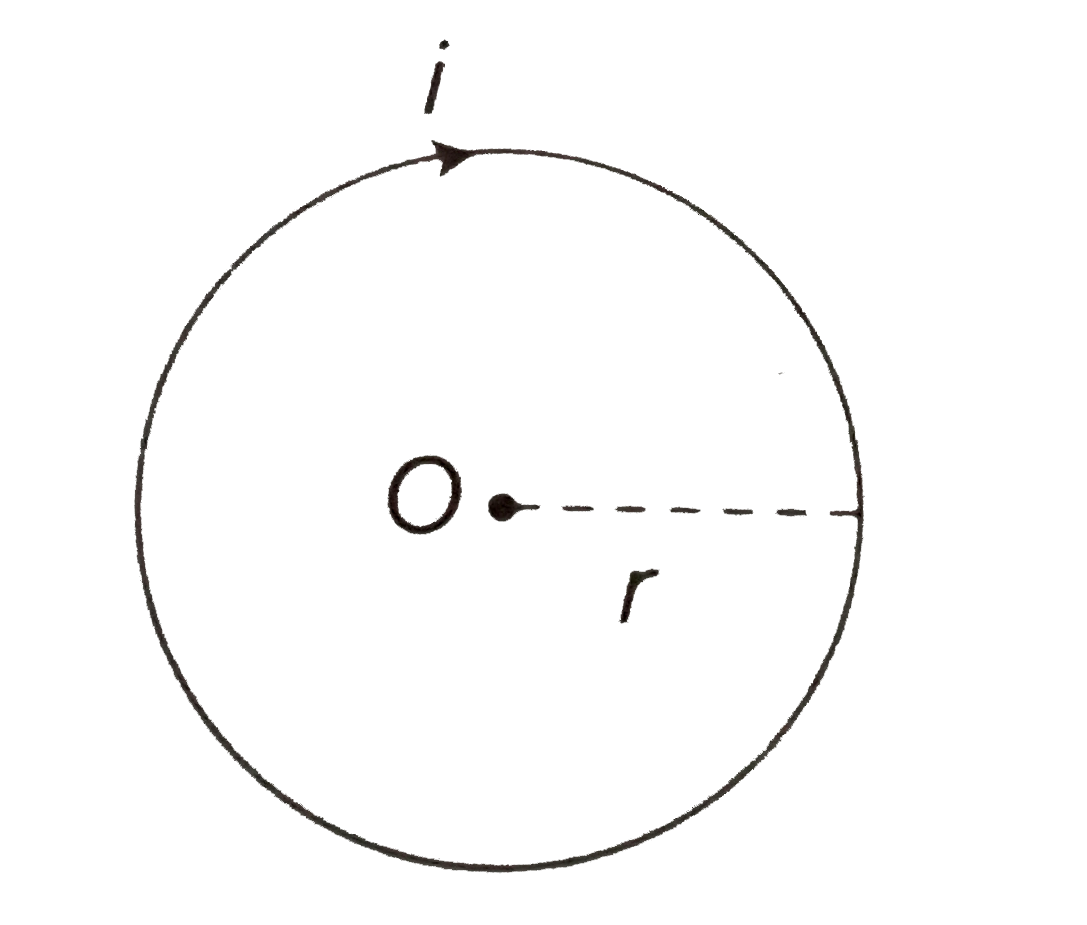
$B_{0} = \frac{μ_{0} i}{2 r} = \frac{μ_{0} i}{2 . \frac{π}{2}} = \frac{μ_{0} i}{π}$
$\frac{B_{0}}{B_{r}} = 2 \times 10^{- 2} = \frac{2}{100} = \frac{1}{50}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A straight wire of length $(2 π^{2})$ metre is carrying a current of $2 A$ and the magnetic field due to it is measured at a point distant $1 c m$ from it. If the wire is to be bent into a circle and is to carry the same current as before, the ratio of the magnetic field at its centre to that obtained in the first case would be
A $50 : 1$
B $1 : 50$
C $100 : 1$
D $1 : 100$
Question 2 - Select One
For the current carrying wire, show that the magnetic field at P is

A $\frac{μ_{0} i}{4 π} (\frac{3 π}{2 R} + \frac{\sqrt{2}}{d}) ⊙$
B $\frac{μ_{0} i}{4 π} (\frac{3 π}{2 R} - \frac{\sqrt{2}}{d}) \otimes$
C $\frac{μ_{0} i}{4 π} (\frac{3 π}{2 R} - \frac{\sqrt{2}}{d}) ⊙$
D $\frac{μ_{0} i}{4 π} (\frac{3 π}{2 R} + \frac{\sqrt{2}}{d}) \otimes$
Question 3 - Select One
A wire of length L carrying a current I is bent into a circle. The magnitude of the magnetic field at the centre of the circle is
A $\frac{π μ_{0} I}{L}$
B $\frac{μ_{0} I}{2 L}$
C $\frac{2 π μ_{0} I}{L}$
D $\frac{μ_{0} I}{2 π L}$

CP SINGH-MAGNETIC FIELD-EXERCISE

Home
Ask Doubt
Profile