Two concentric coils carry the same current in opposite directions. The diameter of the inner coil is equal currents. The outer coil. If the magnetic field produced by the outer coil at the common centre are 1T, the net field at the centre is
1T
2T
3T
4T
The correct Answer is:A

B1=μ0i2r,⊗
B2=μ0i2.2r=μ0i4r,⊙
μ0i4r=1T
B0=B1−B2=μ0i4r=1T
Topper's Solved these Questions
Similar Questions
A current carrying wire is bent into the shape of a square coil. The magnetic field produced at the entre of coil by one arm BC is B. Then the resultant magnetic field at the centre due to all the arms will be-
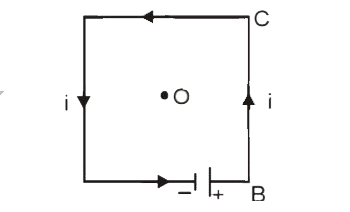 View Solution
View SolutionMagnetic field produced by current carrying coil depends upon the number of turns in the coil.
View Solution
Knowledge Check
- Question 1 - Select One
The magnetic field at the centre of current carrying coil is
Aμ0ni2rBμ02πnirCμ0ni4rDμ0ni - Question 2 - Select One
Two identical coils have a common centre and their planes are at right angles to each other. They carry equal currents. If the magnitude of the magnetic field at the centre due to one of the coils is B then that due to the combination is
ABB√2BCB√2D2B - Question 3 - Select One
At what distance on the axis, from the centre for circular current carrying coil of radius r, the magnetic field becomes 1/8 th of the magnetic field at centre?
A√2rB23/2rC√3rD3√2r
Draw the pattern of magnetic field lines around a current carrying strainght conductor. How does the strength of the magnetic field produced change.
(i) with the distance from the conductor ?
(ii) with increase in current in a conductor ?View SolutionA circular coil of radius R carries a curretn. Find an expression for the magnetic field due to this coil at its centre. Also find the direction of field.
View SolutionIn the net work shown in figure,find the magnetic field at the centre O of the coil.
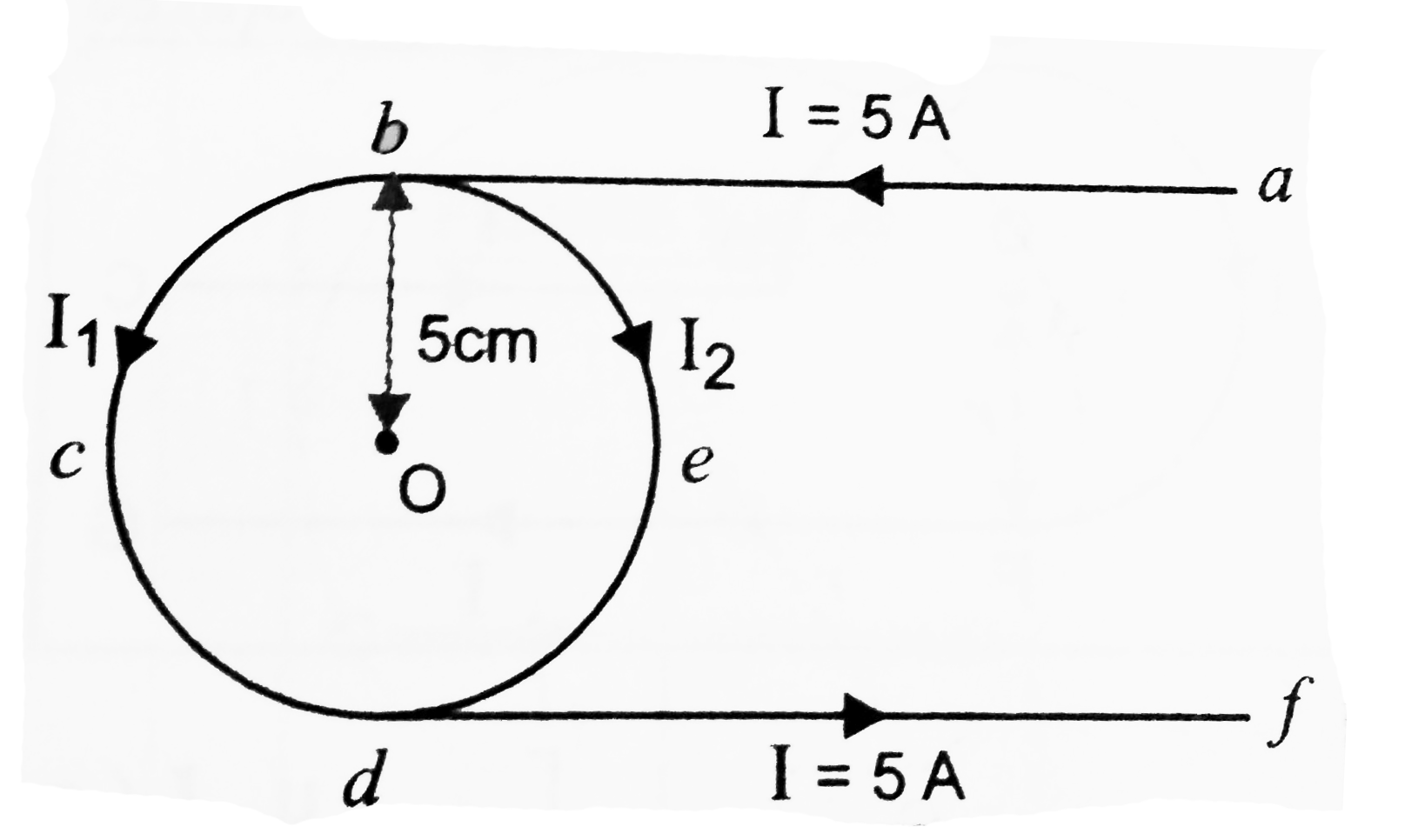 View Solution
View SolutionThe magnetic field at the centre of the current carrying coil
View SolutionA current I is flowing in a hexgonal coil of side a figure. The magnetic field induction at the centre O of the coil will be
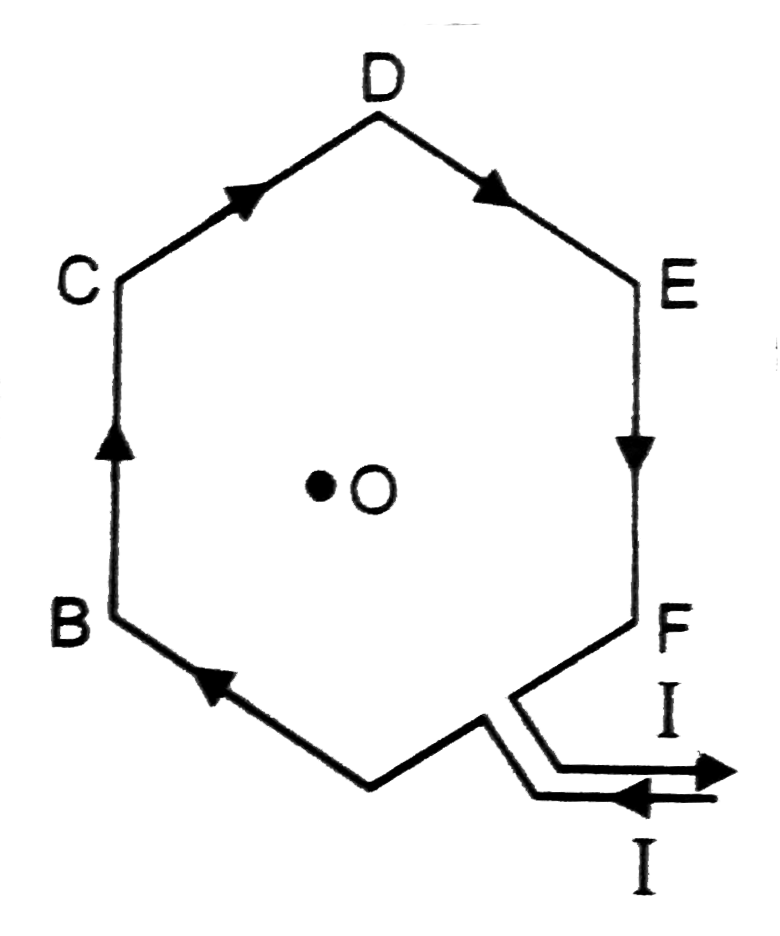 View Solution
View Solution

