Charges +q−4q and +2q are arranged at the corners of an equilatoral triangle of side 0.15m.If the q=1μC their mutual potential energy is
0.4J
0.5J
0.6J
0.8J
The correct Answer is:3
To find the mutual potential energy of the charges arranged at the corners of an equilateral triangle, we can follow these steps:
1. Identify the Charges and Their Values:
- Let the charges be:
- q1=+q=+1μC=+1×10−6C
- q2=−4q=−4×1μC=−4×10−6C
- q3=+2q=+2×1μC=+2×10−6C
2. Determine the Side Length of the Triangle:
- The side length L of the equilateral triangle is given as 0.15m.
3. Use the Formula for Potential Energy Between Two Point Charges:
- The potential energy U between two point charges qi and qj separated by a distance r is given by:
Uij=kqiqjr
- Where k=9×109Nm2/C2 is Coulomb's constant.
4. Calculate the Potential Energy for Each Pair of Charges:
- For q1 and q2:
U12=kq1q2L=9×109(1×10−6)(−4×10−6)0.15
U12=9×109−4×10−120.15=−240×10−3=−0.24J
- For q1 and q3:
U13=kq1q3L=9×109(1×10−6)(2×10−6)0.15
U13=9×1092×10−120.15=120×10−3=0.12J
- For q2 and q3:
U23=kq2q3L=9×109(−4×10−6)(2×10−6)0.15
U23=9×109−8×10−120.15=−480×10−3=−0.48J
5. Calculate the Total Mutual Potential Energy:
- The total potential energy U is the sum of the potential energies of each pair:
U=U12+U13+U23
U=(−0.24)+(0.12)+(−0.48)=−0.60J
Final Answer:
The mutual potential energy of the three charges is U=−0.60J.
- Let the charges be:
- q1=+q=+1μC=+1×10−6C
- q2=−4q=−4×1μC=−4×10−6C
- q3=+2q=+2×1μC=+2×10−6C
- The side length L of the equilateral triangle is given as 0.15m.
- The potential energy U between two point charges qi and qj separated by a distance r is given by:
Uij=kqiqjr
- Where k=9×109Nm2/C2 is Coulomb's constant.
- For q1 and q2:
U12=kq1q2L=9×109(1×10−6)(−4×10−6)0.15
U12=9×109−4×10−120.15=−240×10−3=−0.24J
U13=kq1q3L=9×109(1×10−6)(2×10−6)0.15
U13=9×1092×10−120.15=120×10−3=0.12J
U23=kq2q3L=9×109(−4×10−6)(2×10−6)0.15
U23=9×109−8×10−120.15=−480×10−3=−0.48J
- The total potential energy U is the sum of the potential energies of each pair:
U=U12+U13+U23
U=(−0.24)+(0.12)+(−0.48)=−0.60J
The mutual potential energy of the three charges is U=−0.60J.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Level II(C.W)27videos
ELECTROSTATICS AND GAUSS LAW
- BOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Level III (C.W)55videos
ELECTROSTATICS AND GAUSS LAW
- BOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - C.U.Q133videos
ELECTROSTATICS AND GAUSS LAW
- BOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - ADDITIONAL PROBLEMS14videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Comprehension type6videos
EXPERIMENTAL PHYSICS
Similar Questions
Three charges +q,+qand−q are located at the corners of an equilaterial triangle of side a. Calculate potential energy of the system.
View SolutionThree charges +q,2qand−4q are placed on the three vertices of an equale-laterail triangle of each side0⋅1m. Calculate electrostatic potential energy of the system, take q=10−7C
View Solution
Knowledge Check
- Question 1 - Select One
Three charges each20μC are placed at the corners of an equilateral triangle of side 0.4m The potential energy of the system is
A18×10−6JB9JC9×10−6JD27J - Question 2 - Select One
Three point charges of +2q,+2qand−4q are placed at the corners A, B and C of an equilateral triangle ABC of side x. The magnitude of the electric dipole moment of this system is
A2 qxB3√2qxC3 qxD2√3qx - Question 3 - Select One
Three point charges of +2q,+2qand−4q are placed at the corners A, B and C of an equilateral triangle ABC of side x. The magnitude of the electric dipole moment of this system is
A2qxB3√2qxC3qxD2√3qx
Three charges -q +q and +q are placed at the corner of the equilateral triangle of side a as shown in the figure.The net electric potential at the centroid of the triangle will be (k=(1)/(4 pi varepsilon_(0)))
View SolutionElectric charges q,q,–2q are placed at the corners of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is
View Solution(a) Three point charges q,-4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the figure. Obtain the expression for the magnitude of the resultant electric force acting on the charge q.
(b) Find out the amount of the work done to separate the charges at infinite distance.
 View Solution
View SolutionThree charges Q,(+q) and (+q) are placed at the vertices of an equilateral triangle of side l as shown in the figure. It the net electrostatic energy of the system is zero, then Q is equal to
 View Solution
View SolutionThree charges of (+2q),(−q) and (−q) are placed at the corners A,B and C of an equilateral triangle of side a as shown in the adjoining figure. Then the dipole moment of this combination is
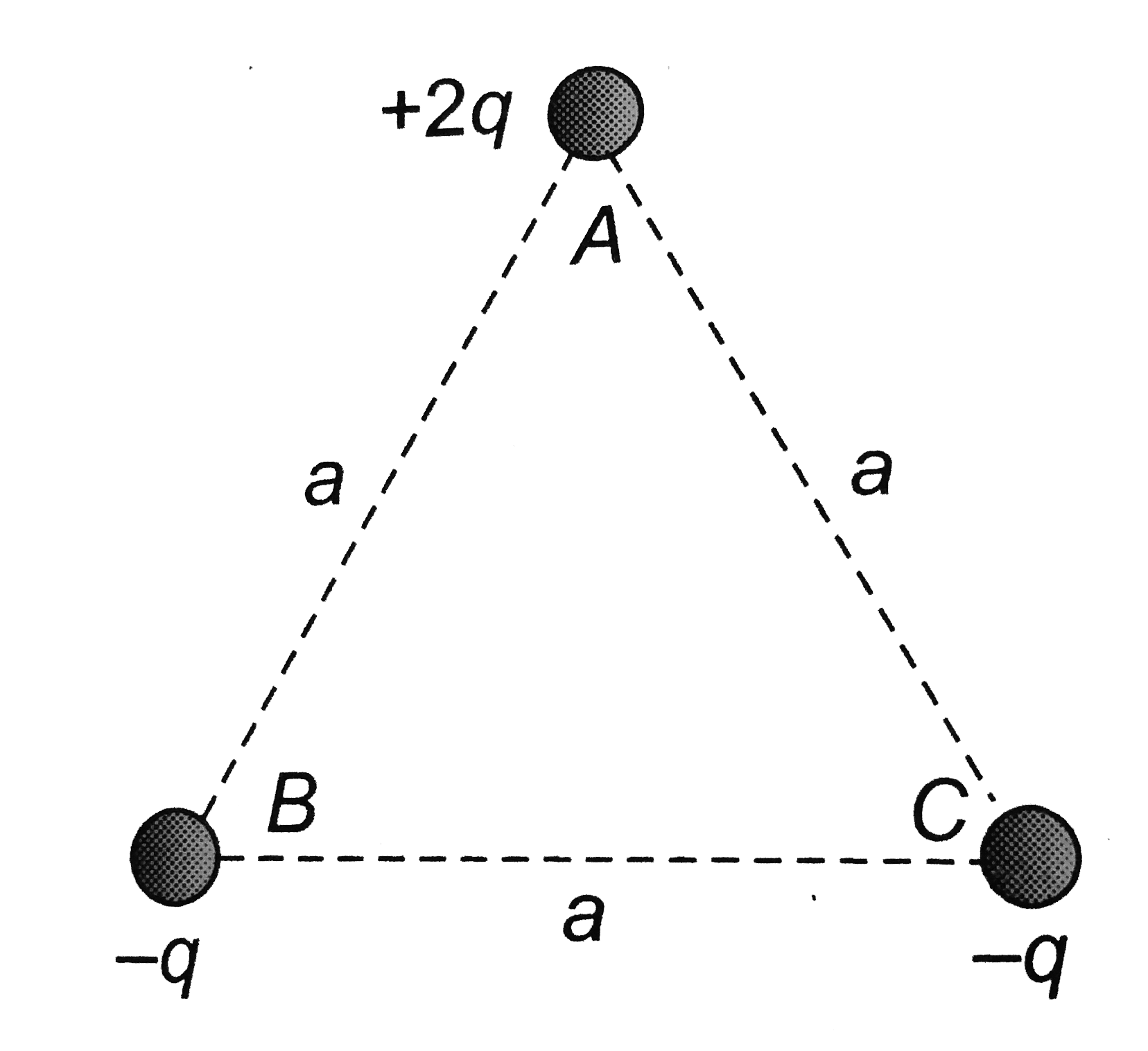 View Solution
View Solution

