A circuit draws a power of 550 watt from a source of 220 volt, 50Hz. The power factor of the circuit is 0.8 and the current lags in phase behind the potential difference. To make the power factor of the circuit as 1.0, The capacitance should be connected in series with it is
75μF
60μF
50μF
65μF
The correct Answer is:A
To solve the problem step by step, we will calculate the necessary capacitance to make the power factor of the circuit equal to 1.0.
Step 1: Calculate the impedance (Z) of the circuit
The formula for power in an AC circuit is given by:
P=Vrms⋅Irms⋅cosϕ
We can rearrange this to find the impedance:
Z=V2rmsP⋅cosϕ
Given:
- P=550W
- Vrms=220V
- cosϕ=0.8
Substituting the values:
Z=(220)2550⋅0.8
Z=48400440
Z=110Ω
Step 2: Calculate the resistance (R) of the circuit
Using the relationship between power factor, resistance, and impedance:
cosϕ=RZ
We can rearrange this to find R:
R=Z⋅cosϕ
Substituting the values:
R=110⋅0.8
R=88Ω
Step 3: Calculate the inductive reactance (X_L)
Using the impedance formula:
Z2=R2+X2L
We can rearrange this to find XL:
XL=√Z2−R2
Substituting the values:
XL=√(110)2−(88)2
XL=√12100−7744
XL=√4356
XL=66Ω
Step 4: Calculate the capacitive reactance (X_C) needed for power factor 1
At power factor 1, the inductive reactance (X_L) must equal the capacitive reactance (X_C):
XC=XL=66Ω
Step 5: Calculate the capacitance (C)
The capacitive reactance is given by:
XC=1ωC
Where ω=2πf and f=50Hz:
ω=2π⋅50=100πrad/s
Now substituting for C:
C=1ωXC
Substituting the values:
C=1100π⋅66
C=16600π
Calculating the value:
C≈120735.6≈4.82×10−5F
Converting to microfarads:
C≈48.2μF
Final Answer
To make the power factor of the circuit equal to 1.0, a capacitance of approximately 48.2 microfarads should be connected in series with it.
The formula for power in an AC circuit is given by:
P=Vrms⋅Irms⋅cosϕ
We can rearrange this to find the impedance:
Z=V2rmsP⋅cosϕ
- P=550W
- Vrms=220V
- cosϕ=0.8
Z=(220)2550⋅0.8
Z=48400440
Z=110Ω
Using the relationship between power factor, resistance, and impedance:
cosϕ=RZ
We can rearrange this to find R:
R=Z⋅cosϕ
Substituting the values:
R=110⋅0.8
R=88Ω
Using the impedance formula:
Z2=R2+X2L
We can rearrange this to find XL:
XL=√Z2−R2
Substituting the values:
XL=√(110)2−(88)2
XL=√12100−7744
XL=√4356
XL=66Ω
At power factor 1, the inductive reactance (X_L) must equal the capacitive reactance (X_C):
XC=XL=66Ω
The capacitive reactance is given by:
XC=1ωC
Where ω=2πf and f=50Hz:
ω=2π⋅50=100πrad/s
Now substituting for C:
C=1ωXC
Substituting the values:
C=1100π⋅66
C=16600π
Calculating the value:
C≈120735.6≈4.82×10−5F
Converting to microfarads:
C≈48.2μF
To make the power factor of the circuit equal to 1.0, a capacitance of approximately 48.2 microfarads should be connected in series with it.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - I (H.W)23videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - II(H.W)13videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - V83videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)14videos
ATOMIC PHYSICS
Similar Questions
A circuit draws a power of 600 W from an AC source of 200 V 50 Hz . The power factor of the circuit is 0.6 . The current lags behing the voltage . What value of capacitance should be connected in the circuit to bring its power factor to unity?
View SolutionThe power factor in LCR series circuit at resonance is
View Solution
Knowledge Check
- Question 1 - Select One
The power factor of the circuit in fig. is 1/√2. The capacitance of the circuit is equal to
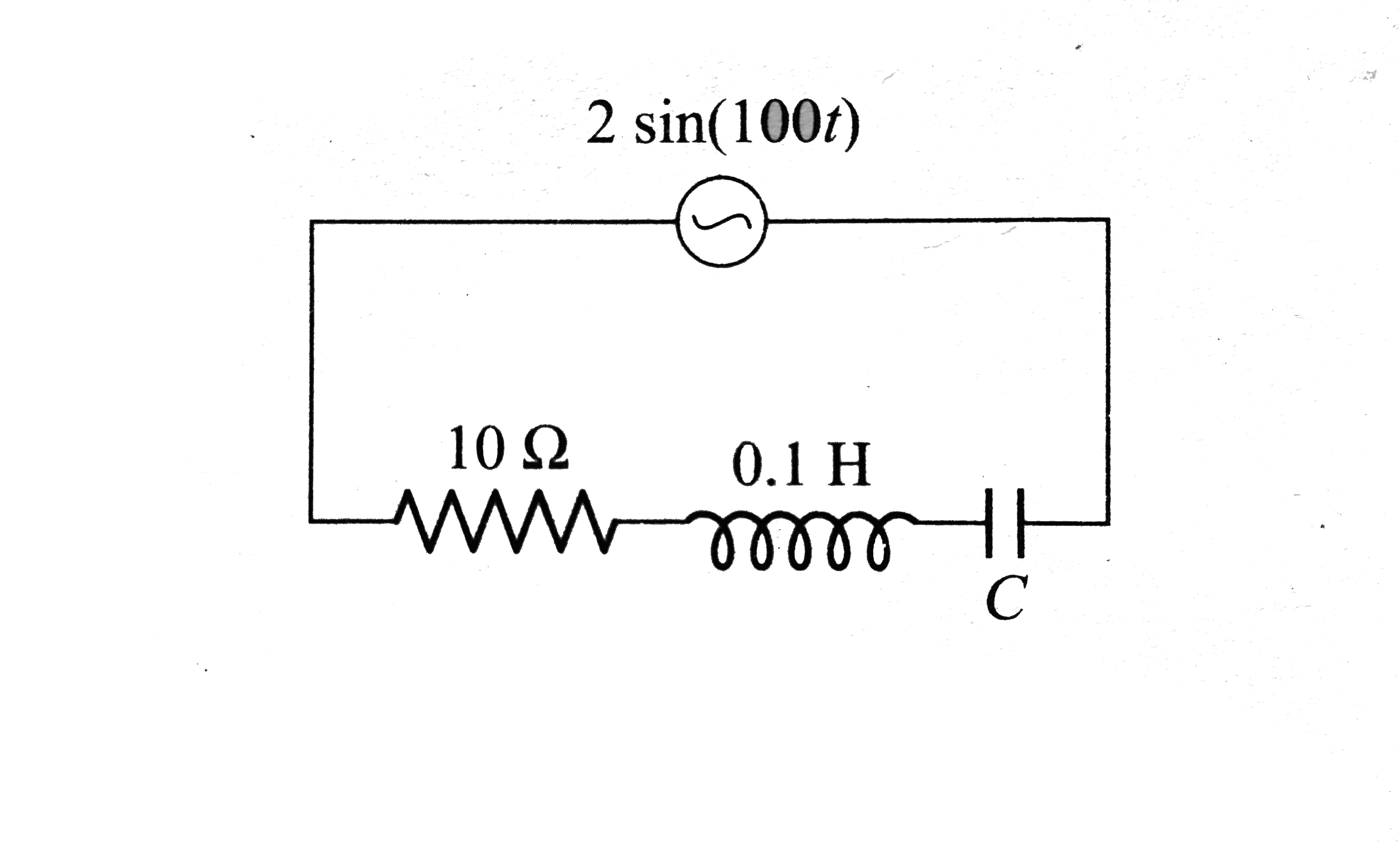 A400(μ)FB300(μ)FC500(μ)FD200(μ)F
A400(μ)FB300(μ)FC500(μ)FD200(μ)F - Question 2 - Select One
The power factor of a series LCR circuit at resonance will be
A1B0C12D1√2 - Question 3 - Select One
The power factor for a purely capacitive circuit is
A1B√2C1√2DZero
An inductive circuit draws a power 550 W from a 220V-50Hz source. The power factor of the circuit is 0.8. The current in the circuit lags behind the voltage. Show that a capacitor of about 142π×10−2F will have to be commected in the circuit to bring its power factor to unity.
View SolutionWhat is the value of power factor for series LR circuit ?
View SolutionPower factor in series LCR circuit at reasonance is
View SolutionThe power factor of a CR circuit is 1√2. If the frequency of ac signal is halved, then the power factor of the circuit becomes
View SolutionA circuit draws 330 W from a 110 V , 60 Hz AC line. The power factor is 0.6 and the current lags the voltage. The capacitance of a series capacitor that will result in a power factor of unity is equal to
View Solution

