In ABC , if BD⊥AC and BC2=2 ACCD , then prove that AB=AC .
To solve the problem, we need to prove that AB=AC given that BD⊥AC and BC2=2⋅AC⋅CD.
1. Identify the Right Triangles:
Since BD⊥AC, triangles ABD and BDC are both right triangles.
2. Apply the Pythagorean Theorem:
For triangle ABD:
AB2=AD2+BD2(1)
For triangle BDC:
BC2=CD2+BD2(2)
3. Use the Given Condition:
We know from the problem statement that:
BC2=2⋅AC⋅CD(3)
4. Express AD:
Since AD=AC−CD, we can substitute this into equation (1):
AB2=(AC−CD)2+BD2
Expanding this gives:
AB2=AC2−2⋅AC⋅CD+CD2+BD2(4)
5. Substitute BC2 from (3) into (2):
We have:
2⋅AC⋅CD=CD2+BD2
Rearranging gives:
BD2=2⋅AC⋅CD−CD2(5)
6. Substitute (5) into (4):
Now, substitute BD2 from (5) into (4):
AB2=AC2−2⋅AC⋅CD+CD2+(2⋅AC⋅CD−CD2)
Simplifying this results in:
AB2=AC2
7. Conclude:
Taking the square root of both sides, we find:
AB=AC
Conclusion:
Thus, we have proved that AB=AC.
Since BD⊥AC, triangles ABD and BDC are both right triangles.
For triangle ABD:
AB2=AD2+BD2(1)
For triangle BDC:
BC2=CD2+BD2(2)
We know from the problem statement that:
BC2=2⋅AC⋅CD(3)
Since AD=AC−CD, we can substitute this into equation (1):
AB2=(AC−CD)2+BD2
Expanding this gives:
AB2=AC2−2⋅AC⋅CD+CD2+BD2(4)
We have:
2⋅AC⋅CD=CD2+BD2
Rearranging gives:
BD2=2⋅AC⋅CD−CD2(5)
Now, substitute BD2 from (5) into (4):
AB2=AC2−2⋅AC⋅CD+CD2+(2⋅AC⋅CD−CD2)
Simplifying this results in:
AB2=AC2
Taking the square root of both sides, we find:
AB=AC
Thus, we have proved that AB=AC.
Topper's Solved these Questions
Similar Questions
In Fig. 5.10, if AC=BD, then prove that AB=CD.
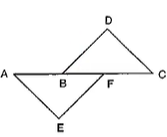
View SolutionIn ABC , ∠A is obtuse, PB⊥AC and QC⊥AB . Prove that: AB×AQ=AC×AP (ii) BC2=(AC×CP+AB×BQ)
View SolutionIn Fig. 4.124, E is a point on side CB produced of an isosceles triangle ABC with AB=AC . If AD⊥BC and EF⊥AC , prove that (i) ABDECF (ii) AB×EF=AD×EC . (FIGURE)
View SolutionIn Figure, it is given that AB=CF, EF=BD and ∠AFE= ∠CBD. Prove that AFE= ≅CBD
View SolutionIn ABC, ∠A=60o . Prove that B C^2=A B^2+A C^2-A B.A C .
View SolutionIn Figure, it is given that AB=BC and AD=EC . Prove that ABE≅CBD

View SolutionIn figure, ABC is a triangle in which ∠ABC=90∘and AD⊥BC. Prove that AC2=AB2+BC2−2BC.BD.

View SolutionIn ABC , AD is a median. Prove that AB2+AC2=2 AD2+2 DC2 .
View SolutionIn a ABC , D and E are points on AB and AC respectively such that DE/BC . If AD=2.4cm, AE=3.2cm, DE=2cm and BC=5cm , find BD and CE .
View SolutionIn figure, ABC is triangle in which ∠ABC=90∘and AD⊥CBproduced. Prove that AC2=AB2+BC2−2BC.BD.

View Solution
