The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is . Find the height of the tower.
To find the height of the tower using the given information, we can follow these steps:
Step 1: Understand the Problem
We have a tower and a point on the ground that is 30 meters away from the base of the tower. The angle of elevation from this point to the top of the tower is given as 30∘.
Step 2: Draw a Diagram
Draw a right triangle where:
- The height of the tower is represented as H.
- The distance from the point on the ground to the base of the tower is 30 m.
- The angle of elevation from the point to the top of the tower is 30∘.
Step 3: Use the Tangent Function
In a right triangle, the tangent of an angle is the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(30∘)=H30
Step 4: Find the Value of tan(30∘)
From trigonometric tables or the unit circle, we know:
tan(30∘)=1√3
Step 5: Set Up the Equation
Substituting the value of tan(30∘) into the equation gives us:
1√3=H30
Step 6: Solve for H
To find H, we can cross-multiply:
H=30⋅1√3
This simplifies to:
H=30√3
Step 7: Rationalize the Denominator
To express H in a more standard form, we can multiply the numerator and denominator by √3:
H=30√33=10√3
Conclusion
The height of the tower is:
H=10√3 meters
---
We have a tower and a point on the ground that is 30 meters away from the base of the tower. The angle of elevation from this point to the top of the tower is given as 30∘.
Draw a right triangle where:
- The height of the tower is represented as H.
- The distance from the point on the ground to the base of the tower is 30 m.
- The angle of elevation from the point to the top of the tower is 30∘.
In a right triangle, the tangent of an angle is the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(30∘)=H30
From trigonometric tables or the unit circle, we know:
tan(30∘)=1√3
Substituting the value of tan(30∘) into the equation gives us:
1√3=H30
To find H, we can cross-multiply:
H=30⋅1√3
This simplifies to:
H=30√3
To express H in a more standard form, we can multiply the numerator and denominator by √3:
H=30√33=10√3
The height of the tower is:
H=10√3 meters
Topper's Solved these Questions
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution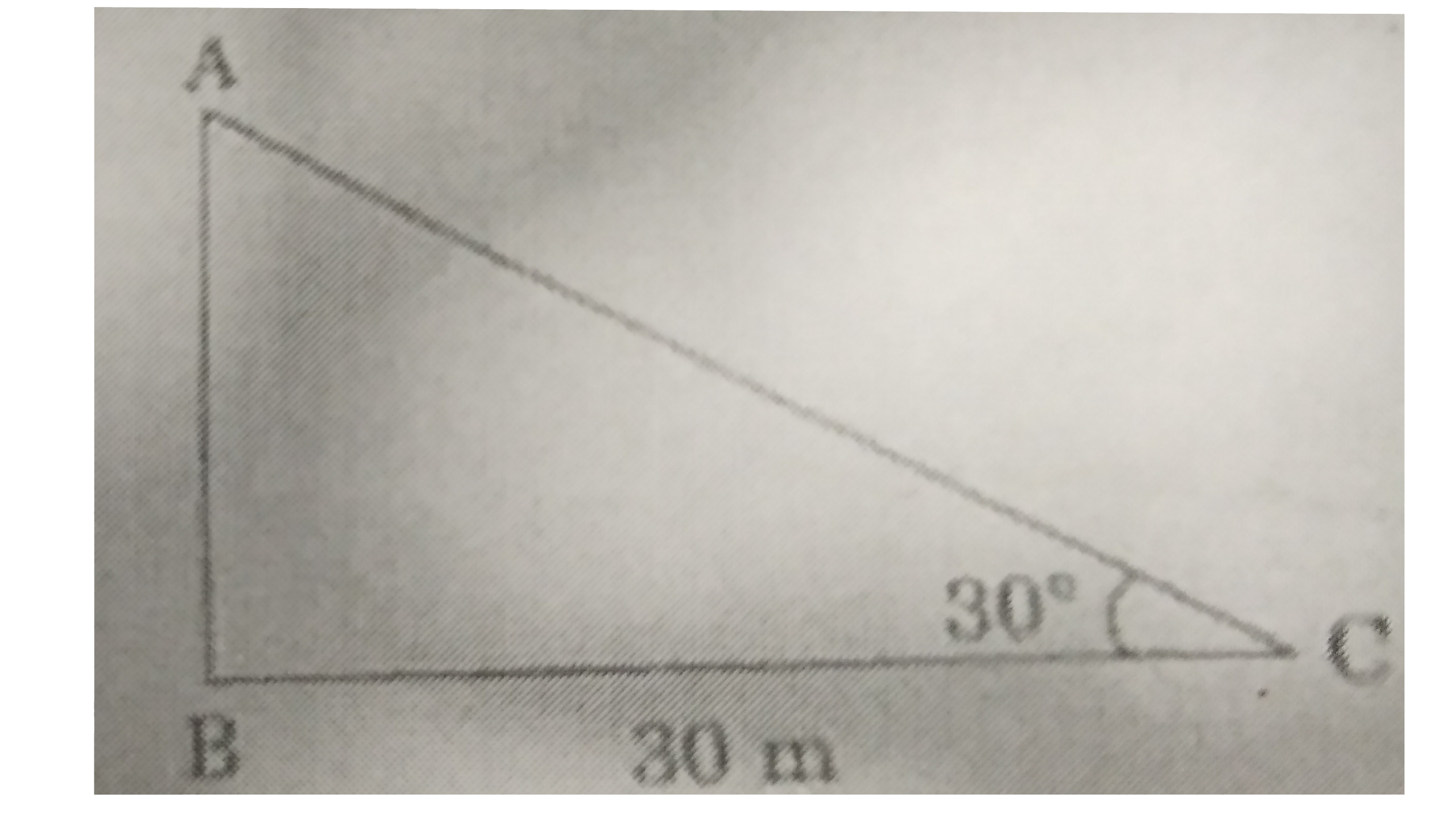
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View Solution
Knowledge Check
Assertion (A): The angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. The height of the tower is 10 m.
Reason (R): The angle of depression from B to A and Angle of elevation from A to B are equal.ABoth A and R are true and R is the correct explanation of A.BBoth A and R are true and R is not correct explanation of A.CA is true but R is false.DA is false but R is true.The angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower , is 30∘ . Then , the height ( in m) of the tower is .
A10√3B30√3C10√3D10
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionIf the angle of elevation of top of a tower from a point on the ground which is 20√3 m away from the foot of the tower is 30∘, then find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground, 50 m away from the foot of the tower, is 60°. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View Solution
