Four lenses are made from the same type of glass, the radius of curvature of each face is given below. Which will have the greatest positive power
10 cm convex and 15 cm concave
5 cm convex and 10 cm concave
15 cm convex and plane
20 cm convex and 30 cm concave
The correct Answer is:B
To determine which of the four lenses has the greatest positive power, we will use the lens maker's formula:
1f=(μ−1)(1r1−1r2)
Where:
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- r1 and r2 are the radii of curvature of the two lens surfaces.
Since all lenses are made from the same type of glass, μ will be constant for all lenses. Therefore, we can focus on the term 1r1−1r2 to find the lens with the greatest positive power, as power P is given by:
P=1f
1. Identify the radii of curvature for each lens:
- Lens A: r1=10cm (convex), r2=−15cm (concave)
- Lens B: r1=5cm (convex), r2=−10cm (concave)
- Lens C: r1=15cm (convex), r2=∞ (plane)
- Lens D: r1=20cm (convex), r2=−30cm (concave)
2. Calculate 1f for each lens using the lens maker's formula:
- Lens A:
1fA=110−(−115)=110+115
=330+230=530=16(positive)
- Lens B:
1fB=15−(−110)=15+110
=210+110=310(positive)
- Lens C:
1fC=115−(−1∞)=115−0=115(positive)
- Lens D:
1fD=120−(−130)=120+130
=360+260=560=112(positive)
3. Compare the values of 1f for each lens:
- Lens A: 16
- Lens B: 310
- Lens C: 115
- Lens D: 112
4. Determine which lens has the greatest positive power:
- Convert to a common denominator to compare:
- Lens A: 530
- Lens B: 930
- Lens C: 230
- Lens D: 2.530
The greatest value is for Lens B, which has a power of 310.
Conclusion:
The lens with the greatest positive power is Lens B with a power of 310.
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- r1 and r2 are the radii of curvature of the two lens surfaces.
- Lens A: r1=10cm (convex), r2=−15cm (concave)
- Lens B: r1=5cm (convex), r2=−10cm (concave)
- Lens C: r1=15cm (convex), r2=∞ (plane)
- Lens D: r1=20cm (convex), r2=−30cm (concave)
- Lens A:
1fA=110−(−115)=110+115
=330+230=530=16(positive)
1fB=15−(−110)=15+110
=210+110=310(positive)
1fC=115−(−1∞)=115−0=115(positive)
1fD=120−(−130)=120+130
=360+260=560=112(positive)
- Lens A: 16
- Lens B: 310
- Lens C: 115
- Lens D: 112
- Convert to a common denominator to compare:
- Lens A: 530
- Lens B: 930
- Lens C: 230
- Lens D: 2.530
The lens with the greatest positive power is Lens B with a power of 310.
Topper's Solved these Questions
- BOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES105videos
REFRACTION AT SPHERICAL SURFACES
- BOOK - CP SINGHCHAPTER - REFLECTION OF LIGHTEXERCISE - EXERCISES67videos
REFLECTION OF LIGHT
- BOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises110videos
REFRACTION OF LIGHT BY PLANE SURFACES
Similar Questions
Four positions of dice are given below. Which letter will be opposite to D?
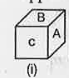
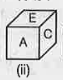
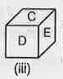
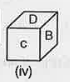 View Solution
View SolutionConvex lens is made of glass of refractive index 1.5 If the radius of curvature of each of the two surfaces is 20cm find the ratio of the powers of the lens, when placed in air to its power, when immersed in a liquid of refractive index 1.25.
View Solution
Knowledge Check
- Question 1 - Select One
Four positions of dice are given below. Which letter will be opposite to D?
 ABBCCDDA
ABBCCDDA - Question 2 - Select One
A plano-concave lens is made of glass (R.l. = 1.5) and the radius of curvature of the curved face is 50 cm. The power of the lens is
A-1.0DB-0.5DC+1.0DD+0.5 D - Question 3 - Select One
A plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
A+0.5DB−0.5DC−2DD+2D
A biconcave lens is made of a material of refractive index μ. If the radius of curvature of each face of the lens is 32 times the focal length of lens, calculate value of μ.
View SolutionA double convex lens has power P and same radii of curvature R of both the surfaces. The radius of carvature of a surface of plano-convex lens made of the same meterial with power 1.5 P is :
View SolutionTwo lenses, one convex and other concave, are of same mass and same radius. Which will have greater moment of inertia?
View SolutionFour towns, all at about the same altitude and on the same line of latitude, have mean January temperatures as given below, which town is farthest from the sea?
View SolutionAll the listed things below are made of flint glass. Which one of these have greatest dispersive power (ω).
View Solution

