Candidates of four schools appear in a mathematics test. The data were as follows School, No. of Candidates, Average Score I II III IV, 60 48 Not available 40, 75 80 55 50 If the average score of the candidates of all the four schools is 66, find the number of candidates that appeared from school III.
Let the number of candidates that appeared from school lll be x

Given:Mean =66
Mean=∑fixi∑fi
66=10340+55x148+x
66(148+x)=10340+55x
9768+66x=10340+55x
66x−55x=10340−9768
11x=572
∴x=57211=52
∴ the Number of candidates from school lll is52
Topper's Solved these Questions
Similar Questions
Candidates of four schools appear in a mathematics test. The data were as follows School, No. of Candidates, Average Score I II III IV, 60 48 Not available 40, 75 80 55 50 If the average score of the candidates of all the four schools is 66, find the number of candidates that appeared from school III.
View SolutionWhat was the total number of failed candidates from school - C in the year 2008 and the number of candidates who appeared in the exam from school -D in the year 2006?
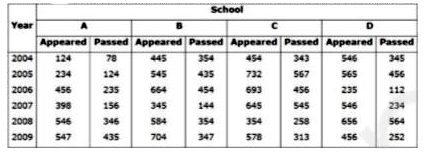 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The average score of boys in an examination of a school is 71 and that of the girls is 73. The average score of the whole school in that examination is 71.8. Find the ratio of the number of boys to the number of girls that appeared in the examination.
A4:5B3:2C3:5D5:2 - Question 2 - Select One
Students of two schools appeared for a common test carrying 100 marks. The arthmetic means of their marks for school I and II are 82 and 86 respectively, If the number of students of school II is 1.5 times the number of students of school I, what is the arithmetic mean of the marks of all the students, of both the school ?
A84.5B84.2C84.4DThis cannot be calculated with the given data - Question 3 - Select One
In the entrance test of Wharton Business School, the maximum marks for each of three papers is n and that for the fourth paper is 2n. Find the number of ways in which a candidate can get 3n marks.
A1/6 (n+1)(n+2)B16(n+1)(5n2+10n+6)C12(n2+3n−6)Dnone of these
In which year was the difference between the number of candidates who appeared and passed in the exam from school - B second lowest?
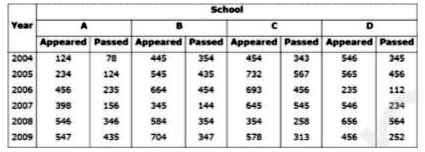 View Solution
View SolutionWhat was the respective ratio between the number of candidates who appeared from school -C in the year 2006 and the number of candidate who passed in the exam from school - D in the year 2009 ?
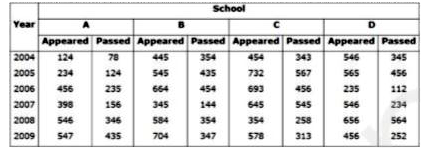 View Solution
View SolutionIn an examination, the average of marks was found to be 50. For deducting marks for computational errors, the marks of 100 candidates had to be changed from 90 to 60 each and so the average of marks came down to 45. The total number of candidates, who appeared at the examination, was :
View SolutionNumber of candidates who passed in the exam from school B in the year 2005 was approximately what percent of number of students appeared in school - A in the year 2008 ?
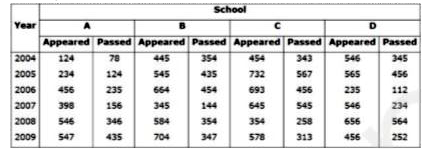 View Solution
View SolutionIn what time will a sum double itself at 8% per annum simple interest ?
View Solution

