An open rectangular tank 1.5 m wide 2m deep ad 2 m long is half filled with water. It is accelerated horizontally at 3.27m/s2 in the direction of its length determine the depth of water at each end of tank.
To solve the problem, we need to determine the depth of water at each end of the tank when it is accelerated horizontally. Here’s a step-by-step solution:
Step 1: Understand the Problem
We have an open rectangular tank that is 1.5 m wide, 2 m deep, and 2 m long, which is half-filled with water (1 m deep). The tank is accelerated horizontally at 3.27m/s2 in the direction of its length.
Step 2: Determine the Angle of the Water Surface
When the tank is accelerated, the water surface will tilt, forming an angle θ with the horizontal. The angle can be found using the formula:
tanθ=ag
where:
- a=3.27m/s2 (acceleration of the tank)
- g=9.8m/s2 (acceleration due to gravity)
Substituting the values:
tanθ=3.279.8≈0.333
Step 3: Calculate the Depth at Each End of the Tank
Let’s denote the depth of water at end A (the end where the tank starts accelerating) as dA and at end B (the opposite end) as dB.
1. Depth at End A:
The depth at end A can be calculated as follows:
dA=d0−w⋅tanθ
where:
- d0=1m (initial depth of water)
- w=1.5m (width of the tank)
Substituting the values:
dA=1−1.5⋅tanθ
Calculating tanθ:
dA=1−1.5⋅0.333≈1−0.5=0.5m
2. Depth at End B:
The depth at end B can be calculated as follows:
dB=d0+w⋅tanθ
Substituting the values:
dB=1+1.5⋅tanθ
Calculating:
dB=1+1.5⋅0.333≈1+0.5=1.5m
Final Answer
- Depth at end A: 0.5m
- Depth at end B: 1.5m
We have an open rectangular tank that is 1.5 m wide, 2 m deep, and 2 m long, which is half-filled with water (1 m deep). The tank is accelerated horizontally at 3.27m/s2 in the direction of its length.
When the tank is accelerated, the water surface will tilt, forming an angle θ with the horizontal. The angle can be found using the formula:
- a=3.27m/s2 (acceleration of the tank)
- g=9.8m/s2 (acceleration due to gravity)
Let’s denote the depth of water at end A (the end where the tank starts accelerating) as dA and at end B (the opposite end) as dB.
The depth at end A can be calculated as follows:
- d0=1m (initial depth of water)
- w=1.5m (width of the tank)
The depth at end B can be calculated as follows:
- Depth at end A: 0.5m
- Depth at end B: 1.5m
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Elasticity)16videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Surface Tension)21videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
Similar Questions
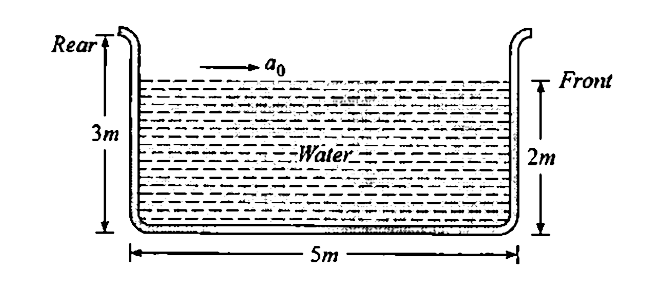
An open reactangular tank with tank with dimensions 5×4m×3m contains water upto a height of 2m is accelerated horizontally along the longer life.
(a) Determine the maximum acceleration that can be given without spilling the water.
(b) If this acceleration is inscreased by 20%. Calculate the percentage of water spilt over.
(c) If initially,the tank is closed at the top and is accelerated horizontally by 9m/s2,find the gauge pressure at the bottom of the front and rear walls of the tank. (g=10m/s2)
 View Solution
View SolutionA raectangular tank 2 m deep, 5 m broad and 10 m long is filied with water . Calculate the thrust on each of the sides and on the base . Density of water 1000kgm−2andg=9.8ms−2)
View Solution
Knowledge Check
- Question 1 - Select One
A rectangular water tank is 3m long, 2m wide and 5 m high. How many litres of water can it hold?
A30000B15000C25000D35000 - Question 2 - Select One
A rectangular water tank is 5 m high, 3 m long and 2 m wide. How many litres of water can it hold?
A30000B15000C25000D35000 - Question 3 - Select One
A rectangular tank of depth 8 m is full of water (μ=4/3). The bottom is seen at the depth
A6 cmB83 cmC8 cmD10 cm
.A rectangular water tank is 5m high, 3m long and 2m wide. How many litres water it can hold
View SolutionA rectangular tank of length 10m, breadth 5m and depth 6m is full of water. How much water does it contain?
View Solution_E01_226_Q01.png)
An open retangular tank 5m×4m×3m high containing water upto a height of 2m is accelerated horizontally along the longer side.
(i). Determine the maximum acceleration that can be given without spilling the water.
(ii). Calculate the percentage of water split over if this acceleration of increased by 20%
(iii). If initially the tank is closed at the top and its accelerated horizontally by 9m/s2 find the gauge pressure at the bottom of the front and rear walls of the tank.View SolutionA closed rectangular tank 10m long, 5m wide and 3m deep is completely filled with an oil of specific gravity 0.92. Find the pressure difference between the rear and front corners of the tank, if it is moving with an acceleration of 3m/s2 in the horizontal direction.
View SolutionA water tank is 11 m long, 10 m wide and 9 m high. It is filled with water to a level of 6 m. What part of the tank is empty?
View Solution

