Calculate the gravitational potential at the centre of base of a solid hemisphere of mass M, radius R.
Gravitational potential at the centre of curvature of a thin uniform wire of mass M, bent into a semicircle of radius R, is also equal V.
In part (A) if the same wire is bent into a quarter of a circle then also the gravitational potential at the centre of curvature will be V.
In part (A) if the wire mass is non uniformly distributed along its length audit is bent into a semicircle radius R, gravitational potential at the centre is V
None of these
The correct Answer is:A, C
Gravitational potential due to hemisphere at the centre is V because distance of each mass particle from the centre O is R. If the distance between the point and mass is changed potential will also change.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 3 (Miscellaneous Type Questions)20videos
GRAVITATION
- BOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 A (Conceptual Subjective Exercise)14videos
GRAVITATION
- BOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 1 (Check your Grasp)28videos
GRAVITATION
- BOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective14videos
GEOMETRICAL OPTICS
- BOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)48videos
KINEMATICS-2D
Similar Questions
Calculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
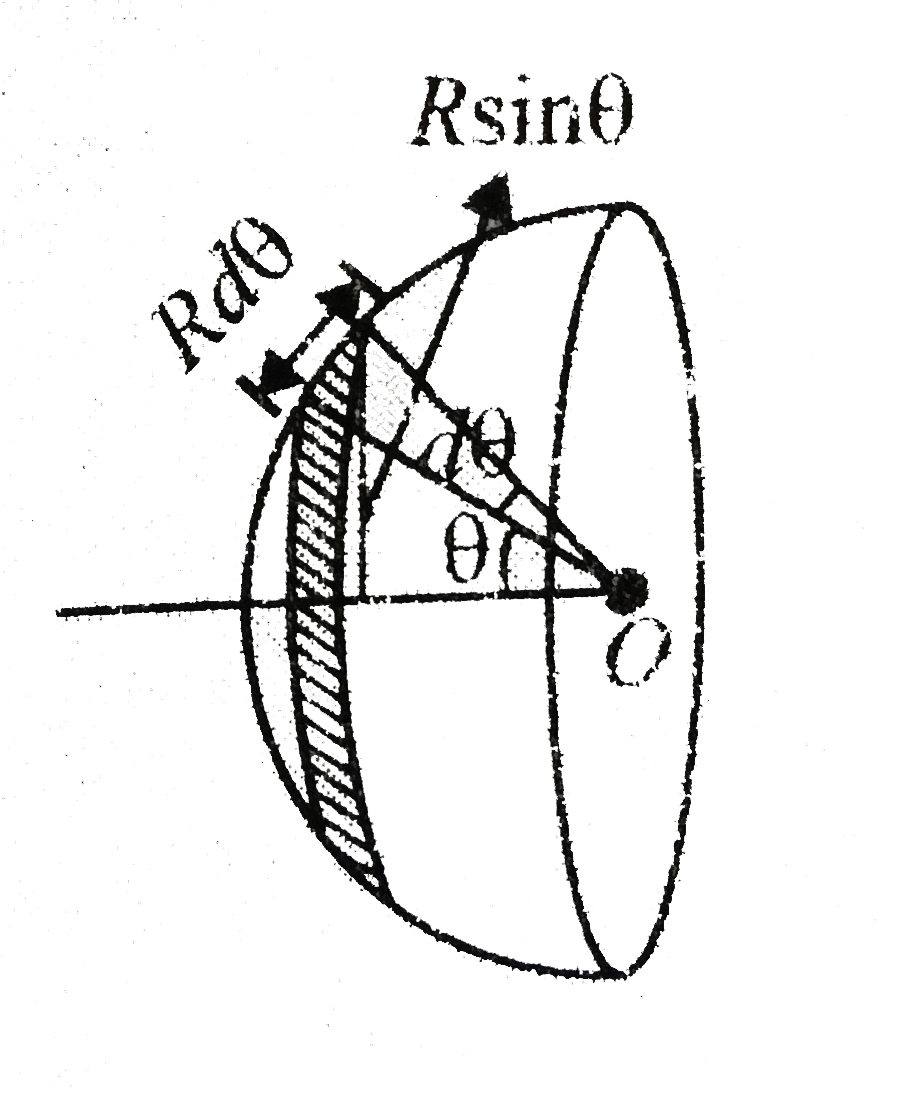 View Solution
View SolutionCalculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
View Solution
Knowledge Check
- Question 1 - Select One
The total surface area of a solid hemisphere of radius r is
Aπr2B2πr2C3πr2D4πr2 - Question 2 - Select One
Find distance of centre of mass of solid hemisphere of radius 8cm from centre
A3 cmB3/8 cmC4 cmD2 cm
The centre of gravity of a solid hemisphere of radius a is
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionThe centre of gravity of a solid hemisphere of radius a is : (A)
View SolutionDetermine the position of the centre of mass of a hemisphere of radius R.
View SolutionCalculate the self-gravitational potential energy of matter forming a. a thin uniform shell of mass M and radius R, b. a uniform sphere of mass m and radius R.
View SolutionA particle of mass m was transferred from the centre of the base of a uniform hemisphere of mass M and radius R into infinity.
What work was performed in the process by the gravitational force exerted on the particle by the hemisphere?View Solution

