A particle of mass m was transferred from the centre of the base of a uniform hemisphere of mass M and radius R into infinity.
What work was performed in the process by the gravitational force exerted on the particle by the hemisphere?
One can imagine that the uniform hemisphere is made up of thin hemispherical layers of radii ranging from 0 to R. Let us consider such a layer (figure). Potential at point O, due to this layer is,
dφ=−γdmr=−3γMR3rdr, where dm=M(2/3)πR2(4πr22)dr
(This is because all points of each hemispherical shell are equidistant from O.)
Hence, φ=∫dφ=−3γMR3R∫0rdr=−3γmM2R
Hence, the work done by the gravitational field force on the particle of mass m, to remove it to infinity is given by the formula
A=mφ, since φ=0 at infinity.
Hence the sought work,
A0−∞=−3γmM2R

(The work done by the external agent is −A.)
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body56videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum82videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
Determine the centre of mass of a uniform hemisphere of radius R.
View SolutionA particle of mass 1kg is kept on the surface of a uniform sphere of mass 20kg and radius 1.0m. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View Solution
Knowledge Check
- Question 1 - Select One or More
A particle at a distance r from the centre of a uniform spherical planet of mass M radius R (ltr) has a velocity of magnitude v.
Afor 0<v<√GMr trajectory may be ellipseBfor v =√GMr trajectory may be ellipseCfor √GMr<v<√2GMr trajectory may be ellipse.Dfor v =sqrt((GM)/r trajectory may be circle - Question 2 - Select One
A particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
A−GmRB−3GmRC−4GmRD−2GmR
Calculate the gravitational potential at the centre of base of a solid hemisphere of mass M, radius R.
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionDetermine the position of the centre of mass of a hemisphere of radius R.
View SolutionA small mass m is transferred from the centre of a hollow sphere of mass M to infinity. Find work done in the process. Compare this with the situation if instead of a hollow sphere, a solid sphere of same mass were there.
View SolutionA small mass m is transferred from the centre of a hollow sphere of mass M to infinity. Find work done in the process. Compare this with the situation if instead of a hollow sphere, a solid sphere of same mass were there.
View SolutionCalculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
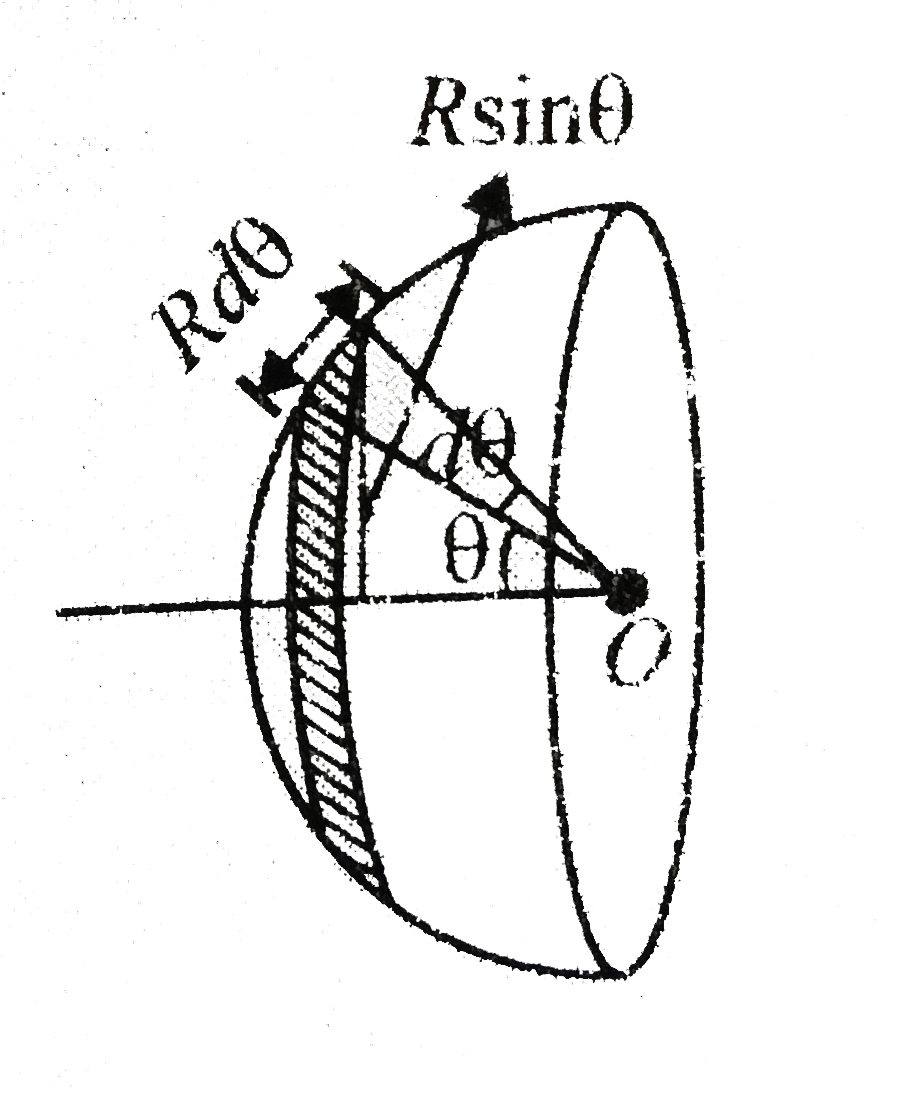 View Solution
View Solution

