_E01_106_Q01.png)
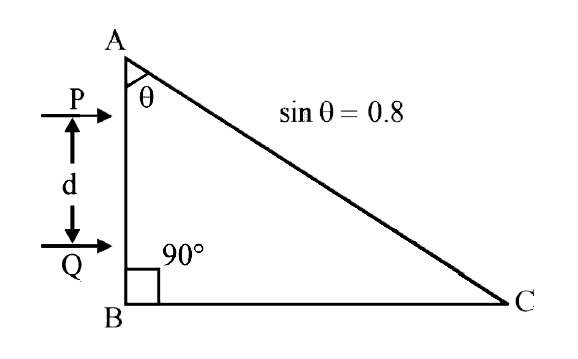
Two parallel beams of light P and Q (separation d) containing radiations of wavelengts and 5000 Å (which are mutually coherent in each wavelength separately) are incident normally on a prism as shown in figure the refractive index of the prism as a function of wavelength is given by the relation where is in Å and b is a positive constant. The value of b is such that the condition for total reflection at the face AC is just satisfied for one wavelength and is not satisfied for the other. A convergent lens is used to bring these transmitted beams into focus. If the intensities of the upper and the lower beams immediately after transmission from the face AC, are 4I and I respectively, find the resultant intensity at the focus.
The correct Answer is:9I
_E01_106_S01.png)
Path difference between rays 1 and 2:
…(i)
Further
Substituting in equation (i), we get
Phase difference between rays 1 and 2 will be 0 or these two rays will interfere constructively. So maximum intensity will be obtained from their interference or
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 5 A (Previous Years Questions)17videos
WAVE OPTICS
- BOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 5 B (Previous Years Questions)19videos
WAVE OPTICS
- BOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 4 A (Conceptual subjective Exercise)9videos
WAVE OPTICS
- BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)7videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
Similar Questions
To deminstrate the phenimenon of interference, we require two sources which emit radiation
11:36View SolutionTwo beams of ligth having intensities I and 4I interface to produce a fringe pattern on a screen. The phase difference between the beams is π2 at point A and π at point B. Then the difference between the resultant intensities at A and B is
05:43View SolutionConsider interference between two sources of intensity I and 4I. Find out resultant intensity where phase difference is (i). π/4
(ii). π
(iii). 4π00:45View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :
 02:52View Solution
02:52View SolutionTwo parallel beams of light P and Q (separation d) containing radiation of wavelengths 4000A and 5000A (which are mutually coherent in each wavelength separately) are incident normally on a prism as shown in fig. The refractive index of the prism as a function of wavelength is given by the relation. μ(λ)=1.20+bλ2 Where λ is in A and b is positive constant. The value of b is such that the condition wave length and is not satisfied for the other.
(a) Find the value of b.
(b) find the deviation of the beams transmitted through the face AC. (c) A convergent lens is used to bring these transmitted beams into focus. If the intensities of transmission form the face AC, are 41 and I respectively, find the resultant intensity at the focus.
 02:31View Solution
02:31View SolutionA beam of light of wavelength 400 nm is incident normally on a right angled prism as shown in Fig. It is observed that light just grazes along the surface AC after falling on it. If refractive index mu of the material of prism varies with wavelength lamda as mu = 1.2 + (b)/(lamda^2) Calculate the value of b and mu of prism material for lamda = 500 nm . Given theta = sin^-1 (0.625) . .
01:08View SolutionAccording to Cauchy's formula, the refractive index mu of a material is related to wavelength lamda as………. .
02:33View SolutionThe angles of incidence and refraction of a monochromatic ray of light of wavelength λ at an air-glass interface are i and r, respectively. A parallel beam of light with a small spread δλ in wavelength about a mean wavelength λ is refracted at the same air-glass interface. The refractive index μ of glass depends on the wavelength λ as μ(λ)=a+b/λ2 where a and b are constants. Then the angular spread in the angle of refraction of the beam is
07:15View SolutionIf energy of photon is Eproph^(a)c^(b)lamda^(d) . Here h= Planck's constant c= speed of light and lamda= wavelength of photon Then, the value of a,b and d are
01:47View SolutionThe phase difference between oscillatory motion of two points separated by a distance of λ2 is ( where λ is the wavelength)
03:42View SolutionWavelengths of different radiations are given below : lamda (A) = 300nm, lamda (B) = 300mu m, lamda(C) = 3nm, lamda(D) = 30 Å Arrange these radiations in the increasing order of their energies.
01:20View Solution400nm तरंगदैर्ध्य का प्रकाश पुंज एक समकोणिक प्रिज्म पर लंबवत आपतित होता है। यह देखा जाता है कि प्रकाश पुंज पृष्ठ AC पर पढ़कर इससे स्पर्श रेखीय निकलता है (नीचे दिए गया चित्र। प्रिज्म के पदार्थ का अपवर्तनांक तरंगदैर्य (λ) के साथ निम्न प्रकार बदलता है
μ=1.2+bλ2
b का मान तथा λ=500nm के लिए प्रिज्म के पदार्थ का अपवर्तनांक ज्ञात कीजिय। दिया है θ=sin−1(0.625)01:30View Solutionदो समान्तर प्रकाश पुँज p तथा Q हैं | इनमें 4000Å तथा 5000Å तरंगदैर्ध्यों के विकिरण हैं ( जो प्रत्येक तरंगदैर्ध्य में अलग - अलग परस्पर कला - सम्बद्ध हैं ) तथा ये एक प्रिज्म पर अभिलम्बवत आपतित हैं | प्रिज्म का अपवर्तनांक n,λ के फलन के रूप में निम्न समीकरण द्वारा दिया जा सकता है,
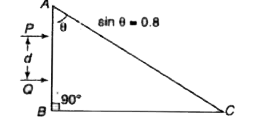
जहाँ λ,Å में है तथा b एक धनात्मक नियतांक है | b का मान इस प्रकार है कि एक तरंगदैर्ध्य के लिए फलक AC पर पूर्ण परावर्तन की शर्त ठीक पूरी होती है, जबकि दूसरी तरंगदैर्ध्य के लिए पूरी नहीं होती |
(a) b का मान ज्ञात कीजिए |
(b) फलक AC से पारगमित पुंजों का विचलन ज्ञात कीजिए |
(c) पारगमित पुंजों को एक अभिसारी लेंस द्वारा फोकस में लाया जाता है | यदि फलक AC से गुजरते ही ऊपरी व निचली पुंजों की तीव्रताएँ क्रमशः तथा I हों तो फोकस पर परिणामी तीव्रता ज्ञात कीजिए |04:28View SolutionThe refractive index ( mu ) of glass is related to the wavelength (lambda) of incident light as mu = A +(B)/(lambda^(2)) , where A and B are constants. Find out the dimensions of A and B.
06:21View Solutionλ/2 दूरी द्वारा अलग किए गए दो बिन्दुओं की दोलनी गति के मध्य कलान्तर है(जहाँ λ तरंगदैर्घ्य है)
View Solution

