Choose the incorrect option/options :
If acceleration is positive than speed of particle is increasing.
If acceleration is negative than speed of particle is decreasing.
In projectile motion speed of particle first decreases than increases.
In ground to ground projectile motion horizontal range cannot be equal to the maximum height from the ground.
The correct Answer is:A, B, C, D
To solve the question of identifying the incorrect options regarding motion and projectile motion, we will analyze each option step-by-step.
1. Option A: If the acceleration is positive, then the particle's speed will increase.
- Analysis: This statement is incorrect. Acceleration indicates the change in velocity. If a particle is moving in the negative direction (i.e., has negative velocity) and experiences positive acceleration, the velocity will decrease (the particle is slowing down). Thus, speed (the magnitude of velocity) will not necessarily increase.
- Conclusion: This option is incorrect.
2. Option B: If the acceleration is negative, then the speed of the particle will also decrease.
- Analysis: This statement is also incorrect. If a particle is moving in the positive direction (positive velocity) and has negative acceleration, it will slow down, leading to a decrease in speed. However, if the particle is moving in the negative direction with negative acceleration, its speed will actually increase (the particle is moving faster in the negative direction). Therefore, this statement is not universally true.
- Conclusion: This option is incorrect.
3. Option C: If the speed of the particle in projectile motion is first decreased, then increased.
- Analysis: This statement is partially correct for vertical motion but misleading for horizontal motion. In projectile motion, the vertical component of speed decreases until the peak and then increases. However, the horizontal component remains constant (assuming no air resistance). If a projectile is thrown horizontally, its horizontal speed does not change, while its vertical speed increases due to gravity. Thus, the overall speed does not follow the pattern described.
- Conclusion: This option is incorrect.
4. Option D: In ground-to-ground projectile motion, the horizontal range can never be equal to the maximum height.
- Analysis: This statement is incorrect. The formulas for maximum height (H) and horizontal range (R) in projectile motion can be manipulated to show that there are angles for which the horizontal range can equal the maximum height. Specifically, if we set the two equations equal, we can find conditions under which this equality holds true.
- Conclusion: This option is incorrect.
Final Conclusion:
All options A, B, C, and D are incorrect.
- Analysis: This statement is incorrect. Acceleration indicates the change in velocity. If a particle is moving in the negative direction (i.e., has negative velocity) and experiences positive acceleration, the velocity will decrease (the particle is slowing down). Thus, speed (the magnitude of velocity) will not necessarily increase.
- Conclusion: This option is incorrect.
- Analysis: This statement is also incorrect. If a particle is moving in the positive direction (positive velocity) and has negative acceleration, it will slow down, leading to a decrease in speed. However, if the particle is moving in the negative direction with negative acceleration, its speed will actually increase (the particle is moving faster in the negative direction). Therefore, this statement is not universally true.
- Conclusion: This option is incorrect.
- Analysis: This statement is partially correct for vertical motion but misleading for horizontal motion. In projectile motion, the vertical component of speed decreases until the peak and then increases. However, the horizontal component remains constant (assuming no air resistance). If a projectile is thrown horizontally, its horizontal speed does not change, while its vertical speed increases due to gravity. Thus, the overall speed does not follow the pattern described.
- Conclusion: This option is incorrect.
- Analysis: This statement is incorrect. The formulas for maximum height (H) and horizontal range (R) in projectile motion can be manipulated to show that there are angles for which the horizontal range can equal the maximum height. Specifically, if we set the two equations equal, we can find conditions under which this equality holds true.
- Conclusion: This option is incorrect.
All options A, B, C, and D are incorrect.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS107videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Physics775videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-330videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems13videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS127videos
TEST SERIES
Similar Questions
Knowledge Check
- Question 1 - Select One
select the incorrect option
ASodium peroxide dissolves in water giving H2O2 and NaOHBBoth LiNO3 and NaNO3 on heating separately decompose and each liberates two gases NO2 and O2CSolvay process can not be used for the manufacture of potassium hydrogen carbonate.DAlkali metals are prepared only by the electrolysis of their fused chlorides. - Question 2 - Select One
select the incorrect option
ASodium peroxide dissolves in water giving H2O2 and NaOHBBoth LiNO3 and NaNO3 on heating separately decompose and each liberates two gases NO2 and O2CSolvay process can not be used for the manufacture of potassium hydrogen carbonate.DAlkali metals are prepared only by the electrolysis of their fused chlorides. - Question 3 - Select One or More
Choose the incorrect option (s):
APicric acid & Benzoic acid can be distringuished by NaHCO3B < rate of SN2 reactionC
< rate of SN2 reactionC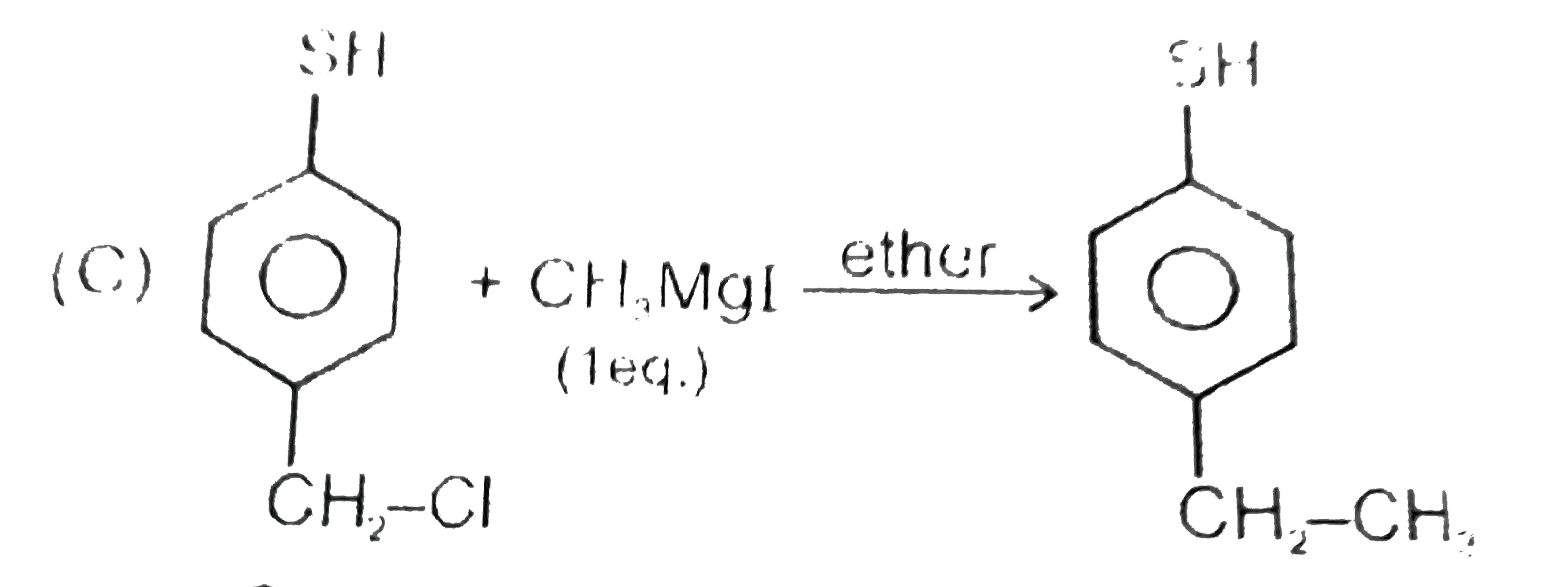 +CH3MgIether−−→(1eq.) D
+CH3MgIether−−→(1eq.) D
does't show tautomerism.