Circles with radii 3, 4 and 5 touch each other externally. If P is the point of intersection of tangents to these circles at their points of contact, then if the distance of P from the points of contact is λ then λ2 is _____
The correct Answer is:5
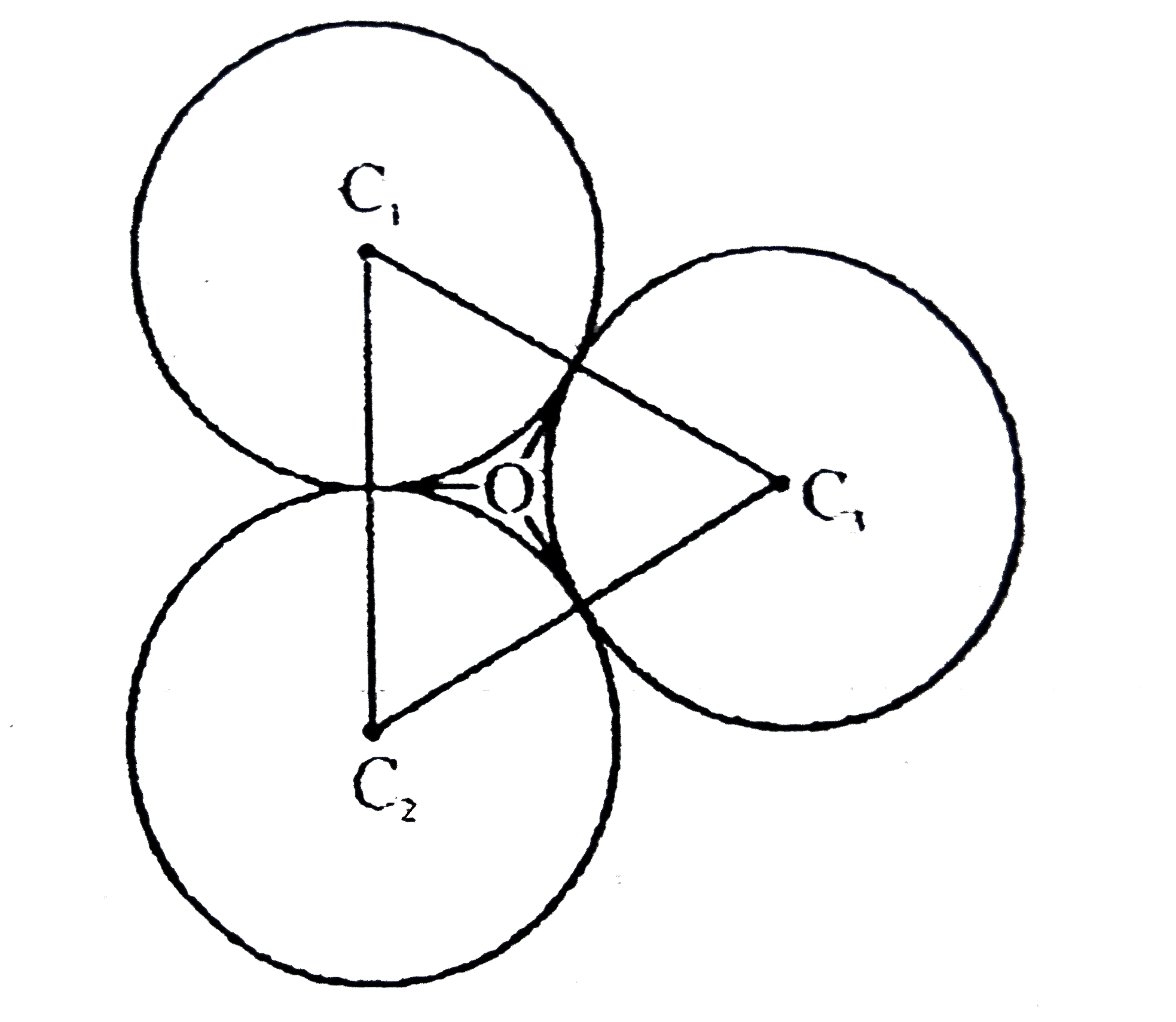
Indeed the required length will be in radius of the triangle
C1C2C3 whose sides are 3+4,4+5,5+3
Use r=Δs to get distance √5⇒λ=5
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Math105videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - MATHEMATICS263videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - I MATHEMATICS SEC - 21videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPEREXERCISE - CHEMISTRY37videos
TEST PAPER
- BOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - MATHEMATICS131videos
TEST SERIES
Similar Questions
Knowledge Check
- Question 1 - Select One
Three circles with radii 3,4 and 5 touch each other externally. If P is the only point of intersection of tangents to these circles, find the distance of P from the points of contact.
A√5B√6C√7D3 - Question 2 - Select One
Two circles touch each other externally at P. AB is a direct common tangent to the two circles, A and B are point of contact and ∠PAB=35∘. Then ∠ABP is
A35∘B55∘C65∘D75∘ - Question 3 - Select One
Two circles touch each other externally at a point P and a direct common tangent touches the circles at the points Q and R respectively. Then ∠QPR is
A45∘B180∘C90∘D60∘
Three circles whose radii are a,b and c and c touch one other externally and the tangents at their points of contact meet in a point. Prove that the distance of this point from either of their points of contact is (abca+b+c)12.
View SolutionTwo circles with radii a and b respectively touch each other externally . Let c be the radius of a circle that touches these two circles as well as a common tangent to the circles. Then
View SolutionTwo circles touch each other externally. Prove that the lengths of the tangent drawn to the two circles from any point on the common tangent lie at the point of contact of two circles are equal .
View SolutionTwo circles with radii 5 cm and 8 cm touch each other externally at a point A. If a straight line through the point A cuts the circles at points P and Q respectively, then AP : AQ is
View SolutionTwo circle touch each other externally at the point (0,k) and y-axis is the common tangent to these circles. Centres of these circle lie on the line
View Solution

