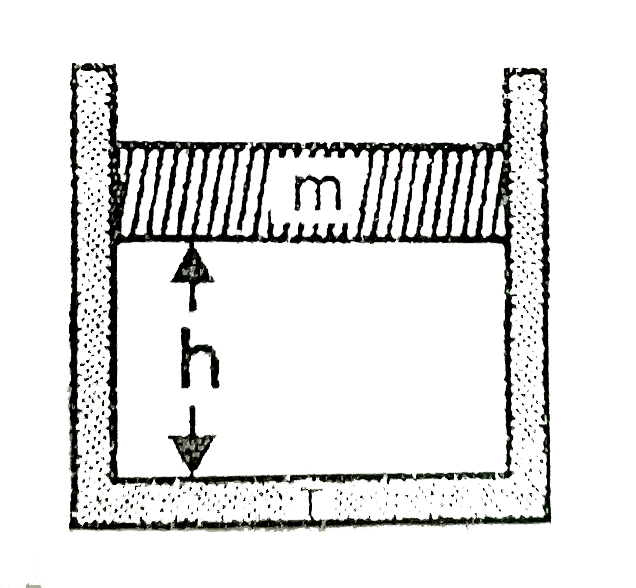
(i) A conducting cylinder whose inside diameter is contains air compressed by a piston of mass , which can slides freely in the cylinder shown in the figure. The entire arrangement is immersed in a water bath whose temperature can be controlled. The syetem is initially in equilibrium at temperature . The initial height of the piston above the bottom of the cylinder is and .
If the temperature of the water bath is gradually increased to a final temperature . Find teh height of the piston (in cm) at that instant?
(ii) In the above question, if we again start from the initial conditions and the temperature is again gradually raised, and weights are added to the piston to keep its height fixed at . Find the value of teh added mass when the final temperature becomes ?
The correct Answer is:(i)
(ii)
(i) Initial pressure of gas final pressure
and
For air inside cylinder
(ii) Here volume is constant
Topper's Solved these Questions
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (D)
2 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (I)
2 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (A)
3 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - RESONANCECHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Exercise
64 Videos
- MAGNETIC FIELD AND FORCESBOOK - RESONANCECHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Exercise
65 Videos
Similar Questions
16g of oxygen at 37∘C is mixed with 14g of nitrogen at 27∘C. Find the temperature of the mixture?
View SolutionFind the molar heat capacity (in terms of R) of a monoatomic ideal gas undergoing the process: PV1/2=constant?
View SolutionWhen a gas expands at constant temperature, why does the pressure decrease? Explanin it on the basis of kinetic theory of gases.
View SolutionThe ratio of r.m.s speed to the r.m.s angular speed of a diatomic gas at certain temperature is (assume m =mass of one molecule, M=molecular mass, I=moment of inertia of the molecules )
View SolutionOne tesla is equal to
View SolutionAir is contained in a piston-cylinder arrangement as shown in Fig. with a cross-sectional area of 4 cm^(2) and an initial volume of 20 cc . The air is initially at a pressure of 1 atm and temperature of 20^(@)C . The piston is connected to a spring whose spring constant is k = 10^(4) N//m , and the spring is initially underformed. How much heat must be added to the air inside cylinder to increase the pressure to 3 atm (for air, CV = 718 J//kg.^(@)C , molecular of air 28.97)?
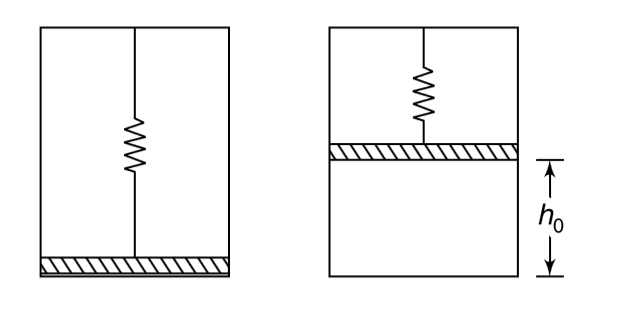
View SolutionA freely sliding massive piston is supported by a spring inside a vertical cylinder as shown. When all air is pumped out of the container, the piston remains in equilibrium with only a tiny gap between the piston and the bottom surface of the cylinder. An ideal gas at temperature T0 is slowly injected under the piston so that it rises to height h0 (see figure). Calculate the height of the piston from the bottom of the container if the temperature of the gas is slowly raised to 4T0.
View SolutionAn adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T_(0) and pressure P_(0) occupies a volume V_(0) in the cylinder. The atmospheric pressure is P_(0) and the atmospheric temperature is T_(1) (gt T_(0)) . Find (a) the temperature of the gas as a function of time (b) the height raised by the piston as a function of time. Neglect friction and heat capacities of the container and the piston.
View SolutionA gas is enclosed in a cylinder with a freely movable piston. The load on the piston is gradually decreased. The temperature of the gas can be changed by placing the cylinder in hot and cold heat reservoirs. The figure shown P-V graph of such a cylinder. What intermediate can be drawn the nature of change in the temperature of the gas ? " "
View SolutionTwo vertical cylinders are connected by a small tube at the bottom. It contains a gas at constant temperature . Initially the pistons are located at the same height. The diameters of the two cylinders are different . Outside the cylinder the space is vaccum . Gravitational acceleration is h,h_(0) = 20cm , m_(1) = 2kg "and" m_(2) =1kg . The pistons are initially in equilibrium. If the masses of the piston are interchanged find the separation between the two pistons when they are again in equilibrium. Assume constant temperature .
View SolutionThe piston cylinder arrangement shown contains a diatomic gas at temperature 300 K The cross sectinal area of the cylinder is 1m2. Initially the height of the piston above th base of the cyclinder is 1 m. The temperature is now raised to 400 K at constant pressure. There is no heat loss in the process.. Find the new height (in m) of the piston above the base of the cylinder.
View Solutionपिस्टन सिलिण्डर निकाय में 300 केल्विन ताप पर द्विपरमाणुक गैस भरी है | सिलिण्डर का अनुप्रस्थ - क्षेत्रफल 1 मी है | प्रारम्भ में पिस्टन की सिलिण्डर के आधार से ऊँचाई 1 मी है | निकाय का ताप अब स्थिर दाब पर बढ़ाकर 400 केल्विन कर दिया जाता है | पिस्टन की आधार से ऊँचाई कितनी हो जायेगी ? यदि बिना ऊष्मा क्षय के पिस्टन को पूर्व स्थिति में ले जाया जाए, तो गैस का साम्यावस्था में ताप कितना होगा ? अपना उत्तर भिन्नात्मक (Fraction) रूप में दे सकते है |

View Solutionएक-परमाणुक आदर्श गैस प्रारम्भिक ताप T3 पर, एक पिस्टन युक्त सिलिण्डर में भरी है। पिस्टन को अचानक स्वतंत्र करके गैस को रुद्धोष्म रूप से, ताप तक प्रसारित होने देते हैं। यदि सिलिण्डर में गैस के प्रसार के पहले एवं बाद में गैस स्तम्भों की लम्बाईयाँ क्रमश: L1 तथा L2 हैं, तब T1/T2 का मान है
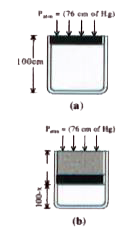
View SolutionA vertical cylinder of height 100cm contains air at a constant temperature and its top is closed by a frictionless piston at atmospheric pressure (76 cm of Hg) as shown figure - a. If mercury is slowly poured on the piston, due to its weight air is compressed. Find the maximum height of the mercury column which can be put on the piston.
View SolutionS is a piston of mass M which can slide inside a cylinder without friction. The walls of the cylinder is adiabatic and the piston is diathermic and area of cross section of cylinder is A. Length of the cylinder is 2l_0 . Initial pressure of each chamber is P_0 . Volume of the chambers are equal. Initially spring is unstretched and has spring constant k. The spring is fixed with piston and wall of the cylinder as shown in the figure. Find out its time period when piston is given small displacement.
View Solution
