Solar radiation is found to have an intensity maximum near the wavelength range of 470nm. Assuming the surface of sun to be perfectly absorbing (a=1), calculate the temperature of solar surface.
Since a=1, sun can be assumed to be emitting as a black body from Wien's law for a black body
λmT=b
⇒T=bλm=0.282(cm−K)(470×10−7cm)
≈6000K.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - RESONANCECHAPTER - HEAT TRANSFEREXERCISE - Board Level Exercise
14 Videos
- HEAT TRANSFERBOOK - RESONANCECHAPTER - HEAT TRANSFEREXERCISE - Exercise-1
1 Video
- HEAT TRANSFERBOOK - RESONANCECHAPTER - HEAT TRANSFEREXERCISE - Advancel Level Problems
17 Videos
- GRAVITATIONBOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - Exercise
21 Videos
- KINEMATICSBOOK - RESONANCECHAPTER - KINEMATICSEXERCISE - Exercise
65 Videos
Similar Questions
The light from th esun is found to have a maximum intensity near the wavelength of 470nm. Assuming that the surface o f the sun emits as a blackbody, calculate the temperture of the surface of the sun.
View SolutionThe sun radiates maximum energy at a wavelength of 470 nm. Assuming the sun's radiation to be similar to that of an abso-lute black body, find the temperature of the photosphere.
View Solution
Knowledge Check
The radiation force experienced by body exposed to radiation of intensity I, assuming surface of body to be perfectly absorbing is:
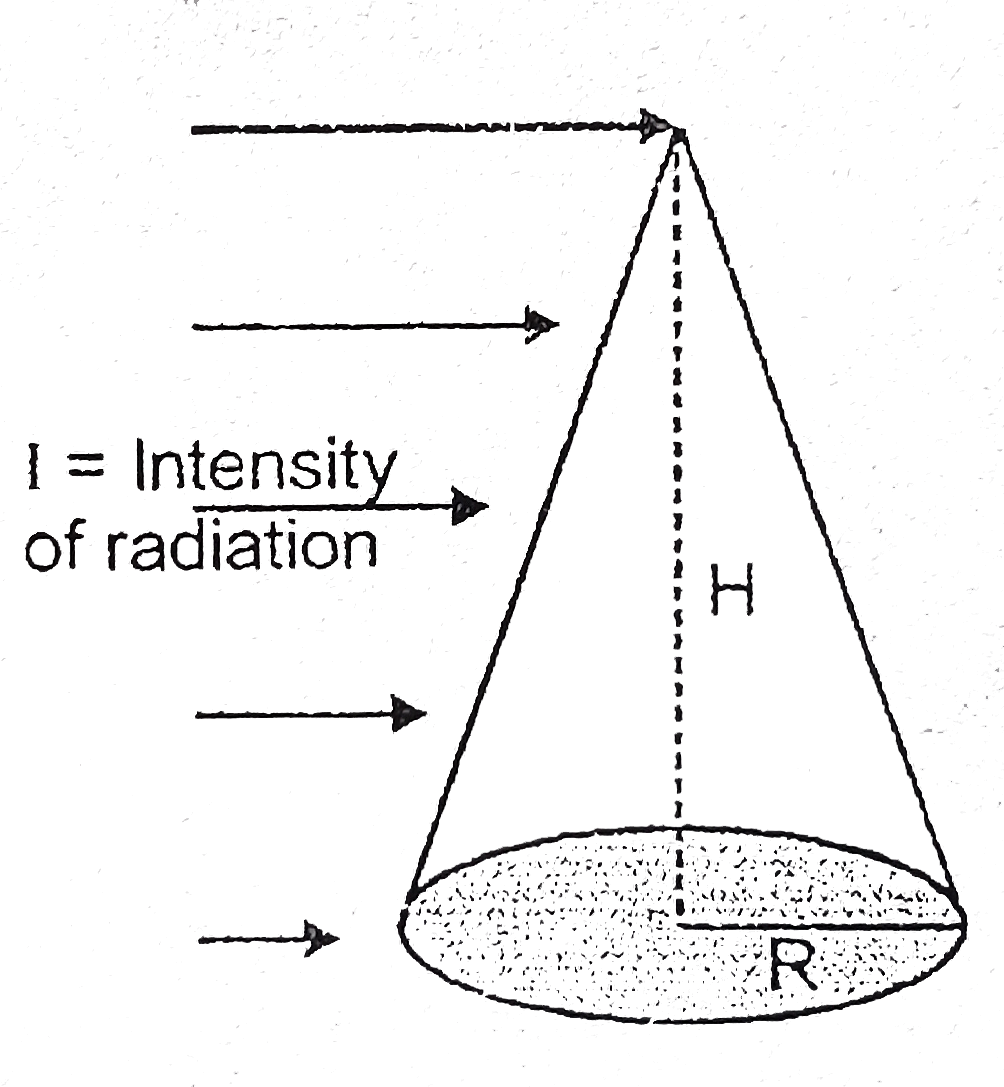 AπR2IcBπRHIcCIRH2cDIRHc
AπR2IcBπRHIcCIRH2cDIRHcThe radiation force experienced by body exposed to radiation of intensity I, assuming surface of body to be perfectly absorbing is:
AπR2IcBπRHIcCIRH2cDIRHcThe sun radiates maximum energy at wavelength 4753Å. The surface temperature of the sun if b=2.888×10−3mK, is
A6076 KB5706 KC4560 KD7000K
Solar radiation with an intensity of 1.36KW/m2 per second falls on the illuminated surface of the Earth. As- suming the sun' s radiates maximum energy at wavelength of 470 nm. Assuming the sun's radiation to be similar to that of an absolute black body. find the temperature of the photosphere.
View SolutionThe radiation emitted by the surface of the Sun emits maximum power at a wavelength of about 500 nm. Assuming the Sun to be a blackbody emitter. If its surface temperature (K) is given by α.Bη×10γ then fill the value of (α+β+γ). (Wien's constant is given by 2.898mm K)
View SolutionThe sun radiates maximum energy at wavelength 4753Å. Estimate the surface temperature of the sun, if b=2.888×10−3mK.
View SolutionIn solar radiation, the intensity of radiation is maximum around the wavelength λm. If R is the radius of the sun and c is the velocity of light, the mass lost by the sum per unit time is proportional to
View SolutionSun and moon emit maximum radiant energy at wavelength 5000 Å and 15μ respectively. Iff surface temperature of sun is 6000 K, then value of surface temperature of moon is
View Solution
