Which of the following can be the valid assignment of probability for outcomes of sample space, $S = {W_{1}, W_{2}, W_{3}}, {where W_{1}, W_{2} and W_{3}$ are mutually exclusive events
Assignment
$\begin{matrix} w_{1} & w_{2} & w_{3} (a) & 0.4 & 0.2 & 0.8 (b) & 0 & 0 & 1 (c) & - \frac{1}{2} & \frac{3}{4} & \frac{1}{2} (d) & \frac{1}{2} & \frac{1}{2} & \frac{1}{4} \end{matrix}$

Video Solution

Text Solution

Verified by Experts

(a) We have, $P (W_{1}) = 0.4, P (W_{2}) = 0.2 and P (W_{3}) = 0.8$
$∴ 0 \leq P (W_{i}) \leq 1, for each W_{i} \in S$
But, $P (W_{1}) + P (W_{2}) + P (W_{3}) = 0.4 + 0.2 + 0.8 = 1.4 > 1$
Hence, this assignment is not valid.
(b) $P (W_{1}) = 0, P (W_{2}) = 0, P (W_{3}) = 1$
Here, $0 \leq P (W (i)) \leq 1, where W_{i} \in S and P (W_{1}) + P (W_{2}) + P (W_{3}) = 1$
Hence this assignment is valid.
(c) Here, $P (W_{1}) < 0$
So, axiom 1 is not satisfied
Hence, this assignment is not valid.
(d) Again, $P (W_{1}) + P (W_{2}) + P (W_{3}) = \frac{1}{2} + \frac{1}{2} + \frac{1}{4} = \frac{5}{4} > 0$
Hence, this assignment is not valid.

Updated on:21/07/2023

If $W_{1} : W_{2} = 2 : 3$ and $W_{1} : W_{3} = 1 : 2$ then $W_{2} : W_{3}$ is
A $3 : 4$
B $4 : 3$
C $2 : 3$
D $4 : 5$
If $W_{1} : W_{2} = 2 : 3$ and $W_{1} : W_{3} = 1 : 2$ , then $W_{2} : W_{3}$ is:
A $3 : 4$
B $4 : 3$
C $2 : 3$
D $4 : 5$
In the circuit shown below $E_{1} = 4.0 V, R_{1} = 2 Ω, E_{2} = 6.0 V, R_{2} = 4 Ω$ and $R_{3} = 2 Ω$ . The current $I_{1}$ is

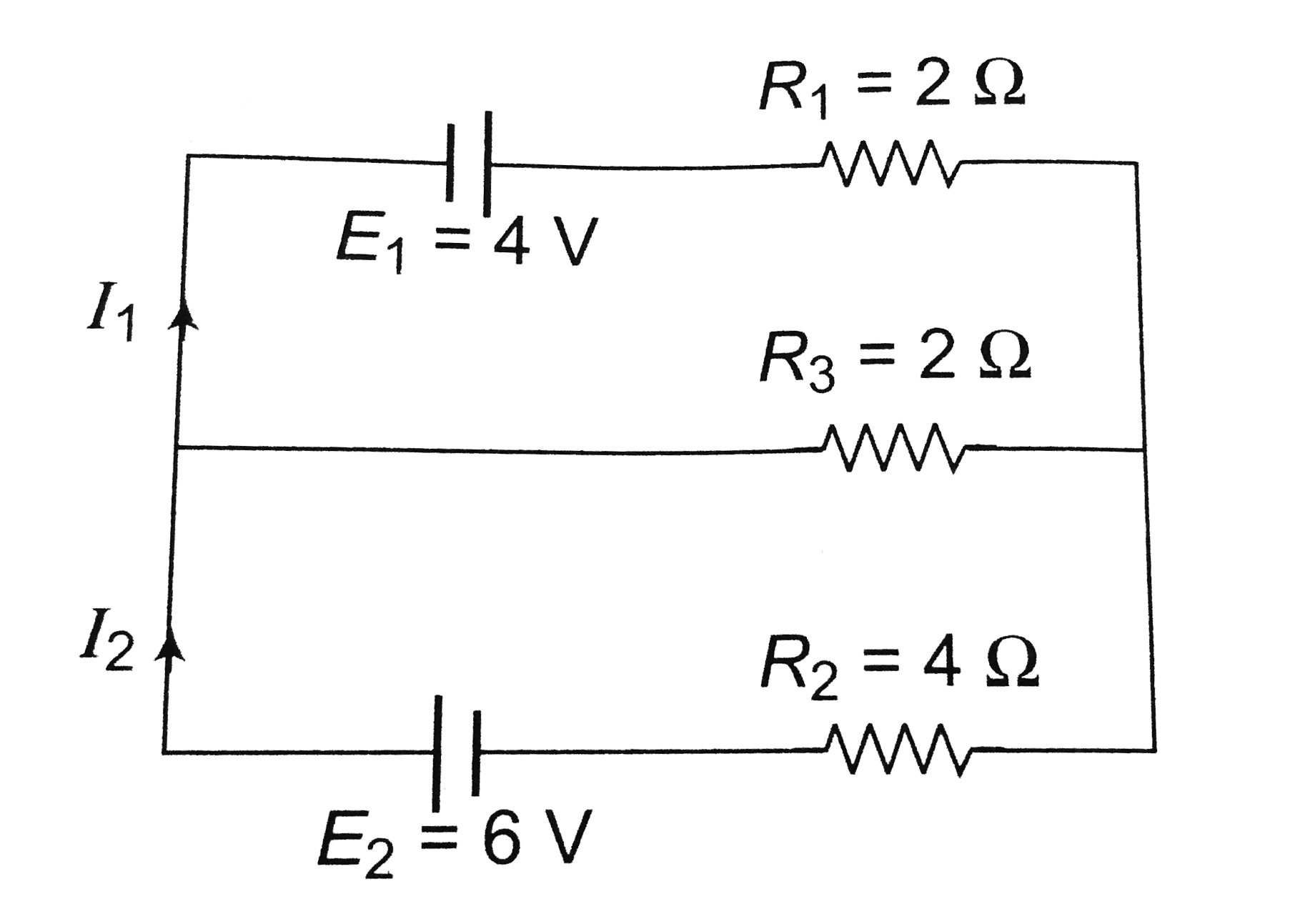A $1.6 A$
B $1.8 A$
C $1.25 A$
D $1.0 A$