A point is chosen at random inside a circle. Find the probability that the point is closer to the centre of the circle than to its circumference.
To find the probability that a point chosen at random inside a circle is closer to the center than to its circumference, we can follow these steps:
Step 1: Understand the Problem
We need to determine the area within a circle where points are closer to the center than to the circumference.
Step 2: Define the Circle
Let the radius of the larger circle be r.
Step 3: Determine the Condition for Closeness
A point inside the circle is closer to the center than to the circumference if it lies within a smaller circle whose radius is half of the larger circle's radius. Therefore, we define a smaller circle with radius r2.
Step 4: Calculate the Area of the Larger Circle
The area A of the larger circle is given by the formula:
A=πr2
Step 5: Calculate the Area of the Smaller Circle
The area A′ of the smaller circle with radius r2 is given by:
A′=π(r2)2=πr24
Step 6: Determine the Probability
The probability P that a randomly chosen point inside the larger circle is also inside the smaller circle (and hence closer to the center) is given by the ratio of the area of the smaller circle to the area of the larger circle:
P=A′A=πr24πr2
Step 7: Simplify the Probability Expression
Cancelling out πr2 from the numerator and denominator, we get:
P=14
Final Answer
Thus, the probability that a point chosen at random inside the circle is closer to the center than to its circumference is:
14
---
We need to determine the area within a circle where points are closer to the center than to the circumference.
Let the radius of the larger circle be r.
A point inside the circle is closer to the center than to the circumference if it lies within a smaller circle whose radius is half of the larger circle's radius. Therefore, we define a smaller circle with radius r2.
The area A of the larger circle is given by the formula:
A=πr2
The area A′ of the smaller circle with radius r2 is given by:
A′=π(r2)2=πr24
The probability P that a randomly chosen point inside the larger circle is also inside the smaller circle (and hence closer to the center) is given by the ratio of the area of the smaller circle to the area of the larger circle:
P=A′A=πr24πr2
Cancelling out πr2 from the numerator and denominator, we get:
P=14
Thus, the probability that a point chosen at random inside the circle is closer to the center than to its circumference is:
14
Topper's Solved these Questions
- PROBABILITYBOOK - AAKASH INSTITUTECHAPTER - PROBABILITYEXERCISE - TRY YOURSELF
36 Videos
- PROBABILITYBOOK - AAKASH INSTITUTECHAPTER - PROBABILITYEXERCISE - ASSIGNMENT (SECTION - A Competition Level Questions)
114 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTECHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
12 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
Similar Questions
There are two circles in the x-y plane whose equations are x2+y2−2y=0 and x2+y2−2y−3=0. A point is chosen at random inside the larger circle. Find the probability that the point has been taken from outside the smaller circle.
View SolutionA point is selected at random from the interior of a circle. The probability that the point is closer to the centre than the boundary of the circle is
View Solution
Knowledge Check
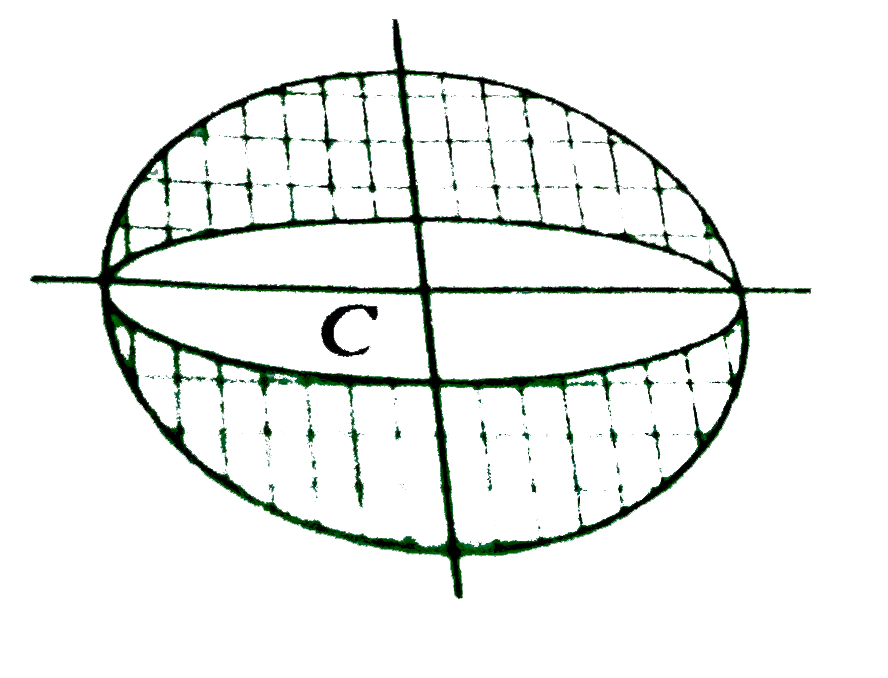
There are some experiment in which the outcomes cannot be identified discretely. For example, an ellipse of eccentricity 2√2/3 is inscribed in a circle and a point within the circle is chosen at random. Now, we want to find the probability that this point lies outside the ellipse. Then, the point must lie in the shaded region shown in Figure. Let the radius of the circle be a and length of minor axis of the ellipse be 2b. Given that
1−b2a2=89orb2a2=19
Then, the area of circle serves as sample space and area of the shaded region represents the area for favorable cases. Then, required probability is
p=Area of shaded regionArea of circle
=πa2−πabπa2=1−ba=1−13=23
Now, answer the following questions.
A point is selected at random inside a circle. The probability that the point is closer to the center of the circle than to its circumference isA1/4B1/2C1/3D1/√2A point is chosen at random inside a circle. What is the probability that the point is closer to the centre of the circle than to its boundary?
A1√2B14C13D12A point is selected at random from inside a circle. The probability that the point is closer to the circumference of the circle than to its centre, is
A23B13C34D14
If a triangle is chosen at random inscribed in a fixed circle and if the probability tha the orthocentre lies inside circumcircle is 1x then x equals __________
View SolutionThere is a point inside a circle. What is the probability that this point is close to the circumference than to the center?
View SolutionThere is a point inside a circle. What is the probability that this point is close to the circumference than to the center?
View SolutionThere is a point inside a circle. What is the probability that this point is close to the circumference than to the centre ?
View SolutionA point is selected randomly from the interior of a circle. The probability that the point is closer to the centre, then the boundary of circle is
View Solution
