A charge Q is filled in a spherical volume of Radius R . Use the concept of energy density , find energy stored inside the charge volume.
For
x<R,E(x)=14πε0QR3x
∴UIN∫dU=∫x=Rx=012ε0E2(x)×4πx2dx=15×Q28πε0R

Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - TRY YOURSELF110videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT (SECTION-A) Objective Type Questions (Only one answer)47videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions25videos
ELECTROMAGNETIC WAVES
- BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)16videos
GRAVITATION
Similar Questions
Knowledge Check
- Question 1 - Select One
A solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
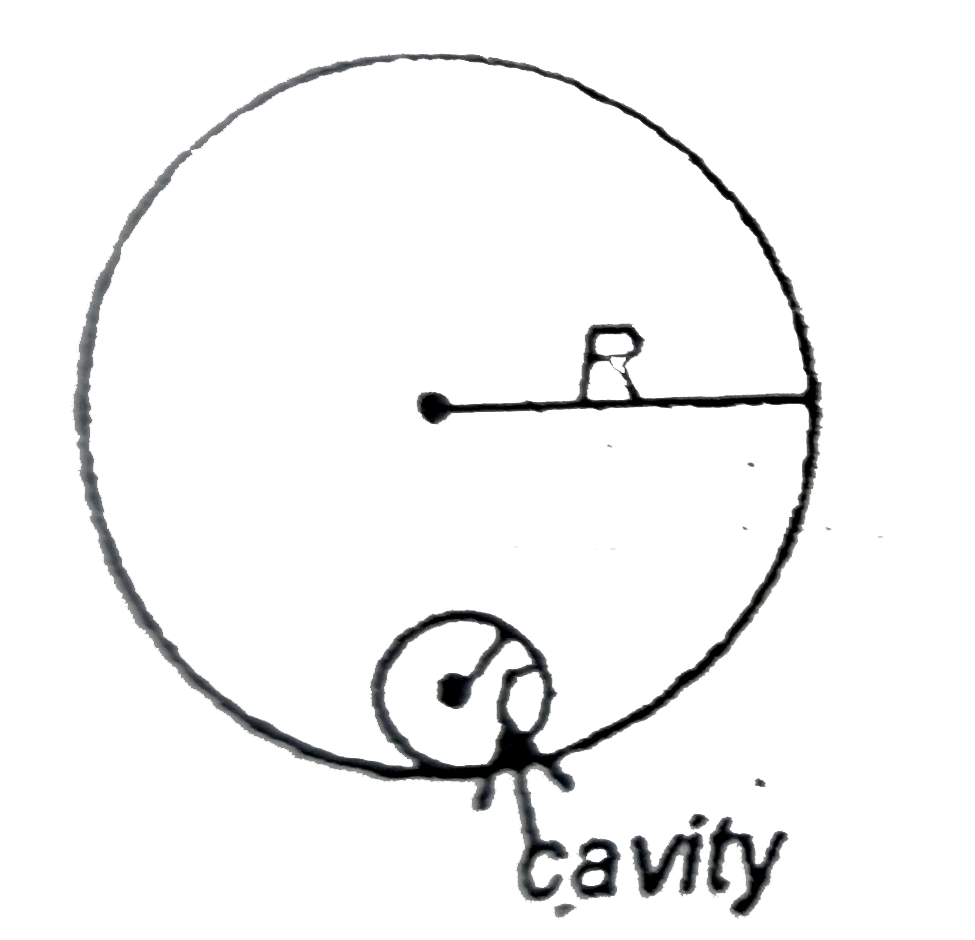 AR3σ2πε0(R−r)2BRσ3πε0C13ε0r3σ(R−r)2Drσ3ε0(R−r)2
AR3σ2πε0(R−r)2BRσ3πε0C13ε0r3σ(R−r)2Drσ3ε0(R−r)2 - Question 2 - Select One
In a charged capacitor, the energy is stored in
Athe negative chargesBthe positive chargesCthe field between the platesDboth 'a' and 'b'
A charge q is distributed over the volume of a sphere of radius R. Assuming the permittivty to be equal to unity, compare the energy stored inside the sphere with the energy pervading the surrounding space.
[Hint: u=12ε0E2.]View SolutionA metal sphere of radius R is charged to a pontital V . (a ) Find the electtrostatic energy stored in the electric field within a concentric sphere of radius 2 R . (b )Show that the electrostatic field energy stored outside the sphere of radius 2R equals that stored within it .
View SolutionUsing the concept of energy density, find the total energy stored in a
a. parallel plate capacitor
b. charged spherical conductor.View SolutionA point charge +Q is placed at the centre of an isolated conducting shell of radius R. Find the electrostatic potential energy stored outside the spherical shell if the shell also contains charge +Q distributed uniformly over it.
 View Solution
View Solution(i). A charge of Q coulomb is uniformly distributed over a spherical volume of radius R metre Obtain an expression for the energy of the system.
(ii). What will be the corresponding expression for the energy needed to completely diassemble the planet earth against the gravitational pull amongst its constituent particles? Assume the earth to be a sphere of uniform mass density. calculate the energy, given that the product of the mass and the radius of the earth to be 2.5×1031kg−mView SolutionCalculate the self potential energy of charge q distributed over the surface of a hollow sphere of radius R. What happens to this self energy when R=0, or when the charge is an ideal point charge?
View Solution

