If all the energy lost from joule heating stays in a wire , and the temperature increases as a result the resistivity also increase. The current will therefore change as a function of time of time, the joule heating will change and so forth. If the wire material has constant heat capacity , the rate of energy loss in wire will be proportional to rate of change of temperature . Assuming that potential stays constant set up a differential equation that describes the rate of change of temperature .If this equation is solved, how can the current he found as a function of time ?
(Given m is the mass of the wire , V is the potential difference across the wire, C is the specfic heat capacity of wire , α is the thermal coefficient of resistance, R0 is the resistance of wire at initial temperature T0).
dTdt=K1+α(T−T0)
Here K=V2mcR0
l(t)=VR0(1+α(T(t)−T0))
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTECHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT SECTION-I(SUBJECTIVE TYPE QUESTIONS)
13 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTECHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTECHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
Knowledge Check
A: When temperature of a metallic wire is increased, its resistance increase .
R : As the temperature is increaed , average relaxtion time increase.AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion , then markBIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion then mark.CIf Assertion is ture statement but Reason is false , then markDIf both Assertion and Reason are false statements then mark.A steady current I flows through a wire of radius r, length L and resistivity ρ. The current produced heat in the wire. The rate of heat loss in a wire is proportional to its surface area. The steady temperature of the wire is independent of–
ALBrCIDρIf the amount of heat given to a system be 35 joules and the amount of work done on the system be 15 joules , then the change in the internal energy of the system is
A−50JB20JC30JD50J
A: When temperature of a metallic wire is increased, its resistance increase .
R : As the temperature is increaed , average relaxtion time increase.
View SolutionA copper wire is stretched so as to increase its length by 0.2%. Calculate the percentage change in the resistance of the wire.
View SolutionA solid material is supplied heat at a constant rate. The temperature of material is changing with heat input as shown in the figure. What does the slope of DE represent ?
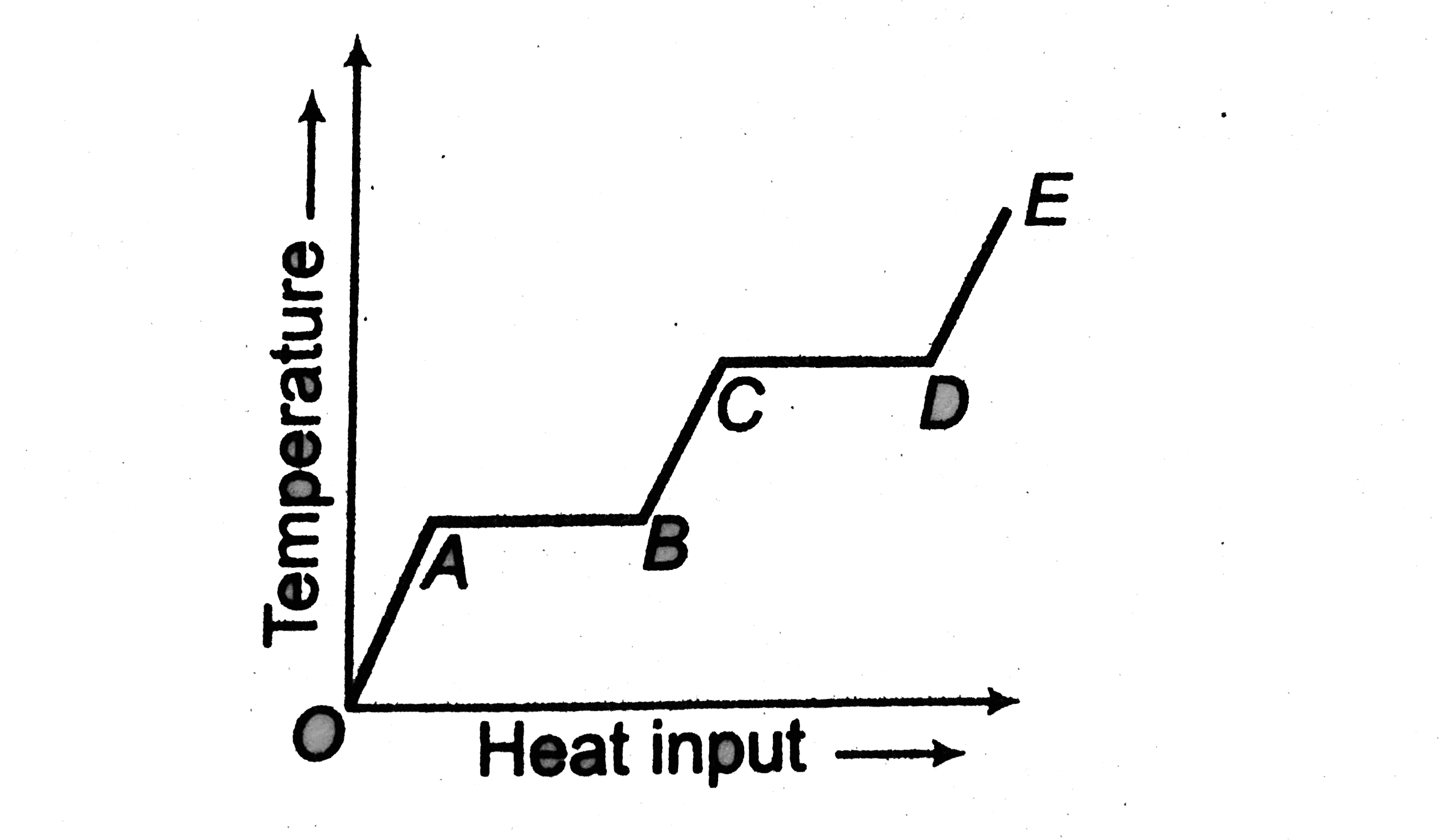
View SolutionIf heat is added at constant volume, 6300 J of heat are required to raise the temperature of an ideal gas by 150 K. If instead, heat is added at constant pressure, 8800 joules are required for the same temperature change. When the temperature of the gas changes by 300K, the internal energy of the gas changes by
View SolutionA solid material is supplied heat at a constant rate. The temperature of material is changing with heat input as shown in the figure. What does the slope of DE represent ?

View Solution
