A thin rectangular plate with sides of length 'a' and 'b' has mass per unit area varying as $α = α_{0} (\frac{x y}{a b})$ where $α_{0}$ is a constant. The co-ordinated of centre of mass of the plate is

A

$(\frac{a}{3}, \frac{b}{3})$

B

$(\frac{a}{2}, \frac{b}{2})$

C

$(\frac{4 a}{3}, \frac{4 b}{3})$

D

$(\frac{2 a}{3}, \frac{2 b}{3})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$d m = (\frac{α_{0} x}{a b}) y (d y) (d x)$

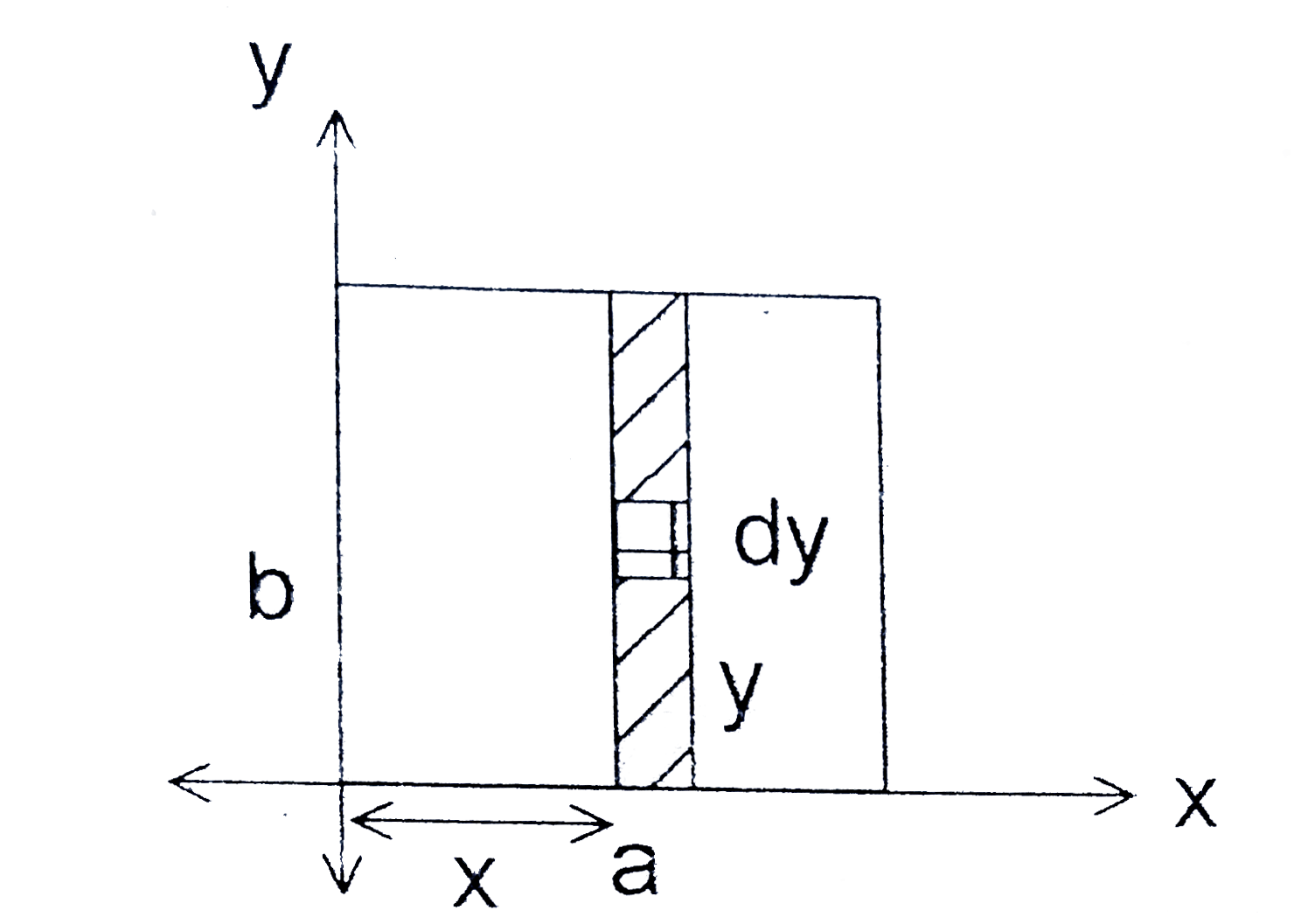
$\to R = (x, y)$
$\frac{\int \to R . d m}{\int d m} = \frac{\int \frac{α_{0} x y}{a b} (d x d y) (x ˆ i + y ˆ j)}{\int \frac{α_{0} x y}{a b} d x . d y}$ $\frac{\int \frac{α_{0} x y}{a b} \times d x . d y ˆ i}{\int \frac{α_{0} x y}{a b} d x . y d} + \frac{\int \frac{α_{0} x y}{a b} d x . d y (y) ˆ j}{\int \frac{α_{0} x y}{a b} d x . d y}$
$y = C O M x =$
$d y = d x =$
$\frac{a \int 0 x^{2} d x}{a \int 0 x d x} ˆ i + \frac{b \int 0 y^{2} d y}{b \int 0 y d x}$
$\frac{2 a}{3} ˆ i + \frac{2 b}{3} ˆ j$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A thin bar of length L has a mass per unit length $λ$ , that increases linerarly with distance from one end. If its total mass is M and its mass per unit length at the lighter end is $λ_{0}$ , then the distance of the centre of mass from the lighter end is
A $\frac{L}{3} + \frac{λ_{0} L^{2}}{8 M}$
B $\frac{L}{3} + \frac{λ_{0} L^{2}}{4 M}$
C $\frac{L}{2} - \frac{λ_{0} L^{2}}{4 M}$
D $\frac{2 L}{3} - \frac{λ_{0} L^{2}}{6 M}$
Question 2 - Select One
If $z (¯ ¯¯¯¯¯¯¯ ¯ z + α) + ¯ z (z + α) = 0$ , where $α$ is a complex constant, then z is represented by a point on
Aa circle
Ba straight line
Ca parabola
Dnone of these

FIITJEE-TEST PAPERS-PHYSICS

Home
Ask Doubt
Profile